-
As a specific group of observables in relativistic heavy ion collisions [1–12], light nuclei such as the deuteron (d), triton (t), helium-3 (3He), and helium-4 (4He) have been under active investigation in recent decades both in experiment [13–23] and theory [24–29]. The STAR experiment at the BNL Relativistic Heavy Ion Collider (RHIC) and the ALICE experiment at the CERN Large Hadron Collider (LHC) have collected a wealth of data on light nucleus production. These data exhibit some fascinating features, especially their non-trivial energy-dependent behaviors in a wide collision energy range from the GeV to TeV scale [17–23]. Theoretical studies have also made significant progress. Two production mechanisms, the thermal production [29–33] and coalescence mechanisms [26, 27, 34–42], have proved to be successful in describing light nucleus formation. In addition, the transport scenario [43–48] is employed to study the evolution and survival of light nuclei during the evolution of the hadronic system.
The coalescence mechanism, in which light nuclei are usually assumed to be produced by the coalescence of jacent nucleons in the phase space, possesses unique characteristics. Many current experimental observations at high RHIC and LHC energies favor nucleon coalescence [18, 19, 22, 23, 49–51]. Recently, the STAR collaboration extended the beam energy scan program to lower collision energies and published the data of both hadrons and light nuclei in Au-Au collisions at
$ \sqrt{s_{NN}}=3 $ GeV [52–55]. These data show very different properties from those at high RHIC and LHC energies, such as the disappearance of partonic collectivity [52] and dominant baryonic interactions [53]. At this low collision energy, besides nucleons, light nuclei, particularly light d, t, and 3He, have been more abundantly created [55] than in higher collision energies [56]. In physics, it is easier for these light nuclei to capture nucleons or other light nuclei to form heavier composite objects. In fact, clear depletions below unity of the proton$ -d $ and$ d-d $ correlation functions measured at such low collision energies indicate the strong final state interaction and further support the possible coalescence of d with the nucleon or other d [57]. How much space is there on earth for particle coalescence other than for nucleons, e.g., composite particles of lower mass numbers coalescing into light nuclei of larger mass numbers or composite particles capturing nucleons to recombine into heavier light nuclei?In this study, we extend the coalescence model, which has been successfully used to explain the momentum dependence of yields and the coalescence factors of different light nuclei at high RHIC and LHC energies [51, 58], to include nucleon+nucleus and nucleus+nucleus coalescence as well as nucleon coalescence. We apply the extended coalescence model to hadronic systems created in Au-Au collisions in the midrapidity area at
$ \sqrt{s_{NN}}=3 $ GeV to study the momentum and centrality dependence of light nucleus production in the low- and intermediate-$ p_T $ regions. We compute the transverse momentum ($ p_T $ ) spectra, yield rapidity densities (${\rm d}N/{\rm d}y$ ), yield ratios, and averaged transverse momenta ($ \langle p_T \rangle $ ) of d, t, 3He, and 4He from central to peripheral collisions and provide the proportions of contributions from different coalescence sources for t, 3He, and 4He in their production. Our study shows that in the 0−10%, 10%−20%, and 20%−40% centralities, besides nucleon coalescence, nucleon$ +d $ coalescence plays an important role in t and 3He production, and nucleon$ +d $ (t, 3He) and$ d+d $ coalescence occupy significant proportions in 4He production. However, in the peripheral 40%−80% centrality, nucleon coalescence plays a dominant role, and nucleon+nucleus coalescence or nucleus+nucleus coalescence seems to disappear.The rest of the paper is organized as follows. In Sec. II, we introduce the coalescence model and present analytic formulas of the momentum distributions of two bodies, three bodies, and four nucleons coalescing into light nuclei. In Sec. III, we apply the model to Au-Au collisions at different rapidity intervals in the midrapidity area at
$ \sqrt{s_{NN}}=3 $ GeV to study the momentum and centrality dependence of the production of various species of light nuclei in the low- and intermediate-$ p_T $ regions. We also provide the proportions of contributions from different coalescence sources for t, 3He, and 4He in their production. In Sec. IV, we summarize our study. -
In this section, we introduce the coalescence model, which is used to deal with light nucleus production. The starting point of the model is a hadronic system produced at the late stage of the evolution of high energy collisions. The hadronic system consists of different species of primordial mesons and baryons. In the first step of the model, all primordial nucleons are allowed to form d, t, 3He, and 4He via nucleon coalescence. Then, in the second step, the formed d, t, and 3He capture the remanent primordial nucleons, i.e., excluding those consumed in the nucleon coalescence process, or other light nuclei to recombine into nuclei with larger mass numbers. We consider not only nucleon interactions resulting in light nucleus production, but also the interactions between the nucleus and the remanent nucleons. In this model, only d, t, 3He, and 4He are included, and light nuclei with mass numbers larger than 4 are abandoned.
In the following, we construct the formalism for the production of various species of light nuclei via different coalescence processes. First, we present the analytic results of two bodies coalescing into light nuclei, which can be applied to processes such as
$ p+n \rightarrow d $ ,$ n+d \rightarrow t $ ,$ p+d \rightarrow {}^{3} {\rm{He}}$ ,$ p+t \rightarrow {}^{4} {\rm{He}}$ ,$ n+{}^{3} {\rm{He}}$ $ \rightarrow {}^{4} {\rm{He}}$ , and$d+d \rightarrow {}^{4} {\rm{He}}$ . Then, we show the analytic results of three bodies coalescing into light nuclei, which can be used to describe these processes, e.g.,$ n+n+p \rightarrow t $ ,$ p+p+n \rightarrow {}^{3} {\rm{He}}$ , and$ p+n+d \rightarrow {}^{4} {\rm{He}}$ . Finally, we provide the analytic result of four nucleons coalescing into 4He, i.e.,$p+p+n+ n \rightarrow {}^{4} {\rm{He}}$ . -
We begin with a hadronic system produced at the final stage of the evolution of high energy collisions and suppose light nuclei
$ L_{j} $ are formed via the coalescence of two hadronic bodies$ h_1 $ and$ h_2 $ . The three-dimensional momentum distribution of the produced light nuclei$ f_{L_{j}}({\boldsymbol{p}}) $ is given by$ \begin{aligned}[b] f_{L_{j}}({\boldsymbol{p}}) = & \int {\rm d}{\boldsymbol{x}}_1{\rm d}{\boldsymbol{x}}_2 {\rm d}{\boldsymbol{p}}_1 {\rm d}{\boldsymbol{p}}_2 f_{h_1h_2}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2) \\ & \times {\cal{R}}_{L_{j}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}), \end{aligned} $

(1) where
$ f_{h_1h_2}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2) $ is the two-hadron joint coordinate-momentum distribution, and$ {\cal{R}}_{L_{j}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}) $ is the kernel function. Hereafter, we use bold symbols to denote three-dimensional coordinate or momentum vectors.In terms of the normalized joint coordinate-momentum distribution denoted by the superscript '
$ (n) $ ', we have$ \begin{aligned}[b] f_{L_{j}}({\boldsymbol{p}})=& N_{h_1h_2} \int {\rm d}{\boldsymbol{x}}_1{\rm d}{\boldsymbol{x}}_2 {\rm d}{\boldsymbol{p}}_1 {\rm d}{\boldsymbol{p}}_2 f^{(n)}_{h_1h_2}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2) \\ & \times {\cal{R}}_{L_{j}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}). \end{aligned} $

(2) $ N_{h_1h_2} $ is the number of all possible$ h_1h_2 $ -pairs and is equal to$ N_{h_1}N_{h_2} $ and$ N_{h_1}(N_{h_1}-1) $ for$ h_1 \neq h_2 $ and$ h_1=h_2 $ , respectively.$ N_{h_i}\; (i=1, 2) $ is the number of hadrons$ h_i $ in the considered hadronic system.The kernel function
$ {\cal{R}}_{L_{j}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}) $ denotes the probability density for$ h_1 $ ,$ h_2 $ with momenta$ {\boldsymbol{p}}_1 $ and$ {\boldsymbol{p}}_2 $ at$ {\boldsymbol{x}}_1 $ and$ {\boldsymbol{x}}_2 $ , respectively, to recombine into$ L_{j} $ of momentum$ {\boldsymbol{p}} $ . It carries the kinetic and dynamical information of$ h_1 $ and$ h_2 $ recombining into light nuclei, and its precise expression should be constrained by, for example, the momentum conservation and constraints due to intrinsic quantum numbers (e.g., spin) [51, 58, 59]. To take these constraints into account explicitly, we rewrite the kernel function in the following form:$ \begin{aligned}[b] {\cal{R}}_{L_{j}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}) =& g_{L_{j}} {\cal{R}}_{L_{j}}^{(x, p)}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2) \\ & \times \delta\left( {\sum^2_{i=1}} {\boldsymbol{p}}_i-{\boldsymbol{p}}\right), \end{aligned} $

(3) where the spin degeneracy factor
$ g_{L_{j}} = (2J_{L_{j}}+1) / \Big[\prod \limits_{i=1}^2(2J_{h_i}+1)\Big] $ .$ J_{L_{j}} $ is the spin of the produced$ L_{j} $ and$ J_{h_i} $ for the primordial hadron$ h_i $ . The Dirac δ function guarantees the momentum conservation in the coalescence. The remaining$ {\cal{R}}_{L_{j}}^{(x, p)}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2) $ can be solved from the Wigner transformation once the wave function of$ L_{j} $ is given with the instantaneous coalescence approximation,$ {\cal{R}}^{(x, p)}_{L_{j}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2) = 8e^{-\tfrac{({\boldsymbol{x}}'_1-{\boldsymbol{x}}'_2)^2}{2\sigma^2}} {\rm e}^{-\tfrac{2\sigma^2(m_2{\boldsymbol{p}}'_{1}-m_1{\boldsymbol{p}}'_{2})^2}{(m_1+m_2)^2\hbar^2c^2}}, $

(4) because we adopt the wave function of a spherical harmonic oscillator, as in Refs. [60, 61]. The superscript '
$ ' $ ' in the coordinate or momentum variable denotes the hadronic coordinate or momentum in the rest frame of the$ h_1h_2 $ -pair.$ m_1 $ and$ m_2 $ are the rest masses of hadron$ h_1 $ and hadron$ h_2 $ , respectively. The width parameter$\sigma= \sqrt{\dfrac{2(m_1+m_2)^2}{3(m_1^2+m_2^2)}} R_{L_{j}}$ , where$ R_{L_{j}} $ is the root-mean-square radius of$ L_{j} $ , and its values for different light nuclei can be found in Ref. [62]. The factor$ \hbar c $ arises from the used GeV·fm unit and is 0.197 GeV·fm.The normalized two-hadron joint distribution
$ f^{(n)}_{h_1h_2}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2) $ is generally coordinate and momentum coupled, especially in central heavy-ion collisions with relatively high collision energies where long collective expansion exists. The coupling intensities and their specific forms are probably different in different phase spaces at different collision energies and different collision centralities. This coupling effect on the production properties of light nuclei in Pb-Pb collisions at the LHC was investigated in our recent study [63]. In Ref. [58], the coalescence model ignoring this coordinate and momentum coupling was proved to be successful in explaining the experimental data of d,$ \bar d $ , and t in Au-Au collisions at RHIC$ \sqrt{s_{NN}}=7.7-54.4 $ GeV. In this study, we attempt to derive production formulas analytically and present the centrality and momentum dependence of light nuclei more intuitively in Au-Au collisions at the lower RHIC energy$ \sqrt{s_{NN}}=3 $ GeV, where partonic collectivity disappears [52]. Therefore, we consider a simple case in which the joint distribution is coordinate and momentum factorized, i.e.,$ \begin{align} f^{(n)}_{h_1h_2}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2) = f^{(n)}_{h_1h_2}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2) f^{(n)}_{h_1h_2}({\boldsymbol{p}}_1, {\boldsymbol{p}}_2). \end{align} $

(5) Substituting Eqs. (3)−(5) into Eq. (2), we have
$ \begin{aligned}[b] f_{L_{j}}({\boldsymbol{p}})= & N_{h_1h_2} g_{L_{j}} \int {\rm d}{\boldsymbol{x}}_1{\rm d}{\boldsymbol{x}}_2 f^{(n)}_{h_1h_2}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2) 8{\rm e}^{-\tfrac{({\boldsymbol{x}}'_1-{\boldsymbol{x}}'_2)^2}{2\sigma^2}} \\ & \times \int {\rm d}{\boldsymbol{p}}_1{\rm d}{\boldsymbol{p}}_2 f^{(n)}_{h_1h_2}({\boldsymbol{p}}_1, {\boldsymbol{p}}_2) {\rm e}^{-\tfrac{2\sigma^2(m_2{\boldsymbol{p}}'_{1}-m_1{\boldsymbol{p}}'_{2})^2}{(m_1+m_2)^2\hbar^2c^2}} \delta \left( {\sum^2_{i=1}} {\boldsymbol{p}}_i-{\boldsymbol{p}}\right) \\ = & N_{h_1h_2} g_{L_{j}} {\cal{A}}_{L_{j}} {\cal{M}}_{L_{j}}({\boldsymbol{p}}),\\[-20pt] \end{aligned} $

(6) where we use
$ {\cal{A}}_{L_{j}} $ to denote the coordinate integral part as$ {\cal{A}}_{L_{j}} = 8\int {\rm d}{\boldsymbol{x}}_1{\rm d}{\boldsymbol{x}}_2 f^{(n)}_{h_1h_2}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2) {\rm e}^{-\tfrac{({\boldsymbol{x}}'_1-{\boldsymbol{x}}'_2)^2}{2\sigma^2}}, $

(7) and use
$ {\cal{M}}_{L_{j}}({\boldsymbol{p}}) $ to denote the momentum integral part as$ {\cal{M}}_{L_{j}}({\boldsymbol{p}}) = \int {\rm d}{\boldsymbol{p}}_1{\rm d}{\boldsymbol{p}}_2 f^{(n)}_{h_1h_2}({\boldsymbol{p}}_1, {\boldsymbol{p}}_2) {\rm e}^{-\tfrac{2\sigma^2(m_2{\boldsymbol{p}}'_{1}-m_1{\boldsymbol{p}}'_{2})^2}{(m_1+m_2)^2\hbar^2c^2}} \delta \left( {\sum\limits^2_{i=1}} {\boldsymbol{p}}_i-{\boldsymbol{p}}\right). $

(8) $ {\cal{A}}_{L_{j}} $ represents the probability of a$ h_1h_2 $ -pair satisfying the coordinate requirement to recombine into$ L_j $ , and$ {\cal{M}}_{L_{j}}({\boldsymbol{p}}) $ represents the probability density of a$ h_1h_2 $ -pair satisfying the momentum requirement to recombine into$ L_j $ with momentum$ {\boldsymbol{p}} $ .Changing the integral variables in Eq. (7) to be
$ {\boldsymbol{X}}= \dfrac{{\boldsymbol{x}}_1+{\boldsymbol{x}}_2}{\sqrt{2}} $ and$ {\boldsymbol{r}}= \dfrac{ {\boldsymbol{x}}_1-{\boldsymbol{x}}_2}{\sqrt{2}} $ , we have$ {\cal{A}}_{L_{j}} = 8\int {\rm d}{\boldsymbol{X}} {\rm d}{\boldsymbol{r}} f^{(n)}_{h_1h_2}({\boldsymbol{X}}, {\boldsymbol{r}}) {\rm e}^{-\frac{{\boldsymbol{r}}'^2}{\sigma^2}}, $

(9) and the normalizing condition
$ \int f^{(n)}_{h_1h_2}({\boldsymbol{X}}, {\boldsymbol{r}}) {\rm d}{\boldsymbol{X}}{\rm d}{\boldsymbol{r}}=1. $

(10) We further assume that the coordinate joint distribution is coordinate variable factorized, i.e.,
$f^{(n)}_{h_1h_2}({\boldsymbol{X}}, {\boldsymbol{r}}) = f^{(n)}_{h_1h_2}({\boldsymbol{X}}) f^{(n)}_{h_1h_2}({\boldsymbol{r}})$ . Adopting$f^{(n)}_{h_1h_2}({\boldsymbol{r}}) = \dfrac{1}{(\pi C_w R_f^2)^{3/2}} {\rm e}^{-{{\boldsymbol{r}}^2}/{C_w R_f^2}}$ as in Refs. [51, 64], we have$ {\cal{A}}_{L_{j}} = \frac{8}{(\pi C_w R_f^2)^{3/2}} \int {\rm d}{\boldsymbol{r}} {\rm e}^{-\frac{{\boldsymbol{r}}^2}{C_wR_f^2}} {\rm e}^{-\frac{{\boldsymbol{r}}'^2}{\sigma^2}}. $

(11) Here,
$ R_f $ is the effective radius of the hadronic system at the light nucleus freeze-out, and$ C_w $ is a distribution width parameter, which is set as 2, as in Refs. [51, 64].Considering instantaneous coalescence in the rest frame of the
$ h_1 h_2 $ -pair, i.e.,$ \Delta t'=0 $ , we obtain$ {\boldsymbol{r}} = {\boldsymbol{r}}' +(\gamma-1)\frac{{\boldsymbol{r}}'\cdot {\boldsymbol{\beta}}}{\beta^2}{\boldsymbol{\beta}}, $

(12) where
$ {\boldsymbol{\beta}} $ is the three-dimensional velocity vector of the center-of-mass frame of the$ h_1h_2 $ -pair in the laboratory frame, and the Lorentz contraction factor$ \gamma=1/\sqrt{1-{\boldsymbol{\beta}}^2} $ . Substituting Eq. (12) into Eq. (11) and integrating from the relative coordinate variable, we can obtain$ {\cal{A}}_{L_{j}} = \frac{8\sigma^3}{(C_w R_f^2+\sigma^2) \sqrt{C_w (R_f/\gamma)^2+\sigma^2}}. $

(13) The γ factor arises because the analytical coordinate integration is based on a static non-evolving emission source with an effective radius
$ R_f $ in the laboratory frame.Because
$ \hbar c/\sigma $ in Eq. (8) has a small value of approximately 0.1, we can mathematically approximate the Gaussian form of the momentum-dependent kernel function as a δ function as follows:$ {\rm e}^{-\tfrac{({\boldsymbol{p}}'_{1}-\frac{m_1}{m_2}{\boldsymbol{p}}'_{2})^2} {(1+\frac{m_1}{m_2})^2 \frac{\hbar^2c^2}{2\sigma^2}}} \approx \left[ \frac{\hbar c}{\sigma} (1+\frac{m_1}{m_2}) \sqrt{\frac{\pi}{2}} \right]^3 \delta\left({\boldsymbol{p}}'_{1}-\frac{m_1}{m_2}{\boldsymbol{p}}'_{2}\right). $

(14) After integrating
$ {\boldsymbol{p}}_1 $ and$ {\boldsymbol{p}}_2 $ from Eq. (8), we can obtain${\cal{M}}_{L_{j}}({\boldsymbol{p}}) = \left(\frac{\hbar c\sqrt{\pi}}{\sqrt{2}\sigma}\right)^3 \gamma f^{(n)}_{h_1h_2}\left(\frac{m_1{\boldsymbol{p}}}{m_1+m_2}, \frac{m_2{\boldsymbol{p}}}{m_1+m_2}\right), $

(15) where γ originates from
$ {\boldsymbol{p}}'_{1}-\dfrac{m_1}{m_2}{\boldsymbol{p}}'_{2}=\dfrac{1}{\gamma} ({\boldsymbol{p}}_{1}-\dfrac{m_1}{m_2}{\boldsymbol{p}}_{2}) $ .Substituting Eqs. (13) and (15) into Eq. (6) and ignoring correlations between the
$ h_1 $ and$ h_2 $ hadrons, we have$ \begin{aligned}[b] f_{L_{j}}({\boldsymbol{p}}) =& \frac{ (\sqrt{2\pi}\hbar c)^3 g_{L_{j}} \gamma}{(C_w R_f^2+\sigma^2) \sqrt{C_w (R_f/\gamma)^2+\sigma^2}} f_{h_1}\left(\frac{m_1{\boldsymbol{p}}}{m_1+m_2}\right) \\ & \times f_{h_2}\left(\frac{m_2{\boldsymbol{p}}}{m_1+m_2}\right). \end{aligned} $

(16) Denoting the Lorentz invariant momentum distribution
$\dfrac{{\rm d}^{2}N}{2\pi p_{T}{\rm d}p_{T}{\rm d}y}$ with$ f^{({\rm{inv}})} $ , we finally obtain$ \begin{aligned}[b] f_{L_j}^{({\rm{inv}})}(p_{T}, y) =\frac{ (\sqrt{2\pi}\hbar c)^3 g_{L_{j}} }{(C_w R_f^2+\sigma^2) \sqrt{C_w (R_f/\gamma)^2+\sigma^2}} \frac{m_1+m_2}{m_1m_2} \end{aligned} $

$ \begin{aligned}[b]\quad\times f_{h_1}^{({\rm{inv}})}\left(\frac{m_1p_{T}}{m_1+m_2}, y\right) f_{h_2}^{({\rm{inv}})}\left(\frac{m_2p_{T}}{m_1+m_2}, y\right) , \end{aligned} $

(17) where y is the longitudinal rapidity.
-
For light nuclei
$ L_{j} $ formed via the coalescence of the three hadronic bodies$ h_1 $ ,$ h_2 $ , and$ h_3 $ , the three-dimensional momentum distribution$ f_{L_{j}}({\boldsymbol{p}}) $ is$ \begin{aligned}[b] f_{L_{j}}({\boldsymbol{p}})=& N_{h_1h_2h_3} \int {\rm d}{\boldsymbol{x}}_1d{\boldsymbol{x}}_2{\rm d}{\boldsymbol{x}}_3 {\rm d}{\boldsymbol{p}}_1 {\rm d}{\boldsymbol{p}}_2{\rm d}{\boldsymbol{p}}_3 f^{(n)}_{h_1h_2h_3}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3; \\ & {\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3) {\cal{R}}_{L_{j}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3, {\boldsymbol{p}}).\\[-20pt] \end{aligned} $

(18) $ N_{h_1h_2h_3} $ is the number of all possible$ h_1h_2h_3 $ -clusters and is equal to$ N_{h_1}N_{h_2}N_{h_3}, \; N_{h_1}(N_{h_1}-1)N_{h_3}, \; N_{h_1}(N_{h_1}-1)(N_{h_1}-2) $ for$ h_1 \neq h_2 \neq h_3 $ ,$ h_1 = h_2 \neq h_3 $ , and$ h_1=h_2=h_3 $ , respectively,$ f^{(n)}_{h_1h_2h_3} $ is the normalized three-hadron joint coordinate-momentum distribution, and$ {\cal{R}}_{L_{j}} $ is the kernel function.We rewrite the kernel function as
$ \begin{aligned}[b] &{\cal{R}}_{L_{j}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3, {\boldsymbol{p}}) \\=\;& g_{L_{j}} {\cal{R}}_{L_{j}}^{(x, p)}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3) \\ & \times \delta( {\sum^3_{i=1}} {\boldsymbol{p}}_i-{\boldsymbol{p}}). \end{aligned} $

(19) The spin degeneracy factor
$ g_{L_{j}} = (2J_{L_{j}}+1) /[\prod \limits_{i=1}^3(2J_{h_i}+1)] $ . The Dirac δ function guarantees momentum conservation.$ {\cal{R}}_{L_{j}}^{(x, p)}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3) $ obtained by solving from the Wigner transformation [60, 61] is$ \begin{aligned}[b] & {\cal{R}}^{(x, p)}_{L_{j}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3)\\ =\;& 8^2 {\rm e}^{-\tfrac{({\boldsymbol{x}}'_1-{\boldsymbol{x}}'_2)^2}{2\sigma_1^2}} {\rm e}^{-\tfrac{2(\frac{m_1{\boldsymbol{x}}'_1}{m_1+m_2}+\frac{m_2{\boldsymbol{x}}'_2}{m_1+m_2}-{\boldsymbol{x}}'_3)^2}{3\sigma_2^2}} \\ & \times {\rm e}^{-\tfrac{2\sigma_1^2(m_2{\boldsymbol{p}}'_{1}-m_1{\boldsymbol{p}}'_{2})^2}{(m_1+m_2)^2\hbar^2c^2}} {\rm e}^{-\tfrac{3\sigma_2^2[m_3{\boldsymbol{p}}'_{1}+m_3{\boldsymbol{p}}'_{2}-(m_1+m_2){\boldsymbol{p}}'_{3}]^2} {2(m_1+m_2+m_3)^2\hbar^2c^2}}. \end{aligned} $

(20) The superscript '
$ \prime $ ' denotes the hadronic coordinate or momentum in the rest frame of the$ h_1h_2h_3 $ -cluster. The width parameter$\begin{aligned}\\[-13pt]\sigma_1=\sqrt{\dfrac{m_3(m_1+m_2)(m_1+m_2+m_3)} {m_1m_2(m_1+m_2)+m_2m_3(m_2+m_3)+m_3m_1(m_3+m_1)}} R_{L_{j}} \end{aligned}$

and
$\sigma_2=\sqrt{\dfrac{4m_1m_2(m_1+m_2+m_3)^2} {3(m_1+m_2)[m_1m_2(m_1+m_2)+m_2m_3(m_2+m_3)+m_3m_1(m_3+m_1)]}} R_{L_{j}} $

With the coordinate and momentum factorization assumption of the joint distribution, we have
$ f_{L_{j}}({\boldsymbol{p}}) = N_{h_1h_2h_3} g_{L_{j}} {\cal{A}}_{L_{j}} {\cal{M}}_{L_{j}}({\boldsymbol{p}}). $

(21) Here, we also use
$ {\cal{A}}_{L_{j}} $ to denote the coordinate integral part as$ \begin{aligned}[b] {\cal{A}}_{L_{j}} = & 8^2\int {e}{\boldsymbol{x}}_1{e}{\boldsymbol{x}}_2{e}{\boldsymbol{x}}_3 f^{(n)}_{h_1h_2h_3}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3) {\rm e}^{-\tfrac{({\boldsymbol{x}}'_1-{\boldsymbol{x}}'_2)^2}{2\sigma_1^2}} \\ & \times {\rm e}^{-\tfrac{2(\frac{m_1{\boldsymbol{x}}'_1}{m_1+m_2}+\frac{m_2{\boldsymbol{x}}'_2}{m_1+m_2}-{\boldsymbol{x}}'_3)^2}{3\sigma_2^2}} , \end{aligned} $

(22) and use
$ {\cal{M}}_{L_{j}}({\boldsymbol{p}}) $ to denote the momentum integral part as$ \begin{aligned}[b] {\cal{M}}_{L_{j}}({\boldsymbol{p}}) = & \int {\rm d}{\boldsymbol{p}}_1{\rm d}{\boldsymbol{p}}_2{\rm d}{\boldsymbol{p}}_3 f^{(n)}_{h_1h_2h_3}({\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3) \delta\left( {\sum^3_{i=1}} {\boldsymbol{p}}_i-{\boldsymbol{p}}\right) \\ & \times {\rm e}^{-\tfrac{2\sigma_1^2(m_2{\boldsymbol{p}}'_{1}-m_1{\boldsymbol{p}}'_{2})^2}{(m_1+m_2)^2\hbar^2c^2}} {\rm e}^{-\tfrac{3\sigma_2^2[m_3{\boldsymbol{p}}'_{1}+m_3{\boldsymbol{p}}'_{2}-(m_1+m_2){\boldsymbol{p}}'_{3}]^2} {2(m_1+m_2+m_3)^2\hbar^2c^2}} . \end{aligned} $

(23) We change integral variables in Eq. (22) to be
$ {\boldsymbol{Y}}= (m_1{\boldsymbol{x}}_1+m_2{\boldsymbol{x}}_2+m_3{\boldsymbol{x}}_3)/(m_1+m_2+m_3) $ ,$ {\boldsymbol{r}}_1= ({\boldsymbol{x}}_1-{\boldsymbol{x}}_2)/ \sqrt{2} $ , and${\boldsymbol{r}}_2=\sqrt{\dfrac{2}{3}} \left(\dfrac{m_1{\boldsymbol{x}}_1}{m_1+m_2}+\dfrac{m_2{\boldsymbol{x}}_2}{m_1+m_2}-{\boldsymbol{x}}_3 \right)$ and further assume the coordinate joint distribution is coordinate variable factorized, i.e.,$ 3^{3/2} f^{(n)}_{h_1h_2h_3}({\boldsymbol{Y}}, {\boldsymbol{r}}_1, {\boldsymbol{r}}_2) = f^{(n)}_{h_1h_2h_3}({\boldsymbol{Y}}) f^{(n)}_{h_1h_2h_3}({\boldsymbol{r}}_1) f^{(n)}_{h_1h_2h_3}({\boldsymbol{r}}_2) $ . Adopting$f^{(n)}_{h_1h_2h_3}({\boldsymbol{r}}_1) = \dfrac{1}{(\pi C_1 R_f^2)^{3/2}} {\rm e}^{-\frac{{\boldsymbol{r}}_1^2}{C_1 R_f^2}}$ and$f^{(n)}_{h_1h_2h_3}({\boldsymbol{r}}_2) = \dfrac{1}{(\pi C_2 R_f^2)^{3/2}} {\rm e}^{-\frac{{\boldsymbol{r}}_2^2}{C_2 R_f^2}}$ , as in Refs. [51, 64], we obtain$ \begin{aligned}[b] {\cal{A}}_{L_{j}} =& 8^2 \frac{1}{(\pi C_1 R_f^2)^{3/2}} \int {\rm d}{\boldsymbol{r}}_1 {\rm e}^{-\frac{{\boldsymbol{r}}_1^2}{C_1 R_f^2}} {\rm e}^{-\frac{({\boldsymbol{r}}'_1)^2}{\sigma_1^2}} \\ & \times \frac{1}{(\pi C_2 R_f^2)^{3/2}} \int {\rm d}{\boldsymbol{r}}_2 {\rm e}^{-\frac{{\boldsymbol{r}}_2^2}{C_2 R_f^2}} {\rm e}^{-\frac{({\boldsymbol{r}}'_2)^2}{\sigma_2^2}}. \end{aligned} $

(24) Comparing relations of
$ {\boldsymbol{r}}_1 $ ,$ {\boldsymbol{r}}_2 $ with$ {\boldsymbol{x}}_1 $ ,$ {\boldsymbol{x}}_2 $ ,$ {\boldsymbol{x}}_3 $ to those of$ {\boldsymbol{r}} $ with$ {\boldsymbol{x}}_1 $ ,$ {\boldsymbol{x}}_2 $ in Sec. II.A, we find that$ C_1 $ is equal to$ C_w $ and$ C_2 $ is$ 4C_w/3 $ when ignoring the mass difference of$ m_1 $ and$ m_2 $ [51, 64]. Considering the Lorentz transformation and integrating from the relative coordinate variables in Eq. (24), we obtain$ \begin{aligned}[b] {\cal{A}}_{L_{j}} =& \frac{8^2 \sigma_1^3\sigma_2^3} { (C_1 R_f^2+\sigma_1^2) \sqrt{C_1 (R_f/\gamma)^2+\sigma_1^2} } \\ & \times \frac{1}{ (C_2 R_f^2+\sigma_2^2) \sqrt{C_2 (R_f/\gamma)^2+\sigma_2^2}} . \end{aligned} $

(25) Approximating the Gaussian form of the momentum-dependent kernel function to be the δ function form and integrating
$ {\boldsymbol{p}}_1 $ ,$ {\boldsymbol{p}}_2 $ , and$ {\boldsymbol{p}}_3 $ from Eq. (23), we obtain$ \begin{aligned}[b] & {\cal{M}}_{L_{j}}({\boldsymbol{p}}) = \left( \frac{\pi \hbar^2 c^2}{\sqrt{3}\sigma_1\sigma_2} \right)^3 \gamma^2 f^{(n)}_{h_1h_2h_3} \\ & \times \left(\frac{m_1{\boldsymbol{p}}}{m_1+m_2+m_3}, \frac{m_2{\boldsymbol{p}}}{m_1+m_2+m_3}, \frac{m_3{\boldsymbol{p}}}{m_1+m_2+m_3}\right).\end{aligned} $

(26) Substituting Eqs. (25) and (26) into Eq. (21) and ignoring correlations between the
$ h_1 $ ,$ h_2 $ , and$ h_3 $ hadrons, we have$ \begin{aligned}[b] f_{L_{j}}({\boldsymbol{p}}) =& \frac{64 \pi^3 \hbar^6 c^6 g_{L_{j}} \gamma^2}{3\sqrt{3}(C_1 R_f^2+\sigma_1^2) \sqrt{C_1 (R_f/\gamma)^2+\sigma_1^2}} \\ & \times \frac{1}{ (C_2 R_f^2+\sigma_2^2) \sqrt{C_2 (R_f/\gamma)^2+\sigma_2^2}} f_{h_1}\left(\frac{m_1{\boldsymbol{p}}}{m_1+m_2+m_3}\right) \\ & \times f_{h_2}\left(\frac{m_2{\boldsymbol{p}}}{m_1+m_2+m_3}\right) f_{h_3}\left(\frac{m_3{\boldsymbol{p}}}{m_1+m_2+m_3}\right) .\\[-19pt] \end{aligned} $

(27) Finally, we obtain the Lorentz invariant momentum distribution
$ \begin{aligned}[b] f_{L_j}^{({\rm{inv}})}(p_{T}, y) =\;& \frac{64 \pi^3 \hbar^6 c^6 g_{L_{j}} }{3\sqrt{3}(C_1 R_f^2+\sigma_1^2) \sqrt{C_1 (R_f/\gamma)^2+\sigma_1^2}} \\ & \times \frac{1}{ (C_2 R_f^2+\sigma_2^2) \sqrt{C_2 (R_f/\gamma)^2+\sigma_2^2}} \\&\times\frac{m_1+m_2+m_3}{m_1m_2m_3} \\ & \times f_{h_1}^{({\rm{inv}})}\left(\frac{m_1p_{T}}{m_1+m_2+m_3}, y\right)\\&\times f_{h_2}^{({\rm{inv}})}\left(\frac{m_2p_{T}}{m_1+m_2+m_3}, y\right) \\ &\times f_{h_3}^{({\rm{inv}})}\left(\frac{m_3p_{T}}{m_1+m_2+m_3}, y\right) . \end{aligned} $

(28) -
For 4He formed via the coalescence of four nucleons, the three-dimensional momentum distribution is
$ \begin{aligned}[b] f_{^4{\rm{He}}}({\boldsymbol{p}})=& N_{ppnn} \int {\rm d}{\boldsymbol{x}}_1{\rm d}{\boldsymbol{x}}_2{\rm d}{\boldsymbol{x}}_3{\rm d}{\boldsymbol{x}}_4 {\rm d}{\boldsymbol{p}}_1 {\rm d}{\boldsymbol{p}}_2{\rm d}{\boldsymbol{p}}_3{\rm d}{\boldsymbol{p}}_4 \\ & \times f^{(n)}_{ppnn}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3, {\boldsymbol{x}}_4;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3, {\boldsymbol{p}}_4) \\ & \times {\cal{R}}_{^4{\rm{He}}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3, {\boldsymbol{x}}_4;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3, {\boldsymbol{p}}_4, {\boldsymbol{p}}), \end{aligned} $

(29) where
$ N_{ppnn}=N_{p}(N_{p}-1)N_{n}(N_{n}-1) $ is the number of all possible$ ppnn $ -clusters,$ f^{(n)}_{ppnn} $ is the normalized four-nucleon joint coordinate-momentum distribution, and$ {\cal{R}}_{^4{\rm{He}}} $ is the kernel function.We rewrite the kernel function as
$ \begin{aligned}[b] & {\cal{R}}_{^4{\rm{He}}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3, {\boldsymbol{x}}_4;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3, {\boldsymbol{p}}_4, {\boldsymbol{p}}) = g_{^4{\rm{He}}} \\ & \; \; \; \; \times {\cal{R}}_{^4{\rm{He}}}^{(x, p)}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3, {\boldsymbol{x}}_4;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3, {\boldsymbol{p}}_4) \delta( {\sum^4_{i=1}} {\boldsymbol{p}}_i-{\boldsymbol{p}}), \end{aligned} $

(30) where the spin degeneracy factor
$ g_{^4{\rm{He}}}=1/16 $ , and$ \begin{aligned}[b] & {\cal{R}}^{(x, p)}_{^4{\rm{He}}}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3, {\boldsymbol{x}}_4;{\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3, {\boldsymbol{p}}_4)=8^3{\rm e}^{-\frac{({\boldsymbol{x}}'_1-{\boldsymbol{x}}'_2)^2}{2\sigma_{^4{\rm{He}}}^2}} \\ &\; \; \; \; \; \; \; \; \; \times {\rm e}^{-\frac{({\boldsymbol{x}}'_1+{\boldsymbol{x}}'_2-2{\boldsymbol{x}}'_3)^2}{6\sigma_{^4{\rm{He}}}^2}} {\rm e}^{-\frac{({\boldsymbol{x}}'_1+{\boldsymbol{x}}'_2+{\boldsymbol{x}}'_3-3{\boldsymbol{x}}'_4)^2}{12\sigma_{^4{\rm{He}}}^2}} \\ &\; \; \; \; \; \; \; \; \; \times {\rm e}^{-\frac{\sigma_{^4{\rm{He}}}^2({\boldsymbol{p}}'_{1}-{\boldsymbol{p}}'_{2})^2}{2\hbar^2c^2}} {\rm e}^{-\frac{\sigma_{^4{\rm{He}}}^2({\boldsymbol{p}}'_{1}+{\boldsymbol{p}}'_{2}-2{\boldsymbol{p}}'_{3})^2}{6\hbar^2c^2}} {\rm e}^{-\frac{\sigma_{^4{\rm{He}}}^2({\boldsymbol{p}}'_{1}+{\boldsymbol{p}}'_{2}+{\boldsymbol{p}}'_{3}-3{\boldsymbol{p}}'_{4})^2}{12\hbar^2c^2}}. \end{aligned} $

(31) Here,
$ \sigma_{^4{\rm{He}}}=\dfrac{2\sqrt{2}}{3}R_{^4{\rm{He}}} $ , and$ R_{^4{\rm{He}}}=1.6755 $ fm [62] is the root-mean-square radius of 4He.Assuming that the normalized joint distribution is coordinate and momentum factorized, we have
$ f_{^4{\rm{He}}}({\boldsymbol{p}}) = N_{ppnn} g_{^4{\rm{He}}} {\cal{A}}_{^4{\rm{He}}} {\cal{M}}_{^4{\rm{He}}}({\boldsymbol{p}}). $

(32) Here, we use
$ {\cal{A}}_{^4{\rm{He}}} $ to denote the coordinate integral part in Eq. (32) as$ \begin{aligned}[b] {\cal{A}}_{^4{\rm{He}}} =\; & 8^3 \int {\rm d}{\boldsymbol{x}}_1{\rm d}{\boldsymbol{x}}_2d{\boldsymbol{x}}_3{\rm d}{\boldsymbol{x}}_4 f^{(n)}_{ppnn}({\boldsymbol{x}}_1, {\boldsymbol{x}}_2, {\boldsymbol{x}}_3, {\boldsymbol{x}}_4) \\ &\times {\rm e}^{-\frac{({\boldsymbol{x}}'_1-{\boldsymbol{x}}'_2)^2}{2\sigma_{^4{\rm{He}}}^2}} {\rm e}^{-\frac{({\boldsymbol{x}}'_1+{\boldsymbol{x}}'_2-2{\boldsymbol{x}}'_3)^2}{6\sigma_{^4{\rm{He}}}^2}} {\rm e}^{-\frac{({\boldsymbol{x}}'_1+{\boldsymbol{x}}'_2+{\boldsymbol{x}}'_3-3{\boldsymbol{x}}'_4)^2}{12\sigma_{^4{\rm{He}}}^2}} , \end{aligned} $

(33) and use
$ {\cal{M}}_{^4{\rm{He}}}({\boldsymbol{p}}) $ to denote the momentum integral part as$ \begin{aligned}[b] {\cal{M}}_{^4{\rm{He}}}({\boldsymbol{p}}) =\;& \int {\rm d}{\boldsymbol{p}}_1{\rm d}{\boldsymbol{p}}_2{\rm d}{\boldsymbol{p}}_3{\rm d}{\boldsymbol{p}}_4 f^{(n)}_{ppnn}({\boldsymbol{p}}_1, {\boldsymbol{p}}_2, {\boldsymbol{p}}_3, {\boldsymbol{p}}_4) \\ & \times {\rm e}^{-\tfrac{\sigma_{^4{\rm{He}}}^2({\boldsymbol{p}}'_{1}-{\boldsymbol{p}}'_{2})^2}{2\hbar^2c^2}} {\rm e}^{-\tfrac{\sigma_{^4{\rm{He}}}^2({\boldsymbol{p}}'_{1}+{\boldsymbol{p}}'_{2}-2{\boldsymbol{p}}'_{3})^2}{6\hbar^2c^2}}\\ &\times {\rm e}^{-\tfrac{\sigma_{^4{\rm{He}}}^2({\boldsymbol{p}}'_{1}+{\boldsymbol{p}}'_{2}+{\boldsymbol{p}}'_{3}-3{\boldsymbol{p}}'_{4})^2}{12\hbar^2c^2}} \delta\left( {\sum^4_{i=1}} {\boldsymbol{p}}_i-{\boldsymbol{p}}\right). \end{aligned} $

(34) We change the integral variables in Eq. (33) to be
$ {\boldsymbol{Z}}= ({\boldsymbol{x}}_1+{\boldsymbol{x}}_2+{\boldsymbol{x}}_3+{\boldsymbol{x}}_4)/2 $ ,$ {\boldsymbol{r}}_1= ({\boldsymbol{x}}_1-{\boldsymbol{x}}_2)/\sqrt{2} $ ,$ {\boldsymbol{r}}_2= ({\boldsymbol{x}}_1+{\boldsymbol{x}}_2- 2{\boldsymbol{x}}_3)/ \sqrt{6} $ , and$ {\boldsymbol{r}}_3= ({\boldsymbol{x}}_1+{\boldsymbol{x}}_2+{\boldsymbol{x}}_3-3{\boldsymbol{x}}_4)/\sqrt{12} $ and assume$ f^{(n)}_{ppnn}({\boldsymbol{Z}}, {\boldsymbol{r}}_1, {\boldsymbol{r}}_2, {\boldsymbol{r}}_3) = f^{(n)}_{ppnn}({\boldsymbol{Z}}) f^{(n)}_{ppnn}({\boldsymbol{r}}_1) f^{(n)}_{ppnn}({\boldsymbol{r}}_2) $ $ f^{(n)}_{ppnn}({\boldsymbol{r}}_3) $ . Adopting$f^{(n)}_{ppnn}({\boldsymbol{r}}_1) = \frac{1}{(\pi C_1 R_f^2)^{3/2}} {\rm e}^{-\frac{{\boldsymbol{r}}_1^2}{C_1 R_f^2}}$ ,$f^{(n)}_{ppnn}({\boldsymbol{r}}_2) = \frac{1}{(\pi C_2 R_f^2)^{3/2}} {\rm e}^{-\frac{{\boldsymbol{r}}_2^2}{C_2 R_f^2}}$ , and$f^{(n)}_{ppnn}({\boldsymbol{r}}_3) = \frac{1}{(\pi C_3 R_f^2)^{3/2}} {\rm e}^{-\frac{{\boldsymbol{r}}_3^2}{C_3 R_f^2}}$ , we obtain$ \begin{aligned}[b] {\cal{A}}_{^4{\rm{He}}} =\;& 8^3\int {\rm d}{\boldsymbol{r}}_1{\rm d}{\boldsymbol{r}}_2{\rm d}{\boldsymbol{r}}_3 f^{(n)}_{ppnn}({\boldsymbol{r}}_1)f^{(n)}_{ppnn}({\boldsymbol{r}}_2)f^{(n)}_{ppnn}({\boldsymbol{r}}_3) \\ & \times {\rm e}^{-\frac{({\boldsymbol{r}}'_1)^2}{\sigma_{^4{\rm{He}}}^2}} {\rm e}^{-\frac{({\boldsymbol{r}}'_2)^2}{\sigma_{^4{\rm{He}}}^2}} {\rm e}^{-\frac{({\boldsymbol{r}}'_3)^2}{\sigma_{^4{\rm{He}}}^2}}. \end{aligned} $

(35) $ C_1 $ ,$ C_2 $ , and$ C_3 $ are equal to$ C_w $ ,$ 4C_w/3 $ , and$ 3C_w/2 $ , respectively [51, 64]. After the Lorentz transformation and integrating the relative coordinate variables from Eq. (35), we obtain$ \begin{aligned}[b] {\cal{A}}_{^4{\rm{He}}} =& \frac{8^3 \sigma_{^4{\rm{He}}}^9} {(C_1 R_f^2+\sigma_{^4{\rm{He}}}^2) \sqrt{C_1 (R_f/\gamma)^2+\sigma_{^4{\rm{He}}}^2} } \\ & \times \frac{1}{(C_2 R_f^2+\sigma_{^4{\rm{He}}}^2) \sqrt{C_2 (R_f/\gamma)^2+\sigma_{^4{\rm{He}}}^2} } \\ & \times \frac{1}{(C_3 R_f^2+\sigma_{^4{\rm{He}}}^2) \sqrt{C_3 (R_f/\gamma)^2+\sigma_{^4{\rm{He}}}^2}} . \end{aligned} $

(36) Approximating the Gaussian form of the momentum-dependent kernel function as the δ function form and after integrating
$ {\boldsymbol{p}}_1 $ ,$ {\boldsymbol{p}}_2 $ ,$ {\boldsymbol{p}}_3 $ , and$ {\boldsymbol{p}}_4 $ in Eq. (34), we obtain$ \begin{aligned}[b]{\cal{M}}_{^4{\rm{He}}}({\boldsymbol{p}}) = & \left(\frac{\pi^{3/2}\hbar^3 c^3}{2\sigma_{^4{\rm{He}}}^3}\right)^3 \gamma^3 f^{(n)}_{p}\left(\frac{{\boldsymbol{p}}}{4}\right) f^{(n)}_{p}\left(\frac{{\boldsymbol{p}}}{4}\right)\\ & \times f^{(n)}_{n}\left(\frac{{\boldsymbol{p}}}{4}\right) f^{(n)}_{n}\left(\frac{{\boldsymbol{p}}}{4}\right) . \end{aligned}$

(37) Substituting Eqs. (36) and (37) into Eq. (32), we have
$ \begin{aligned}[b] f_{^4{\rm{He}}}({\boldsymbol{p}}) =&\frac{64 g_{^4{\rm{He}}} \gamma^3 \pi^{9/2}\hbar^9 c^9} {(C_1 R_f^2+\sigma_{^4{\rm{He}}}^2) \sqrt{C_1 (R_f/\gamma)^2+\sigma_{^4{\rm{He}}}^2} } \\ & \times \frac{1}{(C_2 R_f^2+\sigma_{^4{\rm{He}}}^2) \sqrt{C_2 (R_f/\gamma)^2+\sigma_{^4{\rm{He}}}^2} } \\ & \times \frac{1}{ (C_3 R_f^2+\sigma_{^4{\rm{He}}}^2) \sqrt{C_3 (R_f/\gamma)^2+\sigma_{^4{\rm{He}}}^2}} \\ & \times f_{p}(\frac{{\boldsymbol{p}}}{4}) f_{p}(\frac{{\boldsymbol{p}}}{4}) f_{n}(\frac{{\boldsymbol{p}}}{4}) f_{n}(\frac{{\boldsymbol{p}}}{4}) . \end{aligned} $

(38) Finally, we obtain the Lorentz invariant momentum distribution
$ \begin{aligned}[b] & f_{^4{\rm{He}}}^{({\rm{inv}})}(p_{T}, y) = \frac{256 g_{^4{\rm{He}}} \pi^{9/2}\hbar^9 c^9} {m^3 (C_1 R_f^2+\sigma_{^4{\rm{He}}}^2) \sqrt{C_1 (R_f/\gamma)^2+\sigma_{^4{\rm{He}}}^2} } \\ &\; \; \times \frac{1}{(C_2 R_f^2+\sigma_{^4{\rm{He}}}^2) \sqrt{C_2 (R_f/\gamma)^2+\sigma_{^4{\rm{He}}}^2} } \\ &\; \; \times \frac{1}{ (C_3 R_f^2+\sigma_{^4{\rm{He}}}^2) \sqrt{C_3 (R_f/\gamma)^2+\sigma_{^4{\rm{He}}}^2}} \\ &\; \; \times f_{p}^{({\rm{inv}})}(\frac{p_{T}}{4}, y) f_{p}^{({\rm{inv}})}(\frac{p_{T}}{4}, y) f_{n}^{({\rm{inv}})}(\frac{p_{T}}{4}, y) f_{n}^{({\rm{inv}})}(\frac{p_{T}}{4}, y) , \; \; \; \end{aligned} $

(39) where m is the nucleon mass.
In summary, Eqs. (17), (28), and (39) provide the relationships of light nuclei with primordial hadronic bodies in momentum space in the laboratory frame. They can be directly used to calculate the yields and
$ p_T $ distributions of light nuclei formed via different coalescence channels as long as the primordial hadronic momentum distributions are given. When ignoring the mass differences of primordial hadrons, Eqs. (17) and (28) return to our previous results for d, t, and 3He in Refs. [51, 58], where only nucleon coalescence was considered. -
In this section, we apply the coalescence model from Sec. II to Au-Au collisions at
$ \sqrt{s_{NN}}=3 $ GeV to study the momentum and centrality dependence of the production of different light nuclei in the low- and intermediate-$ p_T $ regions at different rapidity intervals in the midrapidity area. We first introduce the$ p_T $ spectra of the nucleons and then present the$ p_T $ dependence of different coalescence sources for d, t, 3He, and 4He. We finally give the yield rapidity densities${\rm d}N/{\rm d}y$ , yield ratios, and averaged transverse momenta$ \langle p_T \rangle $ of different light nuclei. -
The invariant
$ p_T $ distributions at different rapidity intervals of primordial protons$ f_{p, {\rm{pri}}}^{({\rm{inv}})}(p_{T}, y) $ and neutrons$ f_{n, {\rm{pri}}}^{({\rm{inv}})}(p_{T}, y) $ are necessary inputs for computing the$ p_T $ distributions of light nuclei in our model. The relationship between primordial and final-state protons is as follows:$ f_{p, {\rm{pri}}}^{({\rm{inv}})}(p_{T}, y) - f_{p, {\rm{lignucl}}}^{({\rm{inv}})}(p_{T}, y) + f_{p, {\rm{hypdec}}}^{({\rm{inv}})}(p_{T}, y)= f_{p, {\rm{fin}}}^{({\rm{inv}})}(p_{T}, y). $

(40) The last three terms denote the invariant
$ p_T $ distributions of protons consumed in light nucleus production, protons from hyperon weak decays, and final-state protons. The feed-down contribution from the weak decays of hyperons to protons in the midrapidity area is approximately 1% [55] and that entering light nuclei takes approximately 20% [55]. Considering that most primordial protons (approximately 80%) evolve into final-state ones, we ignore the variation in the shape of the$ p_T $ spectra of primordial and final-state protons. In this case, we can obtain$ f_{p, {\rm{pri}}}^{({\rm{inv}})}(p_{T}, y) \approx \dfrac{1}{80\%}[f_{p, {\rm{fin}}}^{({\rm{inv}})}(p_{T}, y)-f_{p, {\rm{hypdec}}}^{({\rm{inv}})}(p_{T}, y)] $ .Here, we use the blast-wave model to obtain the invariant
$ p_T $ distribution functions of final-state protons minus those from hyperon weak decays by fitting the measured primordial proton data from Ref. [55]. The blast-wave function [65] is given as$ \begin{aligned}[b] f_{p}^{({\rm{inv}})}(p_{T}, y) = & \frac{{\rm d}^{2}N_p}{2\pi p_{T}{\rm d}p_{T}{\rm d}y} \propto \int_{0}^{R} r {\rm d}r m_T I_0 \left(\frac{p_T{\rm sin}h\rho}{T_{\rm kin}}\right) \\ & \times K_1\left(\frac{m_T{\rm cosh}\rho}{T_{\rm kin}}\right) , \end{aligned} $

(41) where r is the radial distance in the transverse plane, R is the radius of the fireball,
$ m_T $ is the transverse mass of the proton,$ I_0 $ and$ K_1 $ are the modified Bessel functions, and the velocity profile$\rho={\rm tanh}^{-1}[\beta_s(\dfrac{r}{R})^n]$ . The surface velocity$ \beta_s $ , the kinetic freeze-out temperature$T_{\rm kin}$ , and n are fitting parameters.Figure 1 shows the invariant
$ p_T $ spectra of primordial protons measured experimentally at the different rapidity intervals$ -0.1<y<0 $ ,$ -0.2<y<-0.1 $ ,$ -0.3<y<-0.2 $ ,$ -0.4<y<-0.3 $ , and$ -0.5<y<-0.4 $ in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV for the centralities 0−10%, 10%−20%, 20%−40%, and 40%−80%. The spectra at different rapidity intervals are scaled by different factors for clarity, as shown in the figure. The filled symbols are experimental data from the STAR collaboration [55]. Different lines are the results of the blast-wave model. Because we focus on testing the validity of the coalescence mechanism in describing light nucleus production at low collision energies, we first employ the best fit of the blast-wave model for the protons to study the fundamental observables of light nuclei, e.g., the$ p_T $ spectra, yield rapidity densities${\rm d}N/{\rm d}y$ , and averaged transverse momenta$ \langle p_T \rangle $ . Theoretical uncertainties from the blast-wave fitting errors are discussed later. Note that the protons shown in Fig. 1 are the primordial ones measured in the experiment. Divided by 80%, we can obtain$ f_{p, {\rm{pri}}}^{({\rm{inv}})}(p_{T}, y) $ , which is the input in the coalescence model, to compute light nucleus production.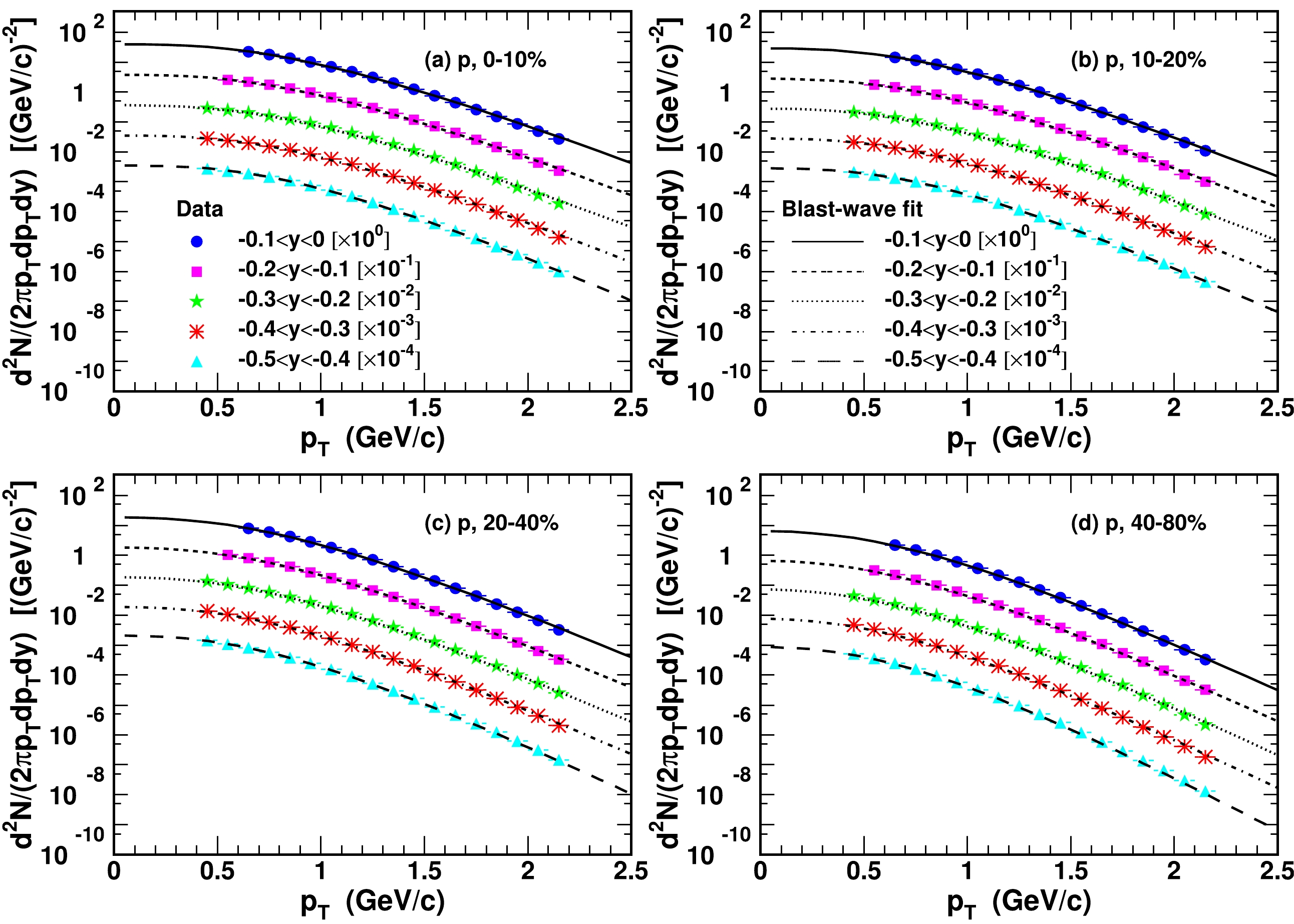
Figure 1. (color online) Invariant
$ p_T $ spectra of primordial protons measured experimentally at different rapidity intervals in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV in centralities (a) 0−10%, (b) 10%−20%, (c) 20%−40%, and (d) 40%−80%. The filled symbols are experimental data [55]. Different lines are the fitting results from the blast-wave model.For the neutron, we assume the same normalized
$ p_T $ distribution as that of the proton at the same rapidity interval and collision centrality. The absolute yield density of the neutron is generally not equal to that of the proton owing to the prominent influences of net nucleons from the colliding Au nuclei. Here, we use$ Z_{np} $ to denote the extent of the yield density asymmetry of the neutron and proton and take their relationship as$ \frac{{\rm d}N_n}{{\rm d}y}=\frac{{\rm d}N_p}{{\rm d}y}\times Z_{np}. $

(42) $ Z_{np}=1 $ corresponds to complete isospin equilibration, and$ Z_{np}=1.49 $ corresponds to isospin asymmetry in the entire Au nucleus. We set$ Z_{np} $ to be a free parameter, and its values in different centrality and rapidity windows are shown in Table 1, which are fixed by the central values of the experimental data of the ratio$ t/{}^{3} {\rm{He}}$ . The uncertainty of$ Z_{np} $ from the experimental errors of$ t/{}^{3} {\rm{He}}$ is approximately 0.1. The values of$ Z_{np} $ in the central and semi-central 0−10%, 10%−20%, and 20%−40% centralities are comparable and close to those evaluated in Ref. [66].$ Z_{np} $ in the 40%−80% centrality is slightly smaller. From the viewpoint of the neutron skin effect [67],$ Z_{np} $ is expected to increase in peripheral collisions. However, note that we study the light nucleus production in the midrapidity area, i.e.,$ y<0.5 $ , and in peripheral collisions, the transparency of nucleons from the colliding nuclei becomes stronger owing to the smaller reaction area and they move to relatively larger rapidity [55]. The participant nucleons from colliding nuclei become fewer in the midrapidity region; hence, the extent of yield asymmetry due to the participant nucleons decreases.Centrality Rapidity $ Z_{np} $ 

$ R_f $ /fm

0−10% $ -0.1<y<0 $ 

1.34 3.27 $ -0.2<y<-0.1 $ 

1.34 3.20 $ -0.3<y<-0.2 $ 

1.24 3.07 $ -0.4<y<-0.3 $ 

1.32 3.06 $ -0.5<y<-0.4 $ 

1.33 3.05 10−20% $ -0.1<y<0 $ 

1.33 2.93 $ -0.2<y<-0.1 $ 

1.33 2.81 $ -0.3<y<-0.2 $ 

1.26 2.75 $ -0.4<y<-0.3 $ 

1.30 2.74 $ -0.5<y<-0.4 $ 

1.33 2.70 20−40% $ -0.1<y<0 $ 

1.34 2.51 $ -0.2<y<-0.1 $ 

1.30 2.38 $ -0.3<y<-0.2 $ 

1.24 2.31 $ -0.4<y<-0.3 $ 

1.27 2.30 $ -0.5<y<-0.4 $ 

1.27 2.29 40−80% $ -0.1<y<0 $ 

1.14 1.39 $ -0.2<y<-0.1 $ 

1.11 1.32 $ -0.3<y<-0.2 $ 

1.00 1.30 $ -0.4<y<-0.3 $ 

1.04 1.27 $ -0.5<y<-0.4 $ 

1.03 1.26 Table 1. Values of
$ Z_{np} $ and$ R_f $ at different rapidity intervals and different centralities in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV.The other parameter in our model is
$ R_f $ , which is fixed by the data of the d yield rapidity density [55], and the fixing uncertainty is approximately 5%. The values of$ R_f $ at different rapidity intervals and centralities in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV are listed in Table 1. For the 0−10% centrality, the fixed values are in the range evaluated by the linear dependence on the cube root of the rapidity density of charged particles, i.e.,$R_f \propto ({\rm d}N_{ch}/{\rm d}y)^{1/3}$ [58, 68]. For other collision centralities,$ R_f $ cannot be evaluated by the relation$ R_f \propto ({\rm d}N_{ch}/{\rm d}y)^{1/3} $ owing to the current lack of data on$ \pi^{\pm } $ and$ K^{\pm } $ . As shown in Table 1,$ R_f $ decreases slightly with increasing rapidity for the same centrality and decreases from central to peripheral collisions. The smaller$ R_f $ in more peripheral collisions leads to stronger suppression of light nucleus production because of the non-negligible light nucleus sizes compared to$ R_f $ , as shown in Eqs. (17), (28), and (39). This suppression effect of light nucleus production in small collision systems has been systematically studied in Ref. [69]. -
Using Eq. (17), we first compute the invariant
$ p_T $ distributions of deuterons at the rapidity intervals$ -0.1<y<0 $ ,$ -0.2<y<-0.1 $ ,$ -0.3<y<-0.2 $ ,$ -0.4<y< -0.3 $ , and$ -0.5<y<-0.4 $ in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV in the centralities 0−10%, 10%−20%, 20%−40%, and 40%−80%. Here,$ h_1 $ and$ h_2 $ in Eq. (17) refer to the proton and neutron, respectively. The different lines scaled by different factors for clarity in Fig. 2 are our theoretical results for final-state deuterons, which refer to the results obtained by subtracting those consumed in nucleus coalescence from those formed via$ p+n $ coalescence in the 0−10%, 10%−20%, and 20%−40% centralities and those formed via$ p+n $ coalescence owing to the absence of nucleus coalescence in the 40%−80% centrality. The disappearance of nucleus coalescence in the 40%−80% centrality is discussed in detail later. The filled symbols with error bars are experimental data from the STAR collaboration [55]. As shown in Fig. 2, our results can effectively reproduce the available data at different rapidity intervals in the midrapidity area from central to peripheral Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV.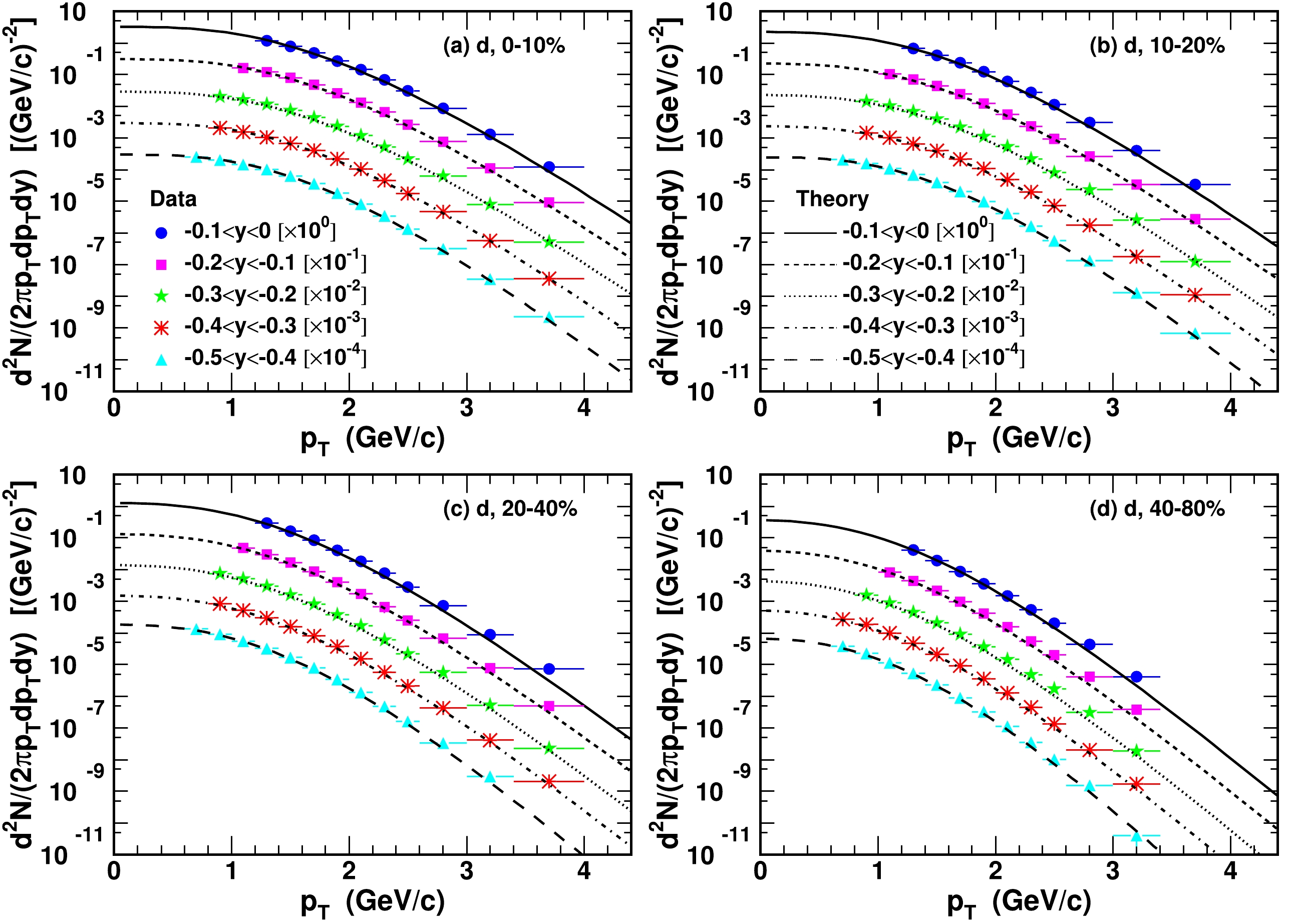
Figure 2. (color online) Invariant
$ p_T $ spectra of final-state deuterons at different rapidity intervals in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV in the centralities (a) 0−10%, (b) 10%−20%, (c) 20%−40%, and (d) 40%−80%. The filled symbols are experimental data [55], and different lines are the theoretical results.We then study the invariant
$ p_T $ distributions of t, 3He, and 4He at the rapidity intervals$ -0.1<y<0 $ ,$ -0.2<y< -0.1 $ ,$ -0.3<y<-0.2 $ ,$ -0.4<y<-0.3 $ , and$ -0.5<y<-0.4 $ in Au-Au collisions at$ \sqrt{s_{NN}}= $ 3 GeV in the centralities 0−10%, 10%−20%, 20%−40%, and 40%−80%. Figure 3 shows the invariant$ p_T $ spectra of tritons. The spectra at different rapidity intervals are scaled by different factors for clarity, as shown in the figure. The filled symbols with error bars are experimental data from the STAR collaboration [55]. The dashed lines are the results of nucleon coalescence, i.e., the contribution of the channel$ n+n+p \rightarrow t $ , the dotted lines are the results of$ n+d $ coalescence, and the solid lines are the final results of$ n+n+p $ coalescence plus$ n+d $ coalescence minus those of$ p+t $ coalescence. Panels (a), (b), and (c) in Fig. 3 show that the results of$ n+n+p $ coalescence plus$ n+d $ coalescence minus those of$ p+t $ coalescence can describe the available data well for central and semi-central Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV, whereas panel (d) shows that triton production in peripheral 40%−80% Au-Au collisions favors$ n+n+p $ coalescence.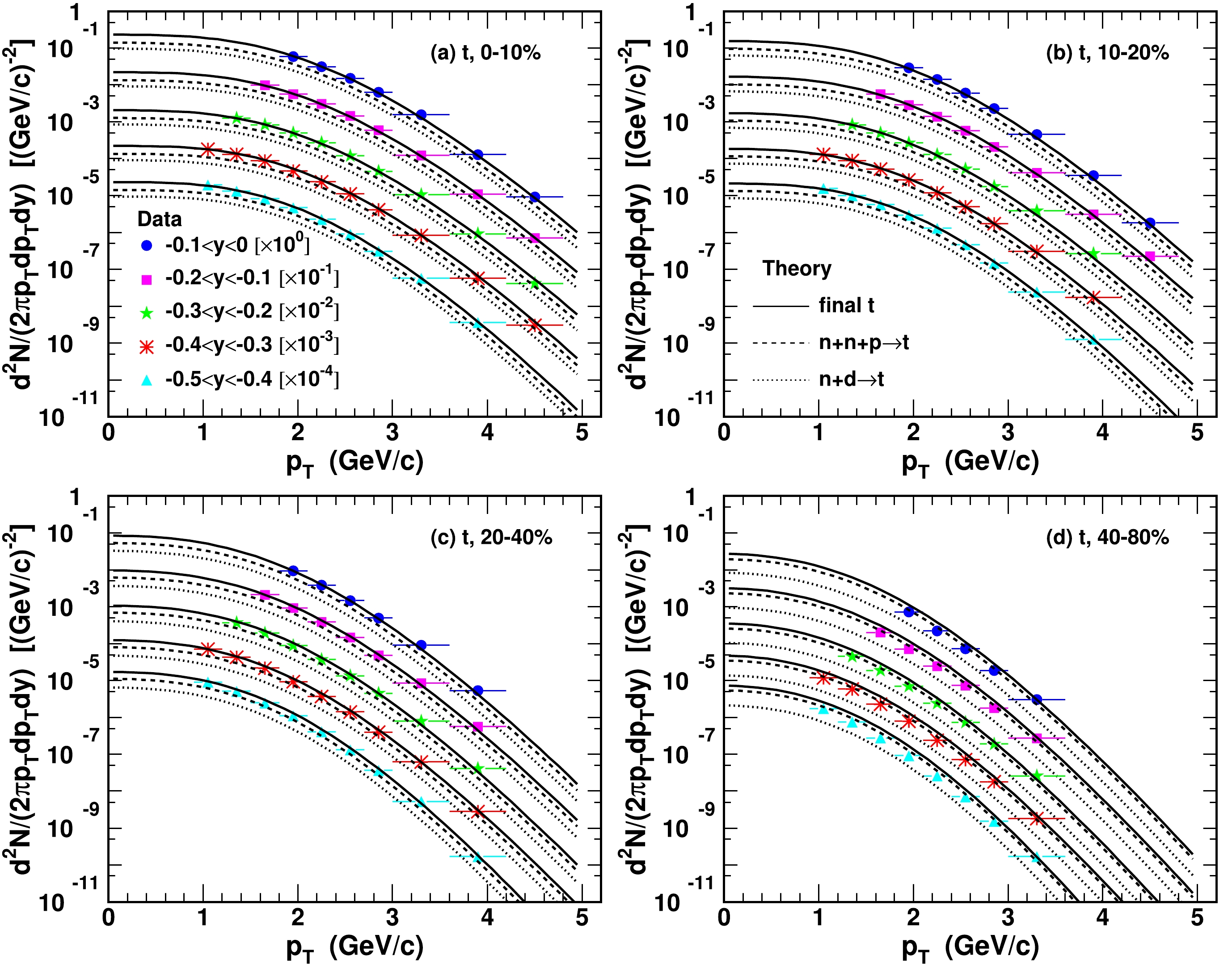
Figure 3. (color online) Invariant
$ p_T $ spectra of tritons at different rapidity intervals in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV in the centralities (a) 0−10%, (b) 10%−20%, (c) 20%−40%, and (d) 40%−80%. The filled symbols are experimental data [55]. The solid, dashed, and dotted lines are the theoretical results of final tritons,$ n+n+p $ coalescence, and$ n+d $ coalescence, respectively.Figure 4 shows the invariant
$ p_T $ spectra of 3He. The spectra at different rapidity intervals are also scaled by different factors for clarity, as shown in the figure. The filled symbols with error bars are experimental data from the STAR collaboration [55]. The dashed lines are the results of nucleon coalescence, i.e., the contribution of the channel$ p+p+n \rightarrow {}^{3} {\rm{He}}$ , the dotted lines are the results of$ p+d $ coalescence, and the solid lines are the final results of$ p+p+n $ coalescence plus$ p+d $ coalescence minus those of$ n+{}^{3} {\rm{He}}$ coalescence. As shown in panels (a), (b), and (c) in Fig. 4, the results of$ p+p+n $ coalescence plus$ p+d $ coalescence minus those of$ n+{}^{3} {\rm{He}}$ coalescence can describe the available data well for central and semi-central Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV. However, panel (d) in Fig. 4 shows that 3He production in peripheral Au-Au collisions favors$ p+p+n $ coalescence. This is similar to the result of the triton.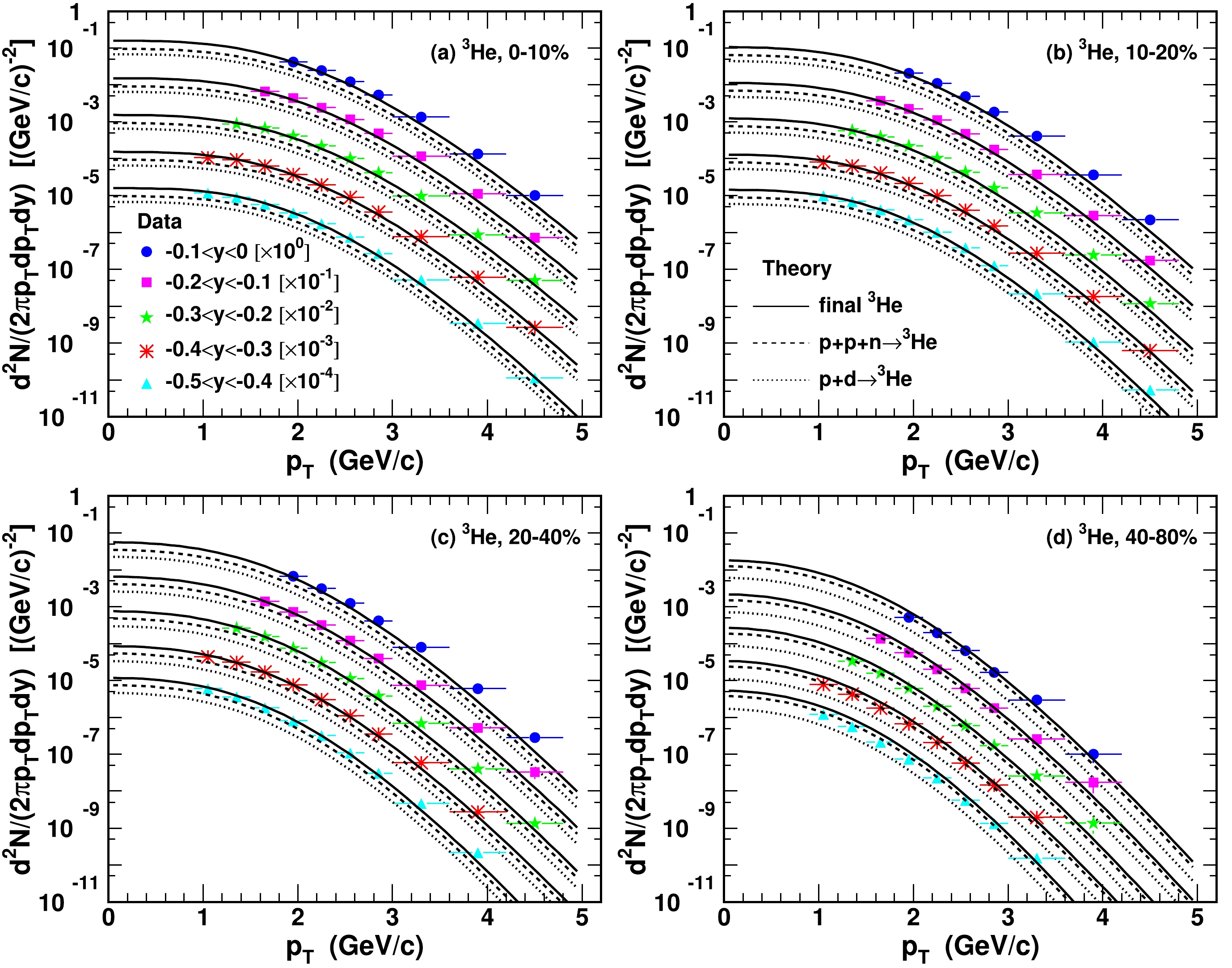
Figure 4. (color online) Invariant
$ p_T $ spectra of 3He at different rapidity intervals in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV in the centralities (a) 0−10%, (b) 10%−20%, (c) 20%−40%, and (d) 40%−80%. The filled symbols are experimental data [55]. The solid, dashed, and dotted lines are the theoretical results of final 3He,$ p+p+n $ coalescence, and$ p+d $ coalescence, respectively.Figure 5 shows the invariant
$ p_T $ spectra of 4He. The spectra at different rapidity intervals are scaled by different factors for clarity, as shown in the figure. The filled symbols with error bars are experimental data from the STAR collaboration [55]. The short-dashed lines are the results of nucleon coalescence, i.e., the contribution of the channel$ p+p+n+n \rightarrow {}^{4} {\rm{He}}$ , and the long-dashed lines are the results of the contributions from the channel$ p+t \rightarrow {}^{4} {\rm{He}}$ . The large-gap dotted lines are the results of the contributions from the channel$ n+{}^{3} {\rm{He}}$ $ \rightarrow {}^{4} {\rm{He}}$ , and the small-gap dotted lines are the results of the contributions from the channel$ d+d\rightarrow {}^{4} {\rm{He}}$ . The dashed-dotted lines are the results of the contributions from the channel$ p+n+d\rightarrow {}^{4} {\rm{He}}$ , and the solid lines are the total results including the above five coalescence channels. As shown in panels (a), (b), and (c) in Fig. 5, the total results including the above five coalescence processes can describe the available data for central and semi-central Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV. However, panel (d) in Fig. 5 shows that 4He production in peripheral Au-Au collisions favors nucleon coalescence, i.e.,$ p+p+n+n $ coalescence. The other four coalescence cases involving nucleon+nucleus or nucleus+nucleus coalescence may not occur.
Figure 5. (color online) Invariant
$ p_T $ spectra of 4He at different rapidity intervals in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV in the centralities (a) 0−10%, (b) 10%−20%, (c) 20%−40%, and (d) 40%−80%. The filled symbols are experimental data [55]. The different lines are the theoretical results.When calculating contributions from different coalescence channels, we apply the hypothesis that nucleon coalescence first occurs and the formed lighter cluster subsequently captures other particles to form a heavier cluster if they meet the coalescence requirements in the phase space. This coalescence time order is constrained to local freeze-out instead of the entire phase space. The results in Figs. 2, 3 , and 4 show that our final results of the
$ p_T $ spectra of d, t, and 3He can describe the experimental data in the 0−10%, 10%−20%, and 20%−40% centralities, whereas in the 40%−80% centrality, our results on nucleon coalescence itself can reproduce the available data. The results in Fig. 5 show that our total results on nucleon coalescence plus nucleon$ +d $ (t, 3He) coalescence plus$ d+d $ coalescence can describe the data of the$ p_T $ spectra of 4He in the 0−10%, 10%−20%, and 20%−40% centralities, whereas in the 40%−80% centrality, nucleon coalescence itself can reproduce the 4He data. This indicates that besides nucleon coalescence, nucleon/nucleus+nucleus coalescence plays an important role in central and semicentral collisions. However, in peripheral collisions, nucleus coalescence seems to disappear. This is probably because the interactions between hadronic rescatterings become insufficiently strong for the formed light nuclei to capture other particles to form heavier objects. -
To observe the contribution proportions of the different coalescence sources of t, 3He, and 4He in their production and the depletion proportions of d, t, and 3He more clearly, we study the yield rapidity densities
${\rm d}N/{\rm d}y$ of light nuclei. After integrating over$ p_T $ , we obtain${\rm d}N/{\rm d}y$ . Table 2 shows our results of d, and Table 3 shows those of t and 3He at different rapidity intervals and centralities in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV. The data with errors are from Ref. [55], and the errors denote the systematic uncertainties.$ {\rm{Theo}}_{pn} $ in the fourth column in Table 2 denotes the result of$ p+n $ coalescing into d.$ {\rm{Theo}}_{nnp} $ and$ {\rm{Theo}}_{nd} $ in the fourth and fifth columns in Table 3 denote the result of$ n+n+p $ coalescing into t and that of$ n+d $ coalescing into t, respectively.$ {\rm{Theo}}_{ppn} $ and$ {\rm{Theo}}_{pd} $ in the ninth and tenth columns in Table 3 denote the result of$ p+p+n $ coalescing into 3He and that of$ p+d $ coalescing into 3He, respectively.$ {\rm{Theo}}_{{\rm{dep}}} $ in the fifth column of Table 2 and the sixth and eleventh columns of Table 3 denote the consumed d, t, and 3He in the nucleus coalescence process, respectively, where they capture other particles to form objects with larger mass numbers.$ {\rm{Theo}}_{{\rm{fin}}} $ in the sixth column of Table 2 and the seventh and twelfth columns of Table 3 denote the final-state d, t, and 3He, respectively. As shown in Tables 2 and 3, our results for$ {\rm{Theo}}_{{\rm{fin}}} $ agree well with the experimental data in the 0−10%, 10%−20%, and 20%−40% centralities. However, in the peripheral 40%−80% centrality, our$ {\rm{Theo}}_{{\rm{fin}}} $ for d underestimates the data and$ {\rm{Theo}}_{{\rm{fin}}} $ values for t and 3He overestimate the data; our results including only nucleon coalescence$ {\rm{Theo}}_{pn} $ ,$ {\rm{Theo}}_{nnp} $ , and$ {\rm{Theo}}_{ppn} $ can describe the corresponding data considerably better. This further indicates that nucleon coalescence is the dominant production for light nuclei in peripheral 40%−80% collisions, and other coalescence channels involving nucleon+nucleus and nucleus+nucleus may not occur.Centrality Rapidity d Data $ {\rm{Theo}}_{pn} $ 

$ {\rm{Theo}}_{{\rm{dep}}} $ 

$ {\rm{Theo}}_{{\rm{fin}}} $ 

0−10% $ -0.1<y<0 $ 

$ 16.21\pm 2.27 $ 

18.18 1.52 16.66 $ -0.2<y<-0.1 $ 

$ 16.19\pm 1.43 $ 

17.21 1.45 15.76 $ -0.3<y<-0.2 $ 

$ 15.27\pm 1.13 $ 

15.61 1.33 14.28 $ -0.4<y<-0.3 $ 

$ 14.76\pm 1.19 $ 

15.93 1.40 14.53 $ -0.5<y<-0.4 $ 

$ 14.14\pm 1.00 $ 

15.52 1.39 14.13 10%−20% $ -0.1<y<0 $ 

$ 9.39\pm 1.03 $ 

10.35 0.80 9.55 $ -0.2<y<-0.1 $ 

$ 9.64\pm 1.11 $ 

10.34 0.85 9.49 $ -0.3<y<-0.2 $ 

$ 9.66\pm 0.73 $ 

9.98 0.84 9.14 $ -0.4<y<-0.3 $ 

$ 9.82\pm 0.76 $ 

10.35 0.90 9.45 $ -0.5<y<-0.4 $ 

$ 10.53\pm 0.65 $ 

10.91 1.02 9.89 20%−40% $ -0.1<y<0 $ 

$ 4.89\pm 0.31 $ 

4.77 0.33 4.44 $ -0.2<y<-0.1 $ 

$ 5.10\pm 0.46 $ 

4.89 0.36 4.53 $ -0.3<y<-0.2 $ 

$ 5.18\pm 0.32 $ 

4.98 0.39 4.59 $ -0.4<y<-0.3 $ 

$ 5.29\pm 0.50 $ 

5.32 0.44 4.88 $ -0.5<y<-0.4 $ 

$ 6.09\pm 0.42 $ 

6.18 0.58 5.60 40%−80% $ -0.1<y<0 $ 

$ 0.97\pm 0.06 $ 

0.91 0.06 0.85 $ -0.2<y<-0.1 $ 

$ 1.00\pm 0.05 $ 

0.93 0.06 0.87 $ -0.3<y<-0.2 $ 

$ 1.07\pm 0.10 $ 

0.96 0.07 0.89 $ -0.4<y<-0.3 $ 

$ 1.26\pm 0.07 $ 

1.10 0.09 1.01 $ -0.5<y<-0.4 $ 

$ 1.59\pm 0.12 $ 

1.40 0.13 1.27 Table 2. Yield rapidity densities
${\rm d}N/{\rm d}y$ of d at different rapidity intervals and centralities in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV. The data are from Ref. [55], and the errors denote systematic uncertainties.Centrality Rapidity t 3He Data $ {\rm{Theo}}_{nnp} $ 

$ {\rm{Theo}}_{nd} $ 

$ {\rm{Theo}}_{{\rm{dep}}} $ 

$ {\rm{Theo}}_{{\rm{fin}}} $ 

Data $ {\rm{Theo}}_{ppn} $ 

$ {\rm{Theo}}_{pd} $ 

$ {\rm{Theo}}_{{\rm{dep}}} $ 

$ {\rm{Theo}}_{{\rm{fin}}} $ 

0−10% $ -0.1<y<0 $ 

$ 2.091\pm 0.219 $ 

1.263 0.860 0.045 2.078 $ 1.436\pm 0.125 $ 

0.860 0.605 0.041 1.424 $ -0.2<y<-0.1 $ 

$ 1.974\pm 0.184 $ 

1.215 0.820 0.043 1.992 $ 1.347\pm 0.122 $ 

0.824 0.576 0.040 1.360 $ -0.3<y<-0.2 $ 

$ 1.754\pm 0.138 $ 

1.102 0.729 0.041 1.790 $ 1.321\pm 0.097 $ 

0.803 0.552 0.038 1.317 $ -0.4<y<-0.3 $ 

$ 1.852\pm 0.125 $ 

1.191 0.787 0.044 1.934 $ 1.270\pm 0.078 $ 

0.814 0.559 0.041 1.332 $ -0.5<y<-0.4 $ 

$ 1.893\pm 0.115 $ 

1.187 0.784 0.045 1.926 $ 1.241\pm 0.070 $ 

0.805 0.552 0.041 1.316 10%−20% $ -0.1<y<0 $ 

$ 1.145\pm 0.214 $ 

0.700 0.455 0.023 1.132 $ 0.805\pm 0.072 $ 

0.471 0.319 0.021 0.769 $ -0.2<y<-0.1 $ 

$ 1.211\pm 0.154 $ 

0.757 0.481 0.026 1.212 $ 0.810\pm 0.044 $ 

0.506 0.336 0.024 0.818 $ -0.3<y<-0.2 $ 

$ 1.179\pm 0.138 $ 

0.742 0.467 0.028 1.181 $ 0.843\pm 0.064 $ 

0.521 0.343 0.024 0.840 $ -0.4<y<-0.3 $ 

$ 1.252\pm 0.088 $ 

0.805 0.506 0.030 1.281 $ 0.868\pm 0.051 $ 

0.548 0.360 0.027 0.881 $ -0.5<y<-0.4 $ 

$ 1.441\pm 0.088 $ 

0.922 0.574 0.036 1.460 $ 0.960\pm 0.066 $ 

0.611 0.399 0.032 0.978 20%−40% $ -0.1<y<0 $ 

$ 0.486\pm 0.060 $ 

0.312 0.188 0.009 0.491 $ 0.319\pm 0.033 $ 

0.202 0.129 0.008 0.323 $ -0.2<y<-0.1 $ 

$ 0.522\pm 0.056 $ 

0.355 0.207 0.011 0.551 $ 0.348\pm 0.043 $ 

0.234 0.145 0.010 0.369 $ -0.3<y<-0.2 $ 

$ 0.541\pm 0.071 $ 

0.383 0.220 0.013 0.590 $ 0.387\pm 0.045 $ 

0.263 0.161 0.012 0.412 $ -0.4<y<-0.3 $ 

$ 0.644\pm 0.044 $ 

0.436 0.249 0.016 0.669 $ 0.440\pm 0.037 $ 

0.292 0.178 0.014 0.456 $ -0.5<y<-0.4 $ 

$ 0.806\pm 0.078 $ 

0.568 0.323 0.023 0.868 $ 0.555\pm 0.036 $ 

0.380 0.231 0.020 0.591 40%−80% $ -0.1<y<0 $ 

$ 0.075\pm 0.015 $ 

0.076 0.032 0.002 0.106 $ 0.048\pm 0.007 $ 

0.049 0.023 0.002 0.070 $ -0.2<y<-0.1 $ 

$ 0.087\pm 0.014 $ 

0.087 0.035 0.003 0.119 $ 0.056\pm 0.007 $ 

0.056 0.026 0.002 0.080 $ -0.3<y<-0.2 $ 

$ 0.087\pm 0.007 $ 

0.090 0.036 0.003 0.123 $ 0.065\pm 0.008 $ 

0.065 0.030 0.002 0.093 $ -0.4<y<-0.3 $ 

$ 0.115\pm 0.007 $ 

0.119 0.046 0.005 0.160 $ 0.079\pm 0.005 $ 

0.082 0.037 0.004 0.115 $ -0.5<y<-0.4 $ 

$ 0.172\pm 0.016 $ 

0.179 0.069 0.008 0.240 $ 0.120\pm 0.010 $ 

0.124 0.055 0.006 0.173 Table 3. Yield rapidity densities
${\rm d}N/{\rm d}y$ of t and 3He at different rapidity intervals and centralities in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV. The data are from Ref. [55], and the errors denote systematic uncertainties.Table 4 shows the results of 4He at different rapidity intervals and centralities in Au-Au collisions at
$ \sqrt{s_{NN}}=3 $ GeV. The data with errors are from Ref. [55], and the errors denote systematic uncertainties.$ {\rm{Theo}}_{ppnn} $ ,$ {\rm{Theo}}_{pnd} $ ,$ {\rm{Theo}}_{pt} $ ,$ {\rm{Theo}}_{n^3{\rm{He}}} $ , and$ {\rm{Theo}}_{dd} $ in the fifth, sixth, seventh, eighth, and ninth columns denote the results of$ p+p+n+n $ ,$ p+n+d $ ,$ p+t $ ,$ n+{}^{3} {\rm{He}}$ , and$ d+d $ coalescing into 4He, respectively.$ {\rm{Theo}}_{{\rm{total}}} $ in the fourth column denotes the total results including all five coalescence sources for 4He.$ {\rm{Theo}}_{{\rm{total}}} $ in the 0−10%, 10%−20%, and 20%−40% centralities and$ {\rm{Theo}}_{ppnn} $ in the peripheral 40%−80% centrality underestimate the central values of the experimental data by approximately 20%. This may be because we do not consider decay contributions from the excited states of 4He. If the decay properties of these excited states become clear and these contributions are included in the future, the theoretical results will better match the data. We employ the averaged deviation degree$\delta_{\rm devi}$ to quantitatively characterize the extent of deviation of our theoretical results from the data, which is defined asCentrality Rapidity Data $ {\rm{Theo}}_{{\rm{total}}} $ 

$ {\rm{Theo}}_{ppnn} $ 

$ {\rm{Theo}}_{pnd} $ 

$ {\rm{Theo}}_{pt} $ 

$ {\rm{Theo}}_{n^3{\rm{He}}} $ 

$ {\rm{Theo}}_{dd} $ 

$\delta_{\rm devi}$ 

0−10% $ -0.1<y<0 $ 

$ 0.2187\pm 0.0207 $ 

0.1599 0.0345 0.0206 0.0447 0.0414 0.0187 7.8% $ -0.2<y<-0.1 $ 

$ 0.1943\pm 0.0162 $ 

0.1546 0.0337 0.0199 0.0432 0.0399 0.0179 6.3% $ -0.3<y<-0.2 $ 

$ 0.1842\pm 0.0196 $ 

0.1481 0.0329 0.0190 0.0414 0.0380 0.0168 7.1% $ -0.4<y<-0.3 $ 

$ 0.1778\pm 0.0102 $ 

0.1587 0.0353 0.0204 0.0444 0.0407 0.0179 5.4% $ -0.5<y<-0.4 $ 

$ 0.1767\pm 0.0106 $ 

0.1603 0.0357 0.0206 0.0448 0.0411 0.0181 4.3% 10%−20% $ -0.1<y<0 $ 

$ 0.1023\pm 0.0179 $ 

0.0824 0.0186 0.0106 0.0231 0.0210 0.0091 6.7% $ -0.2<y<-0.1 $ 

$ 0.1179\pm 0.0157 $ 

0.0943 0.0218 0.0121 0.0264 0.0239 0.0101 5.7% $ -0.3<y<-0.2 $ 

$ 0.1229\pm 0.0114 $ 

0.0977 0.0228 0.0125 0.0274 0.0247 0.0103 6.6% $ -0.4<y<-0.3 $ 

$ 0.1313\pm 0.0083 $ 

0.1072 0.0251 0.0137 0.0300 0.0271 0.0113 6.5% $ -0.5<y<-0.4 $ 

$ 0.1582\pm 0.0087 $ 

0.1288 0.0304 0.0165 0.0361 0.0324 0.0134 7.0% 20%−40% $ -0.1<y<0 $ 

$ 0.0435\pm 0.0025 $ 

0.0325 0.0079 0.0042 0.0091 0.0081 0.0032 9.2% $ -0.2<y<-0.1 $ 

$ 0.0447\pm 0.0037 $ 

0.0407 0.0102 0.0052 0.0114 0.0100 0.0039 7.9% $ -0.3<y<-0.2 $ 

$ 0.0548\pm 0.0031 $ 

0.0478 0.0122 0.0061 0.0134 0.0117 0.0044 9.8% $ -0.4<y<-0.3 $ 

$ 0.0620\pm 0.0054 $ 

0.0564 0.0145 0.0072 0.0158 0.0137 0.0052 6.1% $ -0.5<y<-0.4 $ 

$ 0.0898\pm 0.0050 $ 

0.0822 0.0211 0.0105 0.0230 0.0200 0.0076 7.7% 40%−80% $ -0.1<y<0 $ 

$ 0.0033\pm 0.0002 $ 

0.0085 0.0029 0.0011 0.0023 0.0017 0.0005 64.3% (6.2%) $ -0.2<y<-0.1 $ 

$ 0.0045\pm 0.0008 $ 

0.0105 0.0037 0.0013 0.0028 0.0021 0.0006 57.0% (6.0%) $ -0.3<y<-0.2 $ 

$ 0.0058\pm 0.0003 $ 

0.0123 0.0044 0.0015 0.0033 0.0025 0.0006 53.6% (10.0%) $ -0.4<y<-0.3 $ 

$ 0.0090\pm 0.0005 $ 

0.0176 0.0064 0.0022 0.0046 0.0035 0.0009 49.9% (12.2%) $ -0.5<y<-0.4 $ 

$ 0.0142\pm 0.0008 $ 

0.0310 0.0114 0.0038 0.0082 0.0061 0.0015 55.5% (9.7%) Table 4. Yield rapidity densities
${\rm d}N/{\rm d}y$ of 4He at different rapidity intervals and centralities in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV. The data are from Ref. [55], and the errors denote systematic uncertainties. The last column contains the averaged deviation degree$\delta_{\rm devi}$ of d, t, 3He, and 4He.$ \delta_{\rm devi} = \frac{1}{4} \sum\limits_{j=d, t, ^3{\rm{He}}, ^4{\rm{He}}} \left| \frac{{\rm{Theory}}_{j}-{\rm{Data}}_{j}}{{\rm{Data}}_{j}} \right| . $

(43) The values of
$ \delta_{devi} $ calculated with$ {\rm{Theo}}_{{\rm{fin}}} $ for d, t, and 3He and$ {\rm{Theo}}_{{\rm{total}}} $ for 4He are shown in the last column in Table 4, and those in the parentheses for the 40%−80% centrality are calculated with the results only including nucleon coalescence.Our theoretical results in Tables 2, 3 , and 4 clearly show the contribution proportions of different production sources for d, t, 3He, and 4He in their production in the 0−10%, 10%−20%, and 20%−40% centralities. The proportions of nucleon coalescence and nucleon
$ +d $ coalescence in t and 3He production are approximately 60% and 40%, respectively. The proportions of nucleon,$ p+n+d $ ,$ p+t $ ,$ n+{}^{3} {\rm{He}}$ , and$ d+d $ coalescence in 4He production are approximately 20%, 15%, 30%, 25%, and 10%, respectively. Tables 2 and 3 also show that the depletion of d is approximately 7%−9%, whereas the depletions of t and 3He are both less than 3%. These results reveal that besides nucleon coalescence, other particle coalescences, e.g., composite particles of lower mass numbers coalescing into light nuclei of larger mass numbers or composite particles capturing nucleons to recombine into heavier light nuclei, also play important roles in light nucleus production in central and semi-central collisions at relatively low collision energies. This provides a new possible window to investigate the underestimations of the yield densities of light nuclei in specific models including only nucleon coalescence, such as in Ref. [70]. -
The yield ratios of light nuclei reveal their production correlations and production mechanisms. Figure 6 shows the ratios of light nuclei to protons, that is,
$ d/p $ ,$ t/p $ ,$ d/p^2 $ , and$ t/p^3 $ . The filled circles and squares with error bars denote the experimental data in the rapidity bins$ -0.1<y<0 $ and$ -0.4<y<-0.3 $ , respectively [55]. The open circles and squares, connected with different lines to guide the eye, are the corresponding theoretical results, which include nucleon and nucleon$ +d $ coalescence for the 0−10%, 10%−20%, and 20%−40% centralities but only include nucleon coalescence for 40%−80% centrality. The theoretical uncertainties for the open symbols are from the uncertainties of$ R_f $ and$ Z_{np} $ as well as the blast-wave fitting errors for protons. The open symbols are shifted by 1% in the centrality axis for clarity. Figure 6 (a) and (b) show that both$ d/p $ and$ t/p $ decrease from central to peripheral collisions and increase slightly from$ -0.1<y<0 $ to$ -0.4<y<-0.3 $ . In the coalescence framework,$ d/p $ and$ t/p $ are mainly related to two elements: the nucleon volume density and the stronger suppression effect of light nucleus production in smaller reaction systems [69]. Such decreasing behaviors of$ d/p $ and$ t/p $ from central to peripheral collisions are dominated by the latter. This is similar to that from Pb-Pb to pp collisions at the LHC [69]. The slight increasing behavior from$ -0.1<y<0 $ to$ -0.4<y<-0.3 $ is due to the increasing nucleon volume density, which can be evaluated with$ R_f $ in Table 1 and the primordial protons measured in Ref. [55]. Figure 6 (c) and (d) show that$ d/p^2 $ and$ t/p^3 $ increase from central to peripheral collisions. They also increase but very slightly from$ -0.1<y<0 $ to$ -0.4<y< -0.3 $ . This is because$ d/p^2 $ denotes the probability for a nucleon pair coalescing into d, and$ t/p^3 $ denotes that for three nucleons coalescing into t. They are inversely proportional to the volume. Therefore, with decreasing$ R_f $ , they increase from central to peripheral collisions and also increase from$ -0.1<y<0 $ to$ -0.4<y<-0.3 $ . The coalescence model can nicely explain the centrality- and rapidity-dependent characteristics of$ d/p $ ,$ t/p $ ,$ d/p^2 $ , and$ t/p^3 $ .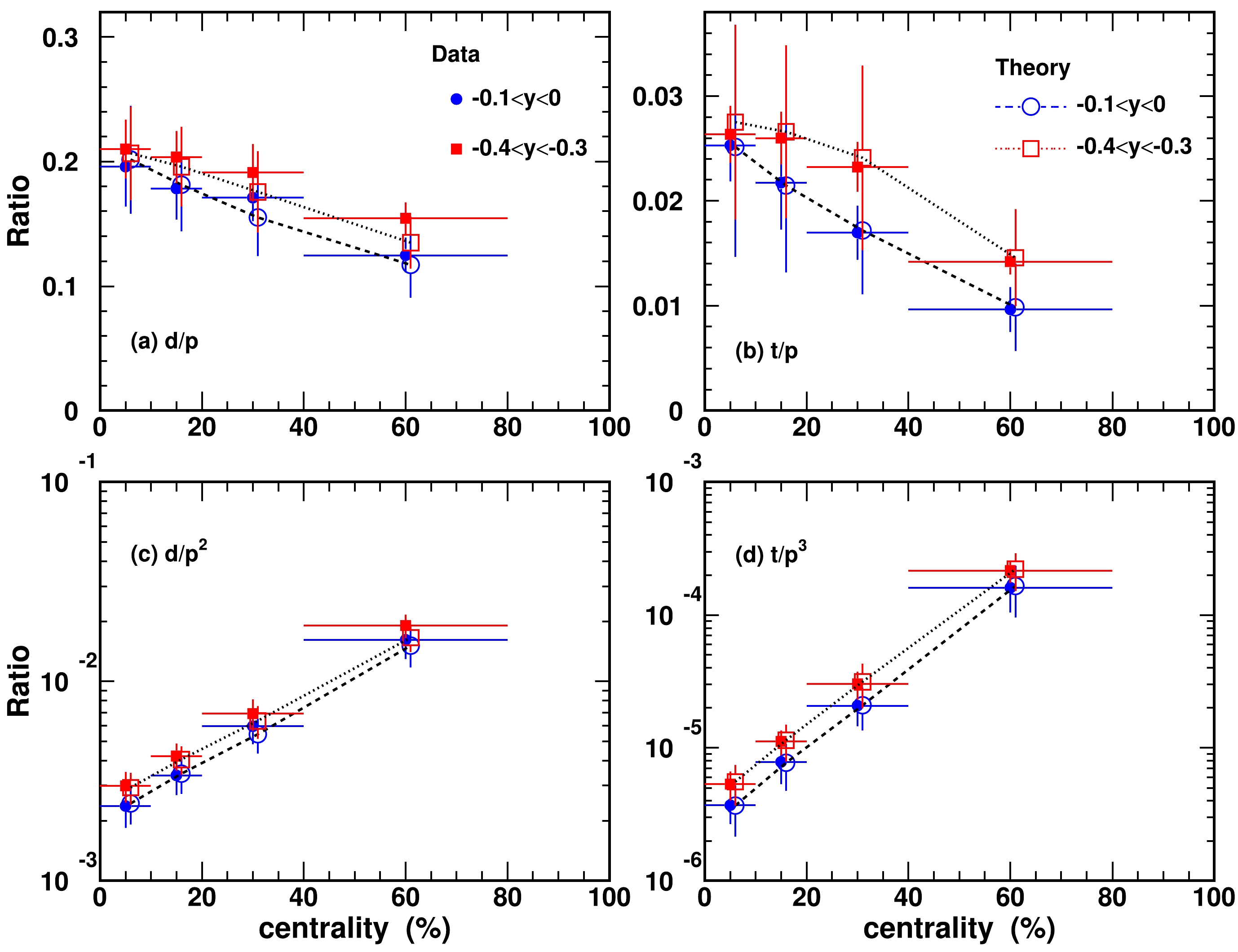
Figure 6. (color online) Ratios (a)
$ d/p $ , (b)$ t/p $ , (c)$ d/p^2 $ , and (d)$ t/p^3 $ as functions of the centrality in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV. The filled symbols are experimental data [55]. The open symbols, connected with different lines to guide the eye, are the theoretical results, which are shifted by 1% in the centrality axis for clarity.Figure 7 shows the ratio of pure light nuclei
$ t/d $ and the compound ratio$ tp/d^2 $ . The filled circles and squares with error bars denote the experimental data in the rapidity bins$ -0.1<y<0 $ and$ -0.4<y<-0.3 $ , respectively [55]. The open circles and squares, connected with different lines to guide the eye, are the corresponding theoretical results, which include nucleon and nucleon$ +d $ coalescence for the 0−10%, 10%−20%, and 20%−40% centralities but only include nucleon coalescence for the 40%−80% centrality. The open stars are the theoretical results of the 40%−80% centrality including both$ n+n+p $ and$ n+d $ coalescence for comparison. The theoretical uncertainties for the open symbols are from the uncertainties of$ R_f $ and$ Z_{np} $ as well as the blast-wave fitting errors for protons. The open circles and squares are shifted by 1% in the centrality axis for clarity. As shown in Fig. 7 , the current data of$ t/d $ and$ tp/d^2 $ remove$ n+d $ coalescence for t production in the peripheral 40%−80% centrality.$ t/d $ exhibits a decreasing trend from central to peripheral collisions, which is similar to that of$ d/p $ . The theoretical results of$ tp/d^2 $ increase slightly from central to peripheral collisions owing to the decreasing volume, which is consistent with those in Ref. [71]. Our theoretical results agree with the current data, except for the slight overestimation of$ tp/d^2 $ for$ -0.4<y<-0.3 $ in the 40%−80% centrality. This may suggest that besides the coalescence mechanism, other production contributions for light nuclei, such as fragmentation from spectators, become necessary in forward rapidity regions in peripheral collisions.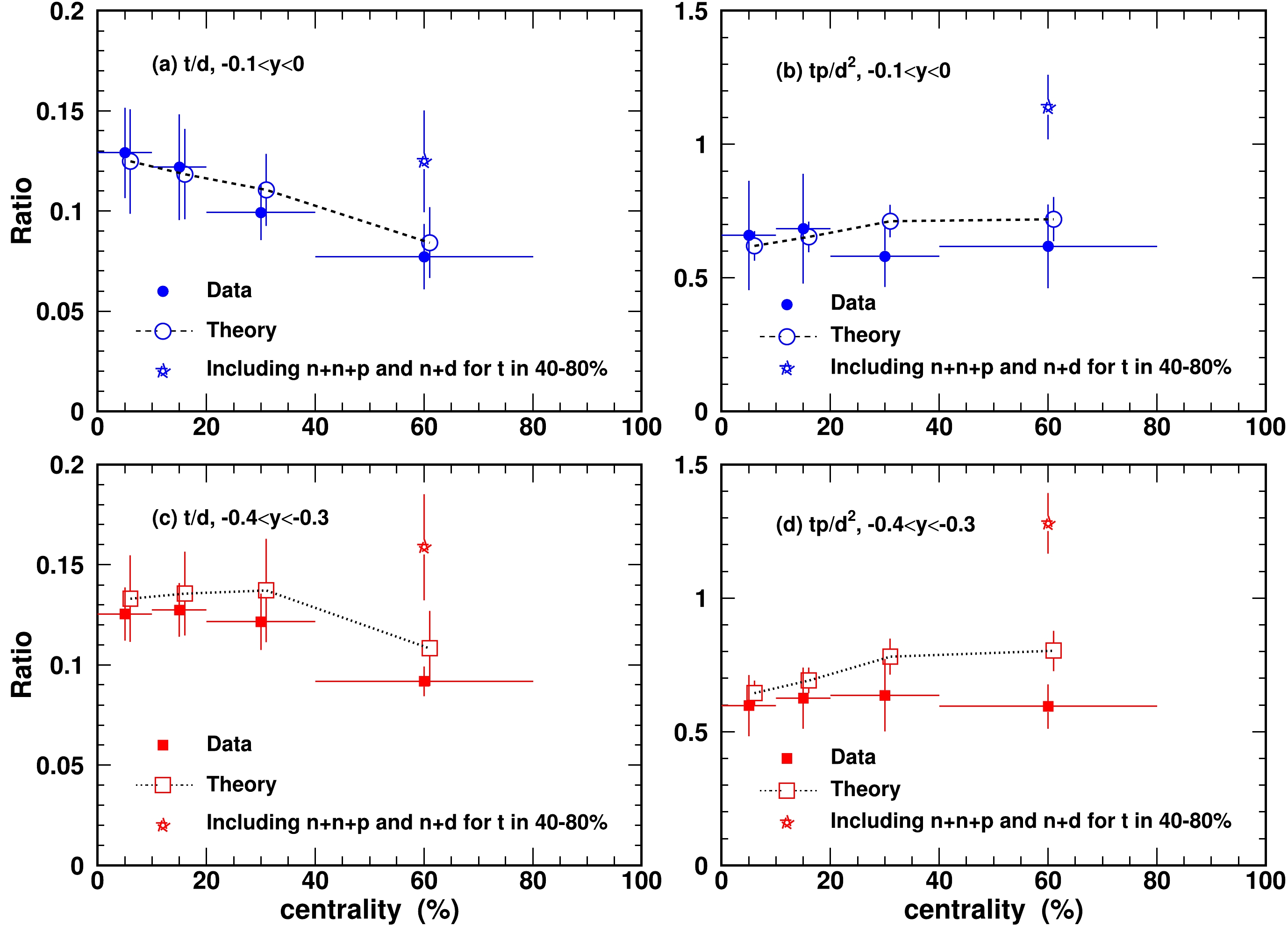
Figure 7. (color online) Ratios
$ t/d $ and$ tp/d^2 $ as functions of centrality in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV. The filled symbols are experimental data [55]. The open symbols, connected with different lines to guide the eye, are the theoretical results, which are shifted by 1% in the centrality axis for clarity. The open stars are the theoretical results of the 40%−80% centrality including both nucleon coalescence and nucleon$ +d $ coalescence for comparison. -
The averaged transverse momenta of different light nuclei reflect the collective motion and bulk properties of hadronic matter at kinetic freeze-out. In this subsection, we study the averaged transverse momenta
$ \langle p_T \rangle $ of d, t, 3He, and 4He at rapidity intervals of$ -0.1<y<0 $ ,$ -0.2<y<-0.1 $ ,$ -0.3<y<-0.2 $ ,$ -0.4<y<-0.3 $ , and$ -0.5<y< -0.4 $ in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV in the centralities 0−10%, 10%−20%, 20%−40%, and 40%−80%. Tables 5 and 6 show the results. The data with errors are from Ref. [55], and the errors denote systematic uncertainties. The$ \langle p_T \rangle_{{\rm{fin}}} $ values in the fourth, sixth, and tenth columns in Table 5 denote our theoretical results for final-state d, t, and 3He, respectively, and$ \langle p_T \rangle_{{\rm{total}}} $ in the fourth column in Table 6 denotes the total results including all five coalescence sources for 4He.$ \langle p_T \rangle_{nnp} $ and$ \langle p_T \rangle_{nd} $ in the seventh and eighth columns in Table 5 denote the results of$ n+n+p $ coalescing into t and$ n+d $ coalescing into t, respectively.$ \langle p_T \rangle_{ppn} $ and$ \langle p_T \rangle_{pd} $ in the eleventh and twelfth columns in Table 5 denote the results of$ p+p+n $ coalescing into 3He and$ p+d $ coalescing into 3He, respectively.$ \langle p_T \rangle_{ppnn} $ ,$ \langle p_T \rangle_{pnd} $ ,$ \langle p_T \rangle_{pt} $ ,$ \langle p_T \rangle_{n^3{\rm{He}}} $ , and$ \langle p_T \rangle_{dd} $ in the fifth, sixth, seventh, eighth, and ninth columns in Table 6 denote the results of$ p+p+n+n $ ,$ p+n+d $ ,$ p+t $ ,$ n+{}^{3} {\rm{He}}$ , and$ d+d $ coalescing into 4He, respectively. Tables 5 and 6 show that for t, 3He, and 4He, the calculated$ \langle p_T \rangle $ values from different coalescence sources are almost the same. This is very different from${\rm d}N/{\rm d}y$ . The theoretical uncertainty for$ \langle p_T \rangle $ is mainly due to the blast-wave fitting uncertainty for the proton$ p_T $ distribution and is 3%$ - $ 7%. The central values of our theoretical results agree with the data with deviations less than 10%.Centrality Rapidity d t 3He Data $ \langle p_T\rangle_{{\rm{fin}}} $ 

Data $ \langle p_T\rangle_{{\rm{fin}}} $ 

$ \langle p_T\rangle_{nnp} $ 

$ \langle p_T\rangle_{nd} $ 

Data $ \langle p_T\rangle_{{\rm{fin}}} $ 

$ \langle p_T\rangle_{ppn} $ 

$ \langle p_T\rangle_{pd} $ 

0−10% $ -0.1<y<0 $ 

$ 1.048\pm 0.033 $ 

1.033 $ 1.363\pm 0.044 $ 

1.343 1.347 1.337 $ 1.412\pm 0.044 $ 

1.340 1.343 1.335 $ -0.2<y<-0.1 $ 

$ 1.049\pm 0.032 $ 

1.028 $ 1.350\pm 0.047 $ 

1.338 1.342 1.332 $ 1.405\pm 0.041 $ 

1.335 1.338 1.330 $ -0.3<y<-0.2 $ 

$ 1.036\pm 0.026 $ 

1.014 $ 1.320\pm 0.037 $ 

1.317 1.321 1.311 $ 1.384\pm 0.045 $ 

1.314 1.318 1.309 $ -0.4<y<-0.3 $ 

$ 1.019\pm 0.031 $ 

1.004 $ 1.291\pm 0.030 $ 

1.308 1.312 1.302 $ 1.358\pm 0.034 $ 

1.305 1.308 1.300 $ -0.5<y<-0.4 $ 

$ 0.987\pm 0.024 $ 

0.976 $ 1.242\pm 0.020 $ 

1.274 1.277 1.268 $ 1.308\pm 0.024 $ 

1.271 1.274 1.266 10%−20% $ -0.1<y<0 $ 

$ 0.996\pm 0.042 $ 

0.965 $ 1.256\pm 0.043 $ 

1.242 1.246 1.236 $ 1.306\pm 0.052 $ 

1.239 1.243 1.234 $ -0.2<y<-0.1 $ 

$ 0.992\pm 0.045 $ 

0.961 $ 1.239\pm 0.035 $ 

1.238 1.241 1.232 $ 1.297\pm 0.039 $ 

1.235 1.238 1.230 $ -0.3<y<-0.2 $ 

$ 0.974\pm 0.024 $ 

0.941 $ 1.217\pm 0.044 $ 

1.209 1.212 1.203 $ 1.275\pm 0.030 $ 

1.206 1.209 1.201 $ -0.4<y<-0.3 $ 

$ 0.956\pm 0.025 $ 

0.935 $ 1.200\pm 0.030 $ 

1.204 1.208 1.199 $ 1.261\pm 0.025 $ 

1.201 1.205 1.197 $ -0.5<y<-0.4 $ 

$ 0.924\pm 0.032 $ 

0.919 $ 1.164\pm 0.014 $ 

1.188 1.191 1.182 $ 1.217\pm 0.032 $ 

1.185 1.188 1.180 20%−40% $ -0.1<y<0 $ 

$ 0.908\pm 0.034 $ 

0.893 $ 1.136\pm 0.053 $ 

1.137 1.140 1.131 $ 1.183\pm 0.050 $ 

1.134 1.137 1.129 $ -0.2<y<-0.1 $ 

$ 0.898\pm 0.042 $ 

0.887 $ 1.122\pm 0.043 $ 

1.128 1.132 1.122 $ 1.171\pm 0.053 $ 

1.125 1.128 1.120 $ -0.3<y<-0.2 $ 

$ 0.880\pm 0.034 $ 

0.871 $ 1.093\pm 0.051 $ 

1.108 1.111 1.102 $ 1.153\pm 0.057 $ 

1.105 1.108 1.101 $ -0.4<y<-0.3 $ 

$ 0.869\pm 0.031 $ 

0.863 $ 1.067\pm 0.027 $ 

1.097 1.100 1.091 $ 1.115\pm 0.021 $ 

1.094 1.097 1.089 $ -0.5<y<-0.4 $ 

$ 0.833\pm 0.019 $ 

0.834 $ 1.039\pm 0.041 $ 

1.061 1.064 1.056 $ 1.063\pm 0.020 $ 

1.059 1.061 1.054 40%−80% $ -0.1<y<0 $ 

$ 0.779\pm 0.023 $ 

0.779 $ 0.925\pm 0.019 $ 

0.971 0.973 0.965 $ 0.978\pm 0.046 $ 

0.968 0.970 0.963 $ -0.2<y<-0.1 $ 

$ 0.774\pm 0.001 $ 

0.767 $ 0.911\pm 0.028 $ 

0.954 0.956 0.948 $ 0.947\pm 0.026 $ 

0.951 0.953 0.947 $ -0.3<y<-0.2 $ 

$ 0.761\pm 0.023 $ 

0.747 $ 0.900\pm 0.011 $ 

0.925 0.927 0.920 $ 0.931\pm 0.032 $ 

0.923 0.925 0.919 $ -0.4<y<-0.3 $ 

$ 0.736\pm 0.001 $ 

0.739 $ 0.878\pm 0.011 $ 

0.915 0.917 0.910 $ 0.899\pm 0.004 $ 

0.912 0.914 0.908 $ -0.5<y<-0.4 $ 

$ 0.706\pm 0.013 $ 

0.718 $ 0.833\pm 0.009 $ 

0.894 0.895 0.889 $ 0.854\pm 0.025 $ 

0.891 0.893 0.888 Table 5. Averaged transverse momenta
$ \langle p_T \rangle $ of d, t, and 3He at different rapidity intervals and centralities in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV. The data are from Ref. [55], and the errors denote systematic uncertainties.Centrality Rapidity Data $ \langle p_T\rangle_{{\rm{total}}} $ 

$ \langle p_T\rangle_{ppnn} $ 

$ \langle p_T\rangle_{pnd} $ 

$ \langle p_T\rangle_{pt} $ 

$ \langle p_T\rangle_{n^3{\rm{He}}} $ 

$ \langle p_T\rangle_{dd} $ 

0−10% $ -0.1<y<0 $ 

$ 1.591\pm 0.048 $ 

1.620 1.630 1.619 1.620 1.617 1.608 $ -0.2<y<-0.1 $ 

$ 1.566\pm 0.041 $ 

1.615 1.625 1.614 1.615 1.612 1.602 $ -0.3<y<-0.2 $ 

$ 1.535\pm 0.046 $ 

1.588 1.598 1.587 1.588 1.585 1.575 $ -0.4<y<-0.3 $ 

$ 1.508\pm 0.022 $ 

1.581 1.591 1.580 1.581 1.578 1.569 $ -0.5<y<-0.4 $ 

$ 1.483\pm 0.003 $ 

1.541 1.550 1.540 1.541 1.539 1.530 10%−20% $ -0.1<y<0 $ 

$ 1.496\pm 0.081 $ 

1.487 1.496 1.486 1.486 1.484 1.475 $ -0.2<y<-0.1 $ 

$ 1.487\pm 0.080 $ 

1.481 1.491 1.480 1.481 1.478 1.469 $ -0.3<y<-0.2 $ 

$ 1.446\pm 0.046 $ 

1.444 1.453 1.443 1.444 1.441 1.432 $ -0.4<y<-0.3 $ 

$ 1.397\pm 0.020 $ 

1.441 1.451 1.440 1.441 1.439 1.430 $ -0.5<y<-0.4 $ 

$ 1.363\pm 0.006 $ 

1.426 1.435 1.425 1.426 1.423 1.415 20%−40% $ -0.1<y<0 $ 

$ 1.316\pm 0.036 $ 

1.348 1.357 1.347 1.348 1.345 1.337 $ -0.2<y<-0.1 $ 

$ 1.296\pm 0.024 $ 

1.337 1.346 1.335 1.336 1.334 1.325 $ -0.3<y<-0.2 $ 

$ 1.262\pm 0.006 $ 

1.313 1.322 1.312 1.313 1.310 1.301 $ -0.4<y<-0.3 $ 

$ 1.227\pm 0.058 $ 

1.299 1.308 1.298 1.299 1.296 1.288 $ -0.5<y<-0.4 $ 

$ 1.173\pm 0.038 $ 

1.258 1.266 1.257 1.258 1.255 1.248 40%−80% $ -0.1<y<0 $ 

$ 1.139\pm 0.048 $ 

1.134 1.141 1.132 1.132 1.129 1.122 $ -0.2<y<-0.1 $ 

$ 1.095\pm 0.043 $ 

1.112 1.118 1.109 1.110 1.107 1.100 $ -0.3<y<-0.2 $ 

$ 1.062\pm 0.005 $ 

1.076 1.082 1.074 1.075 1.072 1.066 $ -0.4<y<-0.3 $ 

$ 1.005\pm 0.026 $ 

1.064 1.070 1.062 1.062 1.060 1.054 $ -0.5<y<-0.4 $ 

$ 0.972\pm 0.072 $ 

1.043 1.048 1.041 1.041 1.039 1.033 Table 6. Averaged transverse momenta
$ \langle p_T \rangle $ of 4He at different rapidity intervals and centralities in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV. The data are from Ref. [55], and the errors denote systematic uncertainties.To decode the collective properties of light nuclei more intuitively, we plot
$ \langle p_T \rangle $ of d, t, 3He, and 4He as functions of the mass$ m_A $ from central to peripheral collisions at the rapidity intervals −0.1 < y < 0, −0.2 < y < −0.1, −0.3 < y < −0.2, −0.4 < y < −0.3, and −0.5 < y < −0.4 in Fig. 8 (a)−(e). The filled symbols are the experimental data [55], and the open symbols are the theoretical results of the coalescence model. For clarity, the mass of 3He is shifted by 0.2 GeV/c2. The proton$ \langle p_T \rangle $ is also plotted. Its data are taken from [55], and the theoretical results are from the blast-wave model of Eq. (41). The different lines are the fitting results from the linear function$ \langle p_T \rangle = k*m_A+p_0 $ , where$ p_0=0.35 $ GeV/c, and k is a slope parameter. Figure 8 shows that$ \langle p_T \rangle $ increases linearly as a function of$ m_A $ . This indicates that there is a mutual averaged velocity at freeze-out for protons and different light nuclei and further supports their collective motion.$ \langle p_T \rangle $ of p, d, t, 3He, and 4He decreases gradually from central to peripheral collisions, indicating stronger transverse collective motion in more central collisions.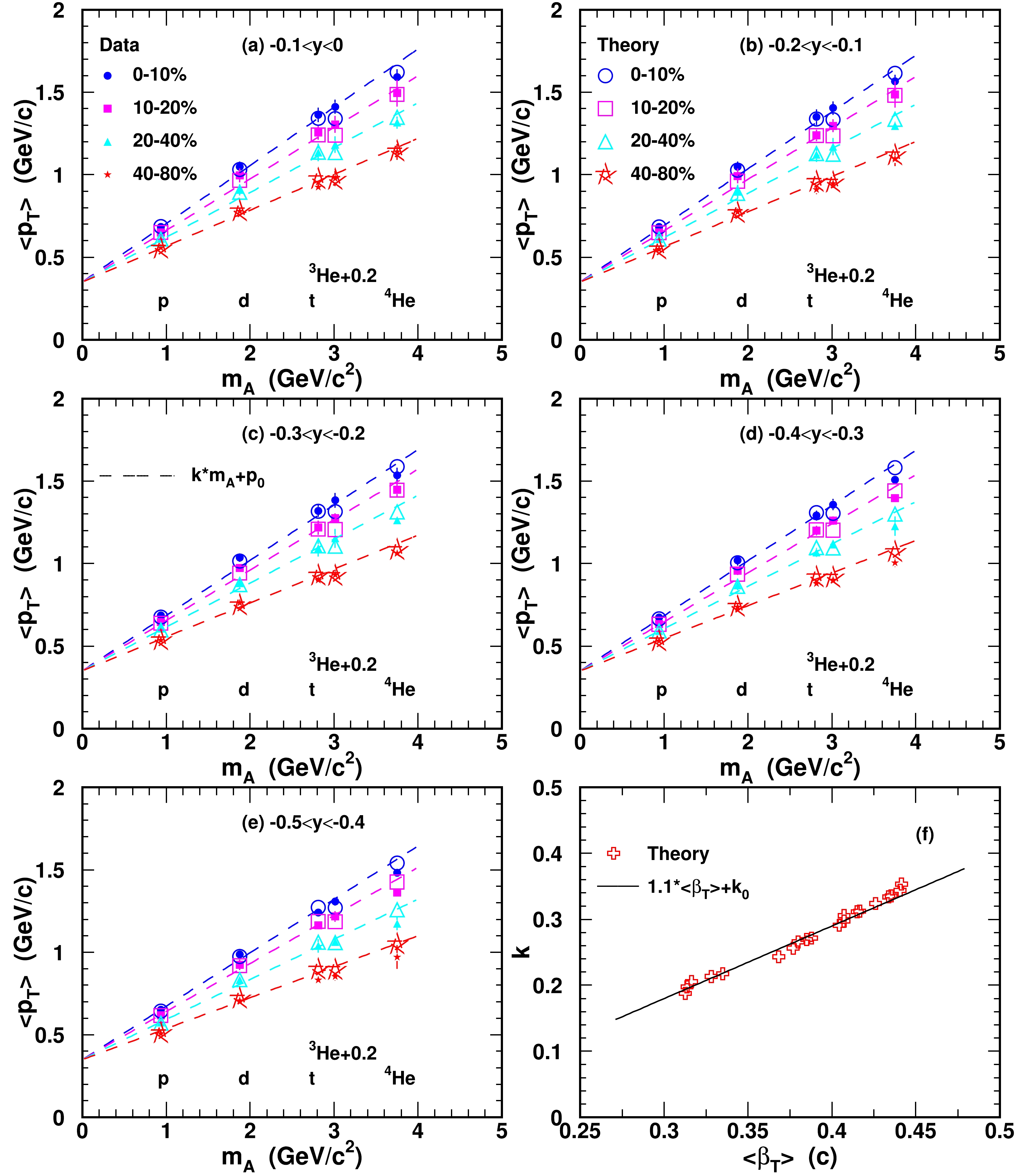
Figure 8. (color online) Averaged transverse momentum
$ \langle p_T \rangle $ as functions of the mass$ m_A $ in Au-Au collisions at$\sqrt{s_{NN}}=3 $ in different rapidity intervals: (a)$ -0.1<y<0 $ , (b)$ -0.2< $ $ y<-0.1 $ , (c)$ -0.3<y<-0.2 $ , (d)$ -0.4<y<-0.3 $ , (e)$ -0.5<y<-0.4 $ , (f) the slope parameter k as a function of$\langle \beta_T \rangle$ . The filled symbols are experimental data [55]. The open symbols are the theoretical results. Different lines are linearly fitting results.The slope parameter k is positively correlated with the collective velocity. If we apply the momentum-velocity relation of the single particle to the averaged case, we have
$ \langle p_T \rangle=m_A \langle \gamma_T \rangle \langle \beta_T \rangle+p_0 $ . Here,$ \langle \beta_T \rangle $ is the averaged transverse collective velocity, and$ \langle \gamma_T \rangle $ is the corresponding Lorentz contraction factor.$ p_0 $ denotes the contribution from the thermal fluctuations. Therefore, we approximately have$ k=\langle \beta_T \rangle/\sqrt{1-\langle \beta_T \rangle^2} $ . This can naturally explain that the larger values of k in more central collisions are due to the stronger collective velocity. We plot k as a function of the averaged transverse velocity of protons obtained via Eq. (41) in Fig. 8 (f) with cross symbols. A linear relationship between k and$ \langle \beta_T \rangle $ is observed because the values of$ \langle \beta_T \rangle $ are in the range 0.31$ \sim $ 0.44, which makes$ 1/\sqrt{1-\langle \beta_T \rangle^2} $ nearly constant, at approximately 1.1. The solid line in Fig. 8 (f) is the fitting result of the function$ 1.1*\langle \beta_T \rangle+k_0 $ .Note that the coalescence model can effectively describe the production properties of various species of light nuclei measured in the midrapidity area in Au-Au collisions at
$ \sqrt{s_{NN}}=3 $ GeV. Compared to those at high RHIC and LHC energies observed in our previous studies [51, 58], coalescence in relativistic heavy ion collisions at lower collision energies have several new characteristics in light nucleus production, e.g., isospin asymmetry from the colliding nuclei and non-negligible nucleus+nucleon/nucleus coalescence. The coalescence mechanism still dominates in the midrapidity area in Au-Au collisions at$ \sqrt{s_{NN}}=3 $ GeV. In the forward rapidity region, the latest data hint at some properties beyond coalescence, e.g., the monotonically decreasing trend of the p and d rapidity distributions accompanied by peak behaviors in the t, 3He, and 4He rapidity distributions [55]. This indicates the necessity of other production mechanisms, such as fragmentation production from heavier nuclei or nuclear fragments. -
We study the different coalescence sources of the production of various species of light nuclei in relativistic heavy ion collisions within the coalescence mechanism. We first extend the coalescence model to include two bodies, three bodies, and four nucleons coalescing into light nuclei and use the assumption of the coordinate-momentum factorization of joint hadronic distributions. We adopt Gaussian forms for the relative coordinate distributions. Based on these simplifications, we obtain analytic formulas of the momentum distributions of light nuclei formed from different production sources coalesced by different hadrons.
Subsequently, we apply the extended coalescence model to Au-Au collisions at
$ \sqrt{s_{NN}}=3 $ GeV to simultaneously investigate the$ p_T $ spectra of d, t, 3He, and 4He at different rapidity intervals in the midrapidity area from central to peripheral collisions. We present the$ p_T $ dependence of different coalescence sources for d, t, 3He, and 4He. We also study the yield rapidity densities$ {\rm d} N/{\rm d} y $ and averaged transverse momenta$ \langle p_T \rangle $ of d, t, 3He, and 4He and provide the proportions of yield densities from different coalescence sources for t, 3He, and 4He in their production and those of depletions for d, t, and 3He. The yield densities from different coalescence sources for a specific type of light nucleus are very different, but the averaged transverse momenta are almost unchanged.Our results indicate the following. (1) The results of
$ p+n $ coalescence minus those depleted in nucleus coalescence effectively reproduce the available data of d in central and semi-central collisions, and the data for peripheral collisions favor$ p+n $ coalescence. (2) The nucleon coalescence plus nucleon$ +d $ coalescence reproduces the available data of t and 3He in central and semi-central collisions (their depletions in forming 4He are less than 3%), and the data for peripheral collisions favor only nucleon coalescence. (3) The nucleon coalescence plus nucleon+nucleus coalescence and nucleus+nucleus coalescence describe the available data of 4He in central and semi-central collisions, and the data for peripheral collisions favor only$ p+p+n+n $ coalescence. -
We thank Prof. Xiao-Feng Luo for helpful discussions and the STAR collaboration for providing us with data for p, d, t, 3He, and 4He in Au-Au collisions at
$ \sqrt{s_{NN}}=3 $ GeV.
Different coalescence sources of light nucleus production in Au-Au collisions at ${ \sqrt{\boldsymbol s_{\boldsymbol N\boldsymbol N}}=\bf 3 }$ GeV
- Received Date: 2023-10-16
- Available Online: 2024-05-15
Abstract: We study the production of light nuclei in the coalescence mechanism of Au-Au collisions at midrapidity at







 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF















 DownLoad:
DownLoad: