1981 Vol. 5, No. 2
Display Method: |
Abstract:
A picture of the dibaryon system is proposed. If the fundamental particles of SU6 group are three baryons p, n and Λ which have spin 1/2, then the dibaryon system must be classified according to SU6 group. SU6 group connect the strange analogy state of spin 0 with strange analogy state of spin 1. The "original" mass difference between Λ hyperon and nucleon leads to the SU6 symmetry breaking. Then, SU3 gives the mass relations between strange analogy states of same spin, and SU6 gives mass relations between the different strange analogy states of spin 0 and 1.
A picture of the dibaryon system is proposed. If the fundamental particles of SU6 group are three baryons p, n and Λ which have spin 1/2, then the dibaryon system must be classified according to SU6 group. SU6 group connect the strange analogy state of spin 0 with strange analogy state of spin 1. The "original" mass difference between Λ hyperon and nucleon leads to the SU6 symmetry breaking. Then, SU3 gives the mass relations between strange analogy states of same spin, and SU6 gives mass relations between the different strange analogy states of spin 0 and 1.
Abstract:
Using coherent fluctuation nuclear model and Glauber's multiple scattering theory,the double charge exchange (DCE) reaction cross sections for π+ on 16O and on 16O are calculated. The results show that, owing to the paring effect, the probability of the double nucleon transition between the isospin non-analogue states increases remarkably: the calculated values of the ratio of a σ 18O (π+, π-) 18Neg.s. to σ (16O (π+,π-) 16Ne)g.s. fit the experimental data quite well. It also shows that, for fitting the experimental data, the magnitude of the 2p-2h configuration components exsited in 16O ground state would not be too large. The effect of the intermediate states in multiple stage processes is estimated, which shows that the contribution of these processes to the DCE reaction is rather small.
Using coherent fluctuation nuclear model and Glauber's multiple scattering theory,the double charge exchange (DCE) reaction cross sections for π+ on 16O and on 16O are calculated. The results show that, owing to the paring effect, the probability of the double nucleon transition between the isospin non-analogue states increases remarkably: the calculated values of the ratio of a σ 18O (π+, π-) 18Neg.s. to σ (16O (π+,π-) 16Ne)g.s. fit the experimental data quite well. It also shows that, for fitting the experimental data, the magnitude of the 2p-2h configuration components exsited in 16O ground state would not be too large. The effect of the intermediate states in multiple stage processes is estimated, which shows that the contribution of these processes to the DCE reaction is rather small.
Abstract:
Quantitative analysis of the space charge force compensation by means of RF quadrupoles in a synchrotron is given. For a bunched beam, if RF quadrupole magnets operating at the fundamental RF frequency (or at most the fundamental and the second harmonic frequency) are applied, the linear betatron turn shift Δv, excited by the linear part of space charge force, would be diminished effectively for the most particles, either in palabolic distribution at longitudinal direction or in Gaussian distribution. Then the space charge limit, mainly decided by nonlinear turn shift, would be raised twice as large as that given in Laslett formular.
Quantitative analysis of the space charge force compensation by means of RF quadrupoles in a synchrotron is given. For a bunched beam, if RF quadrupole magnets operating at the fundamental RF frequency (or at most the fundamental and the second harmonic frequency) are applied, the linear betatron turn shift Δv, excited by the linear part of space charge force, would be diminished effectively for the most particles, either in palabolic distribution at longitudinal direction or in Gaussian distribution. Then the space charge limit, mainly decided by nonlinear turn shift, would be raised twice as large as that given in Laslett formular.
Abstract:
The concept of quasi-Δ33 doorway state is put forward in the paper. Under this simple model, differential cross sections of elastic π--16O scattering is calculated at Tπlab=163, 220, 240, 254, 303, 380 Mev and compared with existing data, We arrive at a agreement with experimential data.
The concept of quasi-Δ33 doorway state is put forward in the paper. Under this simple model, differential cross sections of elastic π--16O scattering is calculated at Tπlab=163, 220, 240, 254, 303, 380 Mev and compared with existing data, We arrive at a agreement with experimential data.
Abstract:
It has been shown that the classical SU(3) Yang-Mills equation with a static and extended external source has static and spherically symmetric solutions only for spherically symmetric external source, and in that external source there is no further static and spherically symmetric solution besides the Coulombic one.
It has been shown that the classical SU(3) Yang-Mills equation with a static and extended external source has static and spherically symmetric solutions only for spherically symmetric external source, and in that external source there is no further static and spherically symmetric solution besides the Coulombic one.
Abstract:
Based on the "Rigid Projectile" Approximation, we have analyzed the Saclay data on the elastic scattering of alpha 12C. At the energy Talab=1.37 GeV. Using the Glauber's multiple scattering theory, we assume that 4He-12C scattering is a multiple scattering process between nucleons in projectile 4He and target nucleus 12C. We don't introduce any free parameter in our calculation. The theoretical differential cross sections are compared with the experimental one. The agreement is satisfactory. It supports strongly the multiple scattering mechanism we have assumed.
Based on the "Rigid Projectile" Approximation, we have analyzed the Saclay data on the elastic scattering of alpha 12C. At the energy Talab=1.37 GeV. Using the Glauber's multiple scattering theory, we assume that 4He-12C scattering is a multiple scattering process between nucleons in projectile 4He and target nucleus 12C. We don't introduce any free parameter in our calculation. The theoretical differential cross sections are compared with the experimental one. The agreement is satisfactory. It supports strongly the multiple scattering mechanism we have assumed.
Abstract:
The second kind ripple of a cascade is analysed under the second order approximation. A formula is derived for the computation. It is pointed out that the zero order approximation approach can be used satisfactorily only when C»n2Cs. The second kind ripple of a symmetric type cascade has also been analysed under zero order approximation. Another formula is given.
The second kind ripple of a cascade is analysed under the second order approximation. A formula is derived for the computation. It is pointed out that the zero order approximation approach can be used satisfactorily only when C»n2Cs. The second kind ripple of a symmetric type cascade has also been analysed under zero order approximation. Another formula is given.
Abstract:
The characteristics of high energy γ-rays are studued using emulsion chamber installed at Mt. Ganbala, Tibet, 5500 m high above the sea level, at atmospheric depth of 520g/cm2. The vertical flux, integral energy spectrum, zenith-angle distribution and the attenuation length in the atmosphere for the high energy γ-rays are obtained. The comparsion of the results with those of the similar works shows satisfactory agreement. Several γ-ray families are selected and analyzed and the energy spectrum, fractional energy spectrum and the mean values of the transverse momentum of γ-rays in the families are obtained and discussed.
The characteristics of high energy γ-rays are studued using emulsion chamber installed at Mt. Ganbala, Tibet, 5500 m high above the sea level, at atmospheric depth of 520g/cm2. The vertical flux, integral energy spectrum, zenith-angle distribution and the attenuation length in the atmosphere for the high energy γ-rays are obtained. The comparsion of the results with those of the similar works shows satisfactory agreement. Several γ-ray families are selected and analyzed and the energy spectrum, fractional energy spectrum and the mean values of the transverse momentum of γ-rays in the families are obtained and discussed.
Abstract:
In order to study the structure of the bound states of the p-p system, a Schrodinger equation of a complex square well with finite depth is solved exactly. We have calculated the variation of the widths Γ of the energy levels with the depth ξ and the range a1 of the virtual part of the potential and compared them with the perturbation results. We come to the conclusion that (ⅰ) the narrow widths of the bound states are obtained; (ⅱ) the perturbation method can not be used to calculate the widths of the levels for a complex square well when the depth of the virtual part of the potential is much larger than the real part; (ⅲ) to describe annihilation of p-p system by means of the absorption potential not only depends on ξ, the depth of the virtual part of the potential, but also on the range a1.
In order to study the structure of the bound states of the p-p system, a Schrodinger equation of a complex square well with finite depth is solved exactly. We have calculated the variation of the widths Γ of the energy levels with the depth ξ and the range a1 of the virtual part of the potential and compared them with the perturbation results. We come to the conclusion that (ⅰ) the narrow widths of the bound states are obtained; (ⅱ) the perturbation method can not be used to calculate the widths of the levels for a complex square well when the depth of the virtual part of the potential is much larger than the real part; (ⅲ) to describe annihilation of p-p system by means of the absorption potential not only depends on ξ, the depth of the virtual part of the potential, but also on the range a1.
Abstract:
Appling the formula on C-G series of SU3 group which was derived by the author, the action of Wigner operator of SU3 group is systematically discussed, the distribution rule of occupation number in C-G series of SU3 group is analysed, and the null space of Wigner operator of SU3 group is determined.
Appling the formula on C-G series of SU3 group which was derived by the author, the action of Wigner operator of SU3 group is systematically discussed, the distribution rule of occupation number in C-G series of SU3 group is analysed, and the null space of Wigner operator of SU3 group is determined.
Abstract:
Some expressions for the nuclear potentials have been obtained by means of nuclear charge dynamics. If point charge structure is assumed for nucleons, the NN interaction thus obtained appears in the same form as that of Cammel-Thaler potential, except that all the coefficients are now fixed and have physics meanings.Expressions obtained for nucleons of finite size will have no singularity at r=0. No cutoff is needed in the short range in using these potentials.The optical potential of the nucleus may be obtained in the same way. The nuclear potentials thus derived are short-ranged with diffused edge and have appromately correct magnitude and range.
Some expressions for the nuclear potentials have been obtained by means of nuclear charge dynamics. If point charge structure is assumed for nucleons, the NN interaction thus obtained appears in the same form as that of Cammel-Thaler potential, except that all the coefficients are now fixed and have physics meanings.Expressions obtained for nucleons of finite size will have no singularity at r=0. No cutoff is needed in the short range in using these potentials.The optical potential of the nucleus may be obtained in the same way. The nuclear potentials thus derived are short-ranged with diffused edge and have appromately correct magnitude and range.
Abstract:
The usual way to check whether a nuclear mass formula is good or not is to compare the calculated mass with experimental value. In this paper some concepts are summaried which check one or two terms in the mass formula separately: (ⅰ) P/P ratio-pairing energy; (ⅱ) Janecke ratio-symmetry energy; (ⅲ) A-dependence of giant resonance energy-symmetry energy; (ⅳ) IMME (isobaric multiplets mass equation)-Coulomb energy; (ⅴ) Difference of Coulomb energies-Coulomb energy; (ⅵ) β-stability lineCoulomb energy and symmetry energy; (ⅶ) Fissibility-Coulomb energy and surface energy. The following nuclear mass formulae are compared with each other: (A) Revised Weizsacker formula; (B) Danos-Gillet formula; (C) Myers-Swiatecki formula; (D) our formula. The main results are the following: (ⅰ) P/P'=1 for (A), (B) or (C);=3/4 for (D), which is in agreement with the experiment. (ⅱ) From the Janecke ratio, the form T(T+1) for the symmetry energy is better than T(T+4) or T2. (ⅲ) By the symmetry term T2/Aa, with a=0.90, the A-depeneence of giant resonance can be explained extremely well. (ⅳ) IMME, M(A, Tz)=a+bTz2+cTz2+dT z3. Only for (D), d≠0. (ⅴ) △Eo=Eo (Z+I)—Ec (Z), For (A) and (B), △EcA1/3/(Z+(1/2))=const.; for (C), △EcA1/3/(Z+(1/2)) (1—1.689)/A2/3=const.; for (D), △Ec Z2/3=const., which is in good agreement with the experiment. (ⅵ) For the β-stability line, if we compare the calculated ZA with the experimental value ZA and calculate the root mean square devidtions, then for (A), RMS=0.450; (B)=0.589; (C)=0.438; (D)=0.429.
The usual way to check whether a nuclear mass formula is good or not is to compare the calculated mass with experimental value. In this paper some concepts are summaried which check one or two terms in the mass formula separately: (ⅰ) P/P ratio-pairing energy; (ⅱ) Janecke ratio-symmetry energy; (ⅲ) A-dependence of giant resonance energy-symmetry energy; (ⅳ) IMME (isobaric multiplets mass equation)-Coulomb energy; (ⅴ) Difference of Coulomb energies-Coulomb energy; (ⅵ) β-stability lineCoulomb energy and symmetry energy; (ⅶ) Fissibility-Coulomb energy and surface energy. The following nuclear mass formulae are compared with each other: (A) Revised Weizsacker formula; (B) Danos-Gillet formula; (C) Myers-Swiatecki formula; (D) our formula. The main results are the following: (ⅰ) P/P'=1 for (A), (B) or (C);=3/4 for (D), which is in agreement with the experiment. (ⅱ) From the Janecke ratio, the form T(T+1) for the symmetry energy is better than T(T+4) or T2. (ⅲ) By the symmetry term T2/Aa, with a=0.90, the A-depeneence of giant resonance can be explained extremely well. (ⅳ) IMME, M(A, Tz)=a+bTz2+cTz2+dT z3. Only for (D), d≠0. (ⅴ) △Eo=Eo (Z+I)—Ec (Z), For (A) and (B), △EcA1/3/(Z+(1/2))=const.; for (C), △EcA1/3/(Z+(1/2)) (1—1.689)/A2/3=const.; for (D), △Ec Z2/3=const., which is in good agreement with the experiment. (ⅵ) For the β-stability line, if we compare the calculated ZA with the experimental value ZA and calculate the root mean square devidtions, then for (A), RMS=0.450; (B)=0.589; (C)=0.438; (D)=0.429.
Abstract:
In this model, the nucleus is consiered as a continuous medium with variable nucleon densities, ρp and ρn. The energy of the system is expressed by the formula:
where ρo=t/(4πa3)[1+exp((r-R)/a)]-1 is a reference density which is assumed to be the average density of an ideal nucleus with N=Z and without coulomb interactions. The binding energy and the density distributions of a nucleus were determined from the condition δE=0.The parameters were determined by fitting the nuclear masses and the general behavior of unclear charge distributions. Their preliminary values are: a=0.528 fm, t=0.3, a1=16.1027 MeV, a3=26.583 MeV, a4=15.19 MeV, a6=14.62 MeY, a2=1/2 a2, a5=1/2 a6. With this set of parameters, together with Myers and Swiatecki's formulae for shell corrcctions and pairing energies, the experimental nuclear masses can be reproduced wi thin 5 MeV and the nuclear mean wqare root radius within a few percent. These constants probably could further be improved by fitting other nuclear properties.With this new mass formula, the empirical mass difference between mirror nuclei can be reproduced within 4% (for A≥20). This is a substantial improvement over the liquid drop model. A theory of nuclear giant multipole resonance was developed by this model. Preliminary calculation on the giant dipole resonance yields rather promising results.
In this model, the nucleus is consiered as a continuous medium with variable nucleon densities, ρp and ρn. The energy of the system is expressed by the formula:
where ρo=t/(4πa3)[1+exp((r-R)/a)]-1 is a reference density which is assumed to be the average density of an ideal nucleus with N=Z and without coulomb interactions. The binding energy and the density distributions of a nucleus were determined from the condition δE=0.The parameters were determined by fitting the nuclear masses and the general behavior of unclear charge distributions. Their preliminary values are: a=0.528 fm, t=0.3, a1=16.1027 MeV, a3=26.583 MeV, a4=15.19 MeV, a6=14.62 MeY, a2=1/2 a2, a5=1/2 a6. With this set of parameters, together with Myers and Swiatecki's formulae for shell corrcctions and pairing energies, the experimental nuclear masses can be reproduced wi thin 5 MeV and the nuclear mean wqare root radius within a few percent. These constants probably could further be improved by fitting other nuclear properties.With this new mass formula, the empirical mass difference between mirror nuclei can be reproduced within 4% (for A≥20). This is a substantial improvement over the liquid drop model. A theory of nuclear giant multipole resonance was developed by this model. Preliminary calculation on the giant dipole resonance yields rather promising results.
Abstract:
Virtual excitation of GR in neutron-nucleus seattering is studied for a particular type of unclei. (Nuclei with a hole in a neutron close shell such as 87Sr.) It is found that for such type of nuclei the contribution to inelastic scattering is important even when the incident neutron energy is arround 3 MeV in contrast to the case of close shell target nuclei as investigated by V. Geramb.For an incident neutron energy En～3MeV, besides the compound nucleus formation, the following two processes also contribute to the inelastic cross section:
The intermediate states involving the virtual excitation of the GR's are treated phenomenologically as well as microscopically. In the former case they are taken to be simple resonances with definite widths. For resonable values of parameters Cλτ (or (ao)λτ), Γλτ and Δ, the contribution to the inelastic cross section due to GR's is about 10 mb. In the latter case, the GR's are taken to be a collection of 1p1h RPA phonons each with definite width. Here the coupling constant x between phonon and nucleons is taken to be 0.00337 MeV in accordance with the value given by A. Bohr, Again the contribution to the inelastic cross section is about 10 mb. One can therefore expect that this reaction mechanism is present in a variety of reactions besides (n, n') with sizable cross section. The energy of the incident partiele E at which the effect of the GR's is expected to be strongest depends on the structure of the target nucleus. For (n, n'). E～hωλτ-Sn where Sn is the neutron seperation energy of the target plus one neutron nuclei in the ground state and hωλτ is energy of GR with isospin τ and multipolarity λ.It is needed further to investigate to what extent the phenomenological treatment of the intermediate states by representing the GR as a single resonance phonon will be valid and what best values for the width should be taken.This has somthing to do with the nature of the GR's when treated as oscillations in the quantum fluid, the nuclear matter of finite extension.
Virtual excitation of GR in neutron-nucleus seattering is studied for a particular type of unclei. (Nuclei with a hole in a neutron close shell such as 87Sr.) It is found that for such type of nuclei the contribution to inelastic scattering is important even when the incident neutron energy is arround 3 MeV in contrast to the case of close shell target nuclei as investigated by V. Geramb.For an incident neutron energy En～3MeV, besides the compound nucleus formation, the following two processes also contribute to the inelastic cross section:
The intermediate states involving the virtual excitation of the GR's are treated phenomenologically as well as microscopically. In the former case they are taken to be simple resonances with definite widths. For resonable values of parameters Cλτ (or (ao)λτ), Γλτ and Δ, the contribution to the inelastic cross section due to GR's is about 10 mb. In the latter case, the GR's are taken to be a collection of 1p1h RPA phonons each with definite width. Here the coupling constant x between phonon and nucleons is taken to be 0.00337 MeV in accordance with the value given by A. Bohr, Again the contribution to the inelastic cross section is about 10 mb. One can therefore expect that this reaction mechanism is present in a variety of reactions besides (n, n') with sizable cross section. The energy of the incident partiele E at which the effect of the GR's is expected to be strongest depends on the structure of the target nucleus. For (n, n'). E～hωλτ-Sn where Sn is the neutron seperation energy of the target plus one neutron nuclei in the ground state and hωλτ is energy of GR with isospin τ and multipolarity λ.It is needed further to investigate to what extent the phenomenological treatment of the intermediate states by representing the GR as a single resonance phonon will be valid and what best values for the width should be taken.This has somthing to do with the nature of the GR's when treated as oscillations in the quantum fluid, the nuclear matter of finite extension.
Abstract:
For some gauge transformation of the general coulomb gauge, there exist a pair of fields connected by that gauge transformation. We revise the proof of Maskawa-Naka-jima theorem.
For some gauge transformation of the general coulomb gauge, there exist a pair of fields connected by that gauge transformation. We revise the proof of Maskawa-Naka-jima theorem.
Abstract:
Some properties of energy lenels for the isotopes 40Zr and 42Mo were discussedIt was then shown that the sudden change of moment of inertia was not diretly correlaled with the transition of nuclear shape but rather with the energy level density of the single particles near fermi surface.
Some properties of energy lenels for the isotopes 40Zr and 42Mo were discussedIt was then shown that the sudden change of moment of inertia was not diretly correlaled with the transition of nuclear shape but rather with the energy level density of the single particles near fermi surface.
IF: 3.6
Monthly founded in 1977
Sponsored by:
ISSN 1674-1137 CN 11-5641/O4
Original research articles, Ietters and reviews Covering theory and experiments in the fieids of
- Particle physics
- Nuclear physics
- Particle and nuclear astrophysics
- Cosmology
Author benefits
- A SCOAP3 participating journal - free Open Access publication for qualifying articles
- Average 24 days to first decision
- Fast-track publication for selected articles
- Subscriptions at over 3000 institutions worldwide
- Free English editing on all accepted articles
News
- Chinese Physics C Outstanding Reviewer Award 2023
- Impact factor of Chinese Physics C is 3.6 in 2022
- 2022 CPC Outstanding Reviewer Awards
- The 2023 Chinese New Year-Office closure
- ãChinese Physics CãBEST PAPER AWARDS 2022
Cover Story
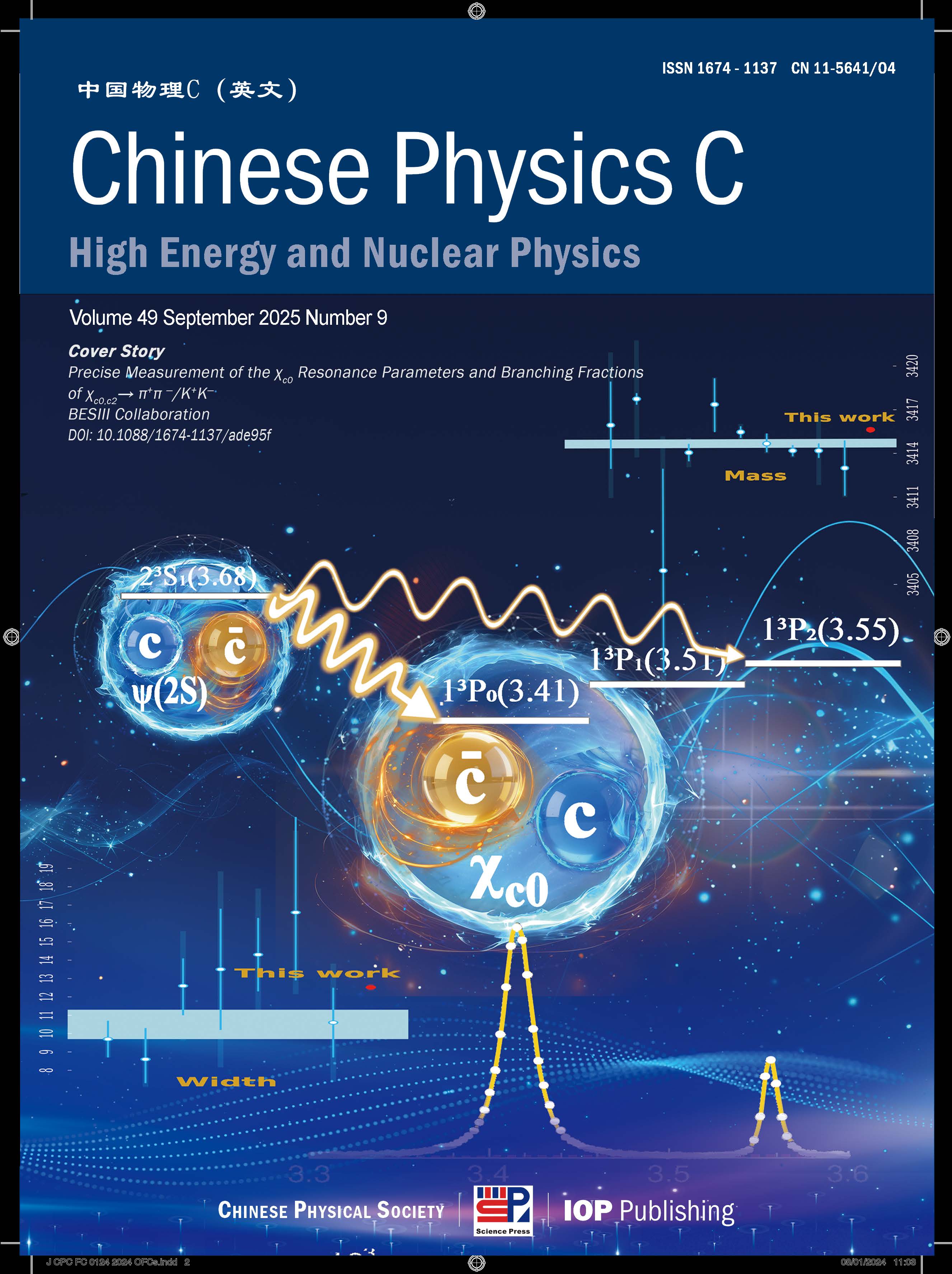
- Cover Story (Issue 9, 2024) Measurement of solar pp neutrino flux using electron recoil data from PandaX-4T commissioning run
- Cover Story (Issue 11, 2024) ï½ Form factor for Dalitz decays from J/Ï to light pseudoscalars
- Cover Story (Issue 3, 2024) | First measurement of the ground-state mass of 22Al helps to evaluate the ab-initio theory
- Cover Story (Issue 2, 2024) | Quark/gluon taggers light the way to new physics
- Cover Story (Issue 8, 2024) | Applyingdeep learning technique to chiral magnetic wave search
Advertising
