-
The correlation coefficient
$ C_{BS} = -3\dfrac{\langle BS\rangle-\langle B\rangle\langle S\rangle}{\langle S^2\rangle-\langle S\rangle^2} $ between the baryon number B and the strangeness number S in a strongly interacting matter was first proposed in Refs. [1-3] for probing the properties of the matter produced in relativistic heavy-ion collisions. A later study suggested, however, that the strangeness population factor$ S_3 = $ $ \dfrac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}/{{{N}}_\Lambda }}}{{{{{N}}_{^3{\rm{He}}}}/{{{N}}_{{p}}}}} $ measured in these collisions could serve as a better probe of the baryon number and strangeness correlation in the produced matter, owing to its different behaviors in QGP and the hadronic matter [4, 5]. Experimentally,$ S_3 $ increases from heavy-ion collisions at AGS [6] to RHIC energy [7], and then decreases to a small value in collisions at the LHC energy [8]. Compared with the predictions of the statistical model [5, 9, 10], the values of$ S_3 $ extracted from the RHIC data, which has large statistical uncertainties, are larger, which led to questioning the data and its interpretation. As to the different values of$ S_3 $ measured at RHIC and LHC, a possible explanation was provided in Ref. [11] by assuming an early freeze-out of$ \Lambda $ compared with nucleons from hadronic matter, and a longer freeze-out time difference at RHIC than at LHC. This idea was further explored in Ref. [12] to study the production of light nuclei in relativistic heavy-ion collisions by considering their finite sizes compared with the size of the produced hadronic matter at the kinetic freeze out.Since the first theoretical estimate (in the 1970s) of the abundance of hypernuclei that could be produced in heavy-ion collisions [13], many studies addressed this very interesting problem, providing increasingly better estimations [6-8, 14-17] (more details can be found in recent topical reviews [18-20]). For the lightest hypernucleus
$ \rm ^3_{\Lambda}H $ , the separation energy of its$ \Lambda $ was very small in early measurements, with a typical value of$ 130 \pm 50 $ keV [21], but a larger value of$ 410 \pm 120 \pm 110 $ keV has been suggested based on some recent measurements and using a more precise method [22]. Owing to this significantly smaller$ \Lambda $ separation energy than the nucleon separation energy in normal nuclei with a similar mass number [23],$ {_\Lambda ^3{\rm{H}}} $ can be considered as a loosely bound$ \rm d-\Lambda $ 2-body system. In relativistic heavy-ion collisions, the hypertriton is expected to be in chemical equilibrium with$ \Lambda $ and deuteron as well as with proton and neutron; then, its yield is the same whether it is calculated from the coalescence of$ \Lambda $ with deuteron or with proton and neutron. As shown in Ref. [17] based on the coalescence model, the yields of hypertritons from these two processes are approximately the same, and the difference is owing to the simplification of taking deuteron as a point particle in this calculation. Therefore, the same$ \rm ^3_{\Lambda}H $ yield appears in both$ S_2 $ and$ S_3 $ , and the ratio$ S_2 = $ $ \dfrac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}}}{{{{{N}}_\Lambda }{{{N}}_{{d}}}}} $ can thus also be used as an observable for probing the correlation between baryon and strangeness in relativistic heavy-ion collisions.In this paper, we study the yield ratios
$ \rm S_3 = $ $ \dfrac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}/{{{N}}_\Lambda }}}{{{{{N}}_{^3{\rm{He}}}}/{{{N}}_{{p}}}}} $ and$ S_2 = $ $ \dfrac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}}}{{{{{N}}_\Lambda }{{{N}}_{{d}}}}} $ in the framework of the coalescence model. We first revisit the study of$ S_3 $ and find that the discrepancy between the ALICE and STAR measurements may be partially owing to the difference in the primordial proton yield used in the two analyses. We then show that the ratio$ S_2 $ , particularly its ratio$ S_2/B_2 $ with respect to$ B_2 $ , which is the coalescence parameter for the production of a deuteron from a proton and neutron pair, is a cleaner probe of the baryon-strangeness correlation in the produced hadronic matter from relativistic heavy-ion collisions. -
In this Section, we first review the experimental data on
$ S_3 $ from relativistic heavy-ion collisions and then use the coalescence model to extract from these data the correlation between$ \Lambda $ and nucleon density fluctuations in the produced matter. -
The value of
$ S_3 $ was measured to be$ 0.36 \pm 0.26 $ in central 11.5A GeV/c Au + Pt collisions [6] and increased to$ 1.08 \pm 0.22 \pm 0.16 $ for Au+Au collisions at$ \rm \sqrt{s_{NN}} $ = 200 GeV with a mixed event sample of a central trigger and a minimal bias trigger [7]. The measured value of$ S_3 $ decreases, however, to$ 0.60 \pm 0.13 \pm 0.21 $ in central Pb-Pb collisions at$ \rm \sqrt{s_{NN}} $ = 2.76 TeV [8]. Preliminary data with an improved precision from Au+Au collisions at$ \rm \sqrt{s_{NN}} $ = 200 GeV revealed a 20% reduction in the value of$ \rm S_3 $ [24], which makes the results from STAR and ALICE comparable within their experimental uncertainties. The proton yield used in the analysis of the$ S_3 $ ratio by the STAR Collaboration is based on the subtraction of protons from the decay of hyperons in its measurements. Replacing the proton yield in the STAR analysis with that from the PHENIX data, which is obtained from a theoretical model for the same collision system and energy [25], can also reduce the value of$ S_3 $ at$ \rm \sqrt{s_{NN}} $ = 200 GeV to a similar value as measured by ALICE [7, 8, 24]. The difference between the results from STAR and ALICE is thus partially owing to the different treatments in the subtraction of the weak decay contribution to the primordial proton yield. Table 1 summarizes the published$ S_3 $ results, together with the value using the proton yield from the PHENIX data in Au+Au collisions at$ \rm \sqrt{s_{NN}} $ = 200 GeV. It is seen that the values of$ S_3 $ from STAR and ALICE are now comparable within their large uncertainties.experiment $ S_3 $ 

AGS $ 0.36 \pm 0.26 $ 

STAR $ 1.08 \pm 0.22 \pm 0.16 $ 

STAR + PH $ 0.90 \pm 0.22 \pm 0.15 $ 

ALICE $ 0.60 \pm 0.13 \pm 0.21 $ 

Table 1. Values of
$ S_3 $ from AGS, STAR and ALICE, with PH indicating that the proton yield is taken from PHENIX [25]. See text for details.Experimentally, there also exists a puzzle related to the
$ \rm ^3_{\Lambda}H/{^3{\rm{He}}} $ ratio [10, 11]. Its value is$ 0.82 \pm 0.16 \pm 0.12 $ in the 0-80% centrality of Au+Au collisions at$ \rm \sqrt{s_{NN}} $ = 200 GeV at RHIC [7], which is considerably larger than the value of$ 0.47 \pm 0.10 \pm 0.13 $ in Pb-Pb collisions at$ \rm \sqrt{s_{NN}} $ = 2.76 TeV and the 0-10% centrality at the LHC [8]. Although a preliminary measurement with improved precision by STAR in Au+Au collisions at$ \rm \sqrt{s_{NN}} $ = 200 GeV revealed a reduction in the$ \rm ^3_{\Lambda}H/{^3{\rm{He}}} $ ratio to a value comparable with that assessed by the ALICE study, its large uncertainty [26] indicates that more precise measurements of$ {_\Lambda ^3{\rm{H}}} $ in high energy heavy-ion collisions are needed. -
To demonstrate the physics that can be extracted from the ratio
$ \rm S_3 $ , we adopted the coalescence model for the present study. According to the coalescence formula denoted as COAL-SH in Ref. [27], the yield of a certain nucleus consisting of$ N_i $ constituent species i (proton, neutron, and$ \Lambda $ ) of mass$ m_i $ from the kinetically frozen-out hadronic matter of local temperature$ T_{\rm K} $ and volume$ V_{\rm K} $ in a heavy-ion collision can be written as$\begin{split}{{{N}}_{{A}}}=& {{{g}}_{{\rm{rel}}}}{{{g}}_{{\rm{size}}}}{{{g}}_{{A}}}{\left( {\sum\limits_{{i}}^{{A}} {{{{m}}_{{i}}}} } \right)^{{\rm{3/2}}}}\left[ {\prod\limits_{{{i = 1}}}^{{A}} {\frac{{{{{N}}_{{i}}}}}{{{{m}}_{{i}}^{{\rm{3/2}}}}}} } \right]\\& \times \prod\limits_{{{i = 1}}}^{{{A - 1}}} {\frac{{{{({\rm{4}}\pi {\rm{/}}\omega )}^{{\rm{3/2}}}}}}{{{{{V}}_{\rm{K}}}{{x}}({\rm{1 + }}{{{x}}^{\rm{2}}})}}} {\left( {\frac{{{{{x}}^{\rm{2}}}}}{{{\rm{1 + }}{{{x}}^{\rm{2}}}}}} \right)^{{{\rm{l}}_{{i}}}}}{{G}}({{{l}}_{{i}}},{{x}}).\end{split}$

(1) In the above,
$ g_{A} = (2S+1)/(\prod_{i = 1}^A(2s_i+1)) $ is the statistical factor for A nucleons and/or$ \Lambda $ of spin$ s_i = 1/2 $ to form a nucleus of spin S;$ g_{\rm rel} $ is the relativistic correction to the effective volume in the momentum space and is set to 1 in the present study, owing to the much larger nucleon mass than the effective temperature of the hadronic matter at kinetic freeze-out; and$ g_{\rm size} $ is the correction owing to the finite size of the produced nucleus and is also taken to be 1 in our study because of the much larger size of the hadronic matter than the sizes of produced light nuclei. The symbols$ l_i $ and$ \omega $ denote, respectively, the orbital angular momentum of the nucleon or$ \Lambda $ in the nucleus and the oscillator constant used in its wave function. The value of$ x = (2T_{\rm K}/\omega)^{1/2} $ is significantly larger than one because of the much larger size of the nucleus than the thermal wavelength of its constituents in the hadronic matter. Since the light nuclei considered in the present study all involve only the$ l = 0 $ s-wave, the suppression factor$ G(l_i,x) $ owing to the orbital angular momentum in the above equation is simply one.For the production of
$ {_\Lambda ^3{\rm{H}}} $ and$ {^3{\rm{He}}} $ , their yields according to Eq. (1), after taking into account the approximations mentioned above, are given by$\begin{split} {{{N}}_{_\Lambda ^3{\rm{H}}}}=&{{{g}}_{_\Lambda ^3{\rm{H}}}}\frac{{{{({{{m}}_\Lambda }{\rm{ + }}{{{m}}_{{p}}}{\rm{ + }}{{{m}}_{{n}}})}^{{\rm{3/2}}}}}}{{{{m}}_\Lambda ^{{\rm{3/2}}}{{m}}_{{p}}^{{\rm{3/2}}}{{m}}_{{n}}^{{\rm{3/2}}}}}{\left( {\frac{{{\rm{2}}\pi }}{{{{{T}}_{\rm{K}}}}}} \right)^{\rm{3}}}\frac{{{{{N}}_\Lambda }{{{N}}_{{p}}}{{{N}}_{{n}}}}}{{{{V}}_{\rm{K}}^{\rm{2}}}},\\ {{{N}}_{^3{{\rm He}}}} =&{{{g}}_{^3{\rm{He}}}}\frac{{{{({\rm{2}}{{{m}}_{{p}}}{\rm{ + }}{{{m}}_{{n}}})}^{{\rm{3/2}}}}}}{{{{m}}_{{p}}^{\rm {3}}{{m}}_{{n}}^{{\rm{3/2}}}}}{\left( {\frac{{{\rm{2}}\pi }}{{{{{T}}_{\rm{K}}}}}} \right)^{\rm{3}}}\frac{{{{N}}_{{p}}^{\rm{2}}{{{N}}_{{n}}}}}{{{{V}}_{\rm{K}}^{\rm{2}}}}. \end{split}$

(2) According to Refs. [28, 29], including possible nucleon and
$ \Lambda $ density fluctuations in heavy-ion collisions at lower energies owing to the spinodal instability during the QGP to hadronic matter phase transition [30-32], modifies the above equations to$\begin{split} {{{N}}_{_\Lambda ^3{\rm{H}}}} \approx & {{{g}}_{_\Lambda ^3{\rm{H}}}}\frac{{{{({{{m}}_\Lambda }{\rm{ + }}{{{m}}_{{p}}}{\rm{ + }}{{{m}}_{{n}}})}^{{\rm{3/2}}}}}}{{{{m}}_\Lambda ^{{\rm{3/2}}}{{m}}_{{p}}^{{\rm{3/2}}}{{m}}_{{n}}^{{\rm {3/2}}}}}{\left(\frac{{{\rm{2}}\pi }}{{{{{T}}_{\rm{K}}}}}\right)^{\rm{3}}}\frac{{{{{N}}_\Lambda }{{{N}}_{{p}}}{{{N}}_{{n}}}}}{{{{V}}_{\rm{K}}^{\rm{2}}}}({\rm{1 \!+\! }}{\alpha _{\Lambda {{p}}}}{\rm{\! +\! }}{\alpha _{\Lambda {{n}}}}{\rm{\! +\! }}{\alpha _{{{np}}}}),\\ {{{N}}_{^3{\rm{He}}}} \approx & {{{g}}_{^3{\rm{He}}}}\frac{{{{({\rm{2}}{{{m}}_{{p}}}{\rm{ + }}{{{m}}_{{n}}})}^{{\rm{3/2}}}}}}{{{{m}}_{{p}}^{\rm{3}}{{m}}_{{n}}^{{\rm{3/2}}}}}{\left(\frac{{{\rm{2}}\pi }}{{{{{T}}_{\rm{K}}}}}\right)^{\rm{3}}}\frac{{{{N}}_{{p}}^{\rm{2}}{{{N}}_{{n}}}}}{{{{V}}_{\rm{K}}^{\rm{2}}}}({\rm{1 + }}\Delta {{p + 2}}{\alpha _{{{np}}}}). \end{split}$

(3) In the above, the proton relative density fluctuation is denoted by
$ \Delta p = \langle (\delta p)^2\rangle/\langle p\rangle^2 $ , where$\begin{split}\langle p\rangle =& \frac{1}{{{V_{\rm K}}}}\int {{n_p}} (\vec r){\rm d}\vec r,\\ \langle {(\delta p)^2}\rangle =& \frac{1}{{{V_{\rm K}}}}\smallint {[{n_p}(\vec r) - \langle {n_p}\rangle ]^2}{\rm d}\vec r, \end{split}$

(4) with
$ n_p(\vec r) $ being the proton density distribution. The quantities$ \alpha_{\Lambda p} $ ,$ \alpha_{\Lambda n} $ , and$ \alpha_{np} $ are, respectively, the$ \Lambda $ -proton,$ \Lambda $ -neutron, and proton-neutron density fluctuation correlation coefficients$ \alpha_{n_1 n_2} = \langle \delta n_1 \delta n_2\rangle/(\langle n_1\rangle\langle n_2\rangle) $ with$ n_1 $ and$ n_2 $ denoting$ \Lambda $ or a nucleon.Taking the same masses for proton and neutron, i.e.,
$ m_p = m_n = m $ , the yield ratio$ S_3 = $ $ \dfrac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}/{{{N}}_\Lambda }}}{{{{{N}}_{^3{\rm{He}}}}/{{{N}}_{{p}}}}} $ is then${{{S}}_{\rm{3}}}{{ = g}}\frac{{{\rm{1 + }}{\alpha _{\Lambda {{p}}}}{\rm{ + }}{\alpha _{\Lambda {{n}}}}{\rm{ + }}{\alpha _{{{np}}}}}}{{{\rm{1 + }}\Delta {{p + 2}}{\alpha _{{{np}}}}}},$

(5) with
$ g = \left(\dfrac{m_\Lambda+2m}{3m_{\Lambda}}\right)^{3/2}\approx 0.845 $ . From the above equation, the sum of the correlation coefficients$ \alpha_{\Lambda p} $ +$ \alpha_{\Lambda n} $ between the$ \Lambda $ density and the proton or neutron density fluctuations can be determined from$ S_3 $ ,$ \Delta p $ , and$ \alpha_{np} $ according to${\alpha _{\Lambda {{p}}}}{\rm{ + }}{\alpha _{\Lambda {{n}}}}{\rm{ = }}\frac{{{{{S}}_{\rm{3}}}}}{{{g}}} \times ({\rm{1 + }}\Delta {{p + 2}}{\alpha _{{{np}}}}){\rm{ - }}{\alpha _{{{np}}}}{\rm{ - 1}}.$

(6) For the value of
$ \alpha_{np} $ , we follow the method in Ref. [29] by using the deuteron yield after including in the coalescence formula COAL-SH the proton and neutron density fluctuations, i.e.,${{{N}}_{{d}}}{\rm{ = }}{{\rm{2}}^{{\rm{3/2}}}}{{{g}}_{{d}}}{\left( {\frac{{{\rm{2}}\pi }}{{{{m}}{{{T}}_{\rm{K}}}}}} \right)^{{\rm{3/2}}}}\frac{{{{{N}}_{{p}}}{{{N}}_{{n}}}}}{{{{{V}}_{\rm{K}}}}}({\rm{1 + }}{\alpha _{{{np}}}}).$

(7) In terms of
$ g_{d-p} = \dfrac{1}{2^{3/2}g_d(2\pi)^3} = \dfrac{2^{1/2}}{3(2\pi)^3} \approx 0.0019 $ ,$ {\cal O}_{d-p} = N_d/N_p^2 $ ,$ R_{np} = N_p/N_n $ , and$ V_{ph} = (2\pi mT_{\rm K})^{3/2}V_{\rm K} $ , the value of$ \alpha_{np} $ can then be calculated from${\alpha _{{{np}}}}{\rm{ = }}{{{g}}_{{{d - p}}}}{{{R}}_{{{np}}}}{{{V}}_{{{ph}}}}{{\cal O}_{{{d - p}}}}{\rm{ - 1}}.$

(8) For the proton density fluctuation
$ \Delta p $ , we consider the ratio${{{N}}_{^3{\rm{He}}}}{{N}}_{{p}}^{\rm{3}} \times \frac{{{{{N}}_{{p}}}}}{{{{{N}}_{{n}}}}}{\rm{ = }}{{\rm{3}}^{{\rm{3/2}}}}{{{g}}_{^3{\rm{He}}}}{\left( {\frac{{{\rm{2}}\pi }}{{{{m}}{{{T}}_{\rm{K}}}}}} \right)^{\rm{3}}}\frac{{\rm{1}}}{{{{V}}_{\rm{K}}^{\rm{2}}}}({\rm{1 + }}\Delta {{p + 2}}{\alpha _{{{np}}}}),$

(9) and determine it from the relation
$\Delta {{p = }}{{{g}}_{^3{\rm{He}} - {{p}}}}{{V}}_{{{ph}}}^{\rm{2}}{{{R}}_{{{np}}}}{{\cal O}_{^3{\rm{He}} - {{p}}}}{\rm{ - 2}}{\alpha _{{{np}}}}{\rm{ - 1}},$

(10) where
$ g_{^3{\rm H}e-p} = \dfrac{1}{3^{3/2}g_{^3{\rm He}}(2\pi)^6} = \dfrac{4}{3^{3/2}(2\pi)^6} \approx 1.25\times10^{-5} $ and$ {\cal O}_{^3{\rm He}-p} = N_{^3{\rm H}e}/N_p^3 $ , and the factors$ V_{ph} $ ,$ R_{np} $ and$ \alpha_{np} $ are the same as above.The effective phase-space volume
$ V_{ph} $ occupied by nucleons in the hadronic matter at kinetic freeze-out can be evaluated from its value at chemical freeze-out, using the relation$ T_{\rm K}^{3/2}V_{\rm K} = \lambda T_{\rm ch}^{3/2}V_{\rm ch} $ , where$ T_{\rm ch} $ and$ V_{\rm ch} $ are, respectively, the temperature and volume of the system at the chemical freeze-out, and$ \lambda $ is a parameter. For collisions at RHIC energies, we take the value of$ T_{\rm ch} $ from the grand canonical ensemble fits to the particle yields in Ref. [33] and that of$ \rm V_{\rm ch} $ to be$ V_{\rm ch} = 4 \mathrm \pi R^3/3 $ as in Ref. [33] for collisions at various centralities, except for collisions at the 0-80% centrality, where it is taken to be proportional to the charged particle multiplicity obtained from Ref. [34]. Using the results from Ref. [33] based on the strangeness suppressed canonical ensemble fits gives almost the same results. The different values of$ V_{\rm ch} $ extracted from collisions at$ \sqrt{s_{NN}} $ = 11.5 and 19.6 GeV are owing to the neglect of experimental uncertainties in our analysis [33]. The values of$ T_{\rm ch} $ and$ V_{\rm ch} $ used in the present study for collisions at the AGS energy are taken from Ref. [35], and for collisions at the LHC energy, they are taken from the COAL-SH model used in Ref. [27]. In our calculations, all hadrons are taken as point particles. Including an exclusive volume for each hadron increases$ V_{\rm ch} $ [35]. The value of$ \lambda $ is determined by assuming that the entropy associated with a single nucleon is the same at the chemical and the kinetic freeze-outs. Explicitly, we use the well-known expression for the entropy associated with a single nucleon in a system in thermal equilibrium, i.e.,$ S/N = 5/2+{\rm{ln}}(V_{{ph}}/N) $ , where$ V_{{ph}} = (2\pi mT)^{3/2}V $ is the phase-space volume of N nucleons of mass m in the system. The constancy of$ S/N $ then requires$ T_{\rm ch}^{3/2}V_{\rm ch}/ N_{\rm ch} = T_{\rm K}^{3/2}V_{\rm K}/N_{\rm K} $ , with$ N_{\rm{ch}} $ and$ N_{\rm{K}} $ being the numbers of nucleons at the chemical and kinetic freeze-outs, respectively, which then leads to$ \lambda = N_{\rm{K}}/N_{\rm{ch}} $ . The values given in Table 2 for$ \lambda $ are obtained from the value$ N_{\rm K} $ measured in experiments and the value$ N_{\rm ch} $ given by the statistical hadronization model that includes all the resonances in PDG [36]. Our approach differs from the naive approach of a hadronic matter of constant number of nucleons expanding with a constant total nucleon entropy after a chemical freeze-out, which would give$ \lambda $ the value of unity, and also that in Ref. [29] based on a multiphase transport model that only includes a small number of resonances and thus gives a smaller value of$ \lambda\approx 1.6 $ . We note that the below results of our study are not qualitatively affected by these variations in$ \lambda $ . As to the value of$ R_{np} = N_p/N_n $ , it can be determined from the measured ratio of charged pions according to the relation$ N_p/N_n = (N_{\pi^+}/N_{\pi^-})^{1/2} $ from the statistical model. In Table 2, we summarize the values of the above parameters for central heavy-ion collisions. For reference, we also provide in Table 3 their values for collisions at the centralities of 0-80% or 0-60%.$\sqrt{s_{NN} } /{\rm GeV}$ 

$ T_{\rm ch} /{\rm GeV} $ 

$ V_{\rm ch} /{\rm fm}^3 $ 

$ R_{np} $ 

$ \alpha_{np} $ 

$ \lambda $ 

4.9 0.132 640 0.925 $ -0.781\pm0.026 $ 

2.23 7.7 0.144 806 0.966 $ -0.744\pm0.024 $ 

2.60 11.5 0.151 875 0.977 $ -0.763\pm0.019 $ 

2.76 19.6 0.158 843 0.987 $ -0.830\pm0.014 $ 

2.92 27 0.160 846 0.988 $ -0.848\pm0.012 $ 

2.97 39 0.160 951 0.990 $ -0.834\pm0.013 $ 

3.00 62.4 0.164 1215 0.992 $ -0.792\pm0.037 $ 

3.16 200 0.168 1334 0.992 $ -0.726\pm0.038 $ 

3.30 2760 0.156 4320 1.00 $ -0.717\pm0.023 $ 

2.94 Table 2. Values of parameters used for 0-10% central collisions.
$ \sqrt{s_{NN}} /{\rm GeV} $ 

$ T_{\rm ch} /{\rm GeV} $ 

$ V_{\rm ch} /{\rm fm}^3 $ 

$ R_{np} $ 

$ \alpha_{np} $ 

$ \lambda $ 

7.7 0.144 268 0.966 $ -0.775\pm0.020 $ 

2.60 11.5 0.151 292 0.977 $ -0.793\pm0.018 $ 

2.76 19.6 0.158 281 0.987 $ -0.851\pm0.014 $ 

2.92 27 0.160 282 0.988 $ -0.867\pm0.012 $ 

2.97 39 0.160 317 0.990 $ -0.859\pm0.013 $ 

3.00 200 0.168 445 0.992 $ -0.747\pm0.036 $ 

3.30 2760 0.156 1800 1.00 $ -0.710\pm0.024 $ 

2.94 Table 3. Same as Table 2 for values of parameters used in the calculations for 0-80% centrality in collisions at RHIC energies and for 0-60% centrality at the LHC energy.
We note that if we have assumed instead that the total entropy is the same at the chemical and kinetic freeze-outs, i.e.,
$ T_{\rm K}^3V_K = T_{\rm ch}^3V_{\rm ch} $ [37], a somewhat smaller$ V_{\rm K} $ would have been obtained. Since it has been shown in Ref. [38] that the total entropy increases from the chemical to the kinetic freeze-out, and the entropy associated with a baryon is essentially constant, we adopt in the present study the condition$ T_{\rm K}^{3/2}V_{\rm K} = \lambda T_{\rm ch}^{3/2}V_{\rm ch} $ of constant entropy associated with a nucleon. Although the values of$ T_{\rm K} $ are not used in our calculations, it is useful to show them as references. According to Ref. [33] based on a blast-wave model fit to measured proton, pion, and kaon transverse momentum spectra, the values of$ T_{\rm K} $ are 126, 117, 119, 114, 116, 118, 88, 88 MeV for central heavy-ion collisions at$ \sqrt{s_{NN}} $ = 4.9, 7.7, 11.5, 19.6, 27, 39, 200, and 2760 GeV, respectively.As shown in Tables 2 and 3, the extracted values for the neutron and proton density fluctuation correlation
$ \alpha_{np} $ are negative with appreciable magnitude, indicating that neutrons and protons are anti-correlated in the matter produced in relativistic heavy-ion collisions, similar to the findings in Ref. [29]. For the proton density fluctuation$ \Delta p $ , the extracted values are 0.656$ \pm $ 0.049, 0.536$ \pm $ 0.083, and 0.727$ \pm $ 0.085 for collisions at$ \sqrt{s_{NN}} $ = 4.9, 200, and 2760 GeV, respectively [6, 7, 39]. It shows a non-monotonic behavior as a function of the collision energy, from$ \sqrt{s_{NN}} $ = 7.7 GeV to 200 GeV, using the preliminary data of$ {^3{\rm{He}}} $ yield from Ref. [24], similar to that of the neutron density fluctuation extracted from the yield ratio$ \dfrac{N_t N_p}{N_{d}^2} $ [40]. From the measured values of$ S_3 $ and extracted values for$ \alpha_{np} $ and$ \ \Delta p $ , one can then determine the values of$ \alpha_{\Lambda n}+\alpha_{\Lambda p} $ from heavy-ion collisions at various energies, according to Eq. (6). These results will be shown in the next Section, to compare with the correlation coefficient$ \alpha_{\Lambda d} $ of the$ \Lambda $ and deuteron density fluctuations that is extracted from measured$ S_2 = $ $ \dfrac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}}}{{{{{N}}_\Lambda }{{{N}}_{{d}}}}} $ ratio. -
In this Section, we first consider the
$ S_2 = $ $ \dfrac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}}}{{{{{N}}_\Lambda }{{{N}}_{{d}}}}} $ ratio in the framework of the coalescence model. Its ratio$ S_2/B_2 $ with respect to the coalescence parameter$ B_2 $ for the production of a deuteron from the coalescence of a proton and a neutron is then studied. Based on the experimental data from the RHIC and LHC, we further extract the correlation coefficient$ \rm \alpha_{\Lambda d} $ between the$ \Lambda $ and deuteron density fluctuations. -
Approximating
$ {_\Lambda ^3{\rm{H}}} $ as a bound system of$ \Lambda $ and a deuteron, the yield of$ {_\Lambda ^3{\rm{H}}} $ in heavy-ion collisions can be calculated from the coalescence of$ \Lambda $ and a deuteron using Eq. (1). Including the effect of the deuteron and$ \Lambda $ density fluctuations, it is given by${{{N}}_{_\Lambda ^3{\rm{H}}}}{\rm{ = }}{{{g}}_{_\Lambda ^3{\rm{H}}}}\frac{{{{\left({{{m}}_\Lambda }{\rm{ + }}{{{m}}_{{d}}}\right)}^{{\rm{3/2}}}}}}{{{{m}}_\Lambda ^{{\rm{3/2}}}{{m}}_{{d}}^{{\rm{3/2}}}}}{\left(\frac {{{\rm{2}}\pi }}{{{{{T}}_{\rm{K}}}}}\right)^{{\rm{3/2}}}}\frac{{{{{N}}_\Lambda }{{{N}}_{{d}}}}}{{{{{V}}_{\rm{K}}}}}({\rm{1 + }}{\alpha _{\Lambda {{d}}}}),$

(11) with
$ \alpha_{\Lambda d} = \langle \delta n_{\Lambda}\delta n_{d}\rangle/(\langle n_{\Lambda}\rangle\langle n_{d}\rangle) $ being the correlation coefficient between the deuteron and$ \Lambda $ density fluctuations. The$ S_2 $ ratio is then${{{S}}_{\rm{2}}}{\rm{ = }}\frac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}}}{{{{{N}}_\Lambda }{{{N}}_{{d}}}}}{\rm{ = }}{{{g}}_{_\Lambda ^3{\rm{H}}}}\frac{{{{\left({{{m}}_\Lambda }{\rm{ + }}{{{m}}_{{d}}}\right)}^{{\rm{3/2}}}}}}{{{{m}}_\Lambda ^{{\rm{3/2}}}{{m}}_{{d}}^{{\rm{3/2}}}}}{\left(\frac{{{\rm{2}}\pi }}{{{{{T}}_{\rm{K}}}}}\right)^{{\rm{3/2}}}}\frac{{\rm{1}}}{{{{{V}}_{\rm{K}}}}}({\rm{1 + }}{\alpha _{\Lambda {{d}}}}),$

(12) with
$ g_{S_2} = \left(\dfrac{1}{3}\dfrac{(m_{\Lambda}+m_d)^{3/2}}{m_{\Lambda}^{3/2}m_d^{3/2}}(2\pi)^{3/2}\right)^{-1} \approx 0.12 $ , from which we can express the density fluctuation correlation coefficient$ \alpha_{\Lambda d} $ in terms of$ S_2 $ as${\alpha _{\Lambda {{d}}}}{\rm{ = }}{{{g}}_{{{{S}}_{\rm{2}}}}}{{{S}}_{\rm{2}}}{{T}}_{\rm{K}}^{{\rm{3/2}}}{{{V}}_{\rm{K}}}{\rm{ - 1}}.$

(13) In the left plot of Fig. 1, we show by solid symbols the extracted ratio
$ S_2 = $ $ \dfrac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}}}{{{{{N}}_\Lambda }{{{N}}_{{d}}}}} $ using the experimental data from AGS [6, 41, 42], RHIC [7, 43, 44], and LHC [8, 39, 45]. Open symbols represent the results for the$ {_\Lambda ^3{\rm{H}}} $ yield in the collision at the 0-10% centrality, where the RHIC data are obtained from multiplying the measured$ {_\Lambda ^3{\rm{H}}} $ data at the 0-80% centrality by a factor of 3. We have checked, using data available from the RHIC BES program, that the deuteron to$ \Lambda $ yield ratio is larger by a factor of 3 for collisions at the 0-10% centrality than at the 0-80% centrality, independent of the collision energy [43, 46]. Solid and dashed lines in the left window of Fig. 1 are results from calculations based on the statistical model of Refs. [47-49] as in Ref. [50] using the parameters in Tables 2 and 3 and after taking into account the feed-down correction to the$ \Lambda $ yield. Clearly, although there are missing data points in the large collision energy range, the ratio$ S_2 $ in collisions at the 0-10% centrality seems to be independent of the collision energy$ \sqrt{s_{NN}} $ considering the large uncertainty of the AGS data. In addition, the model calculation describes reasonably well the data at the LHC energy, but over-predicts the results for collisions at the AGS and RHIC energies.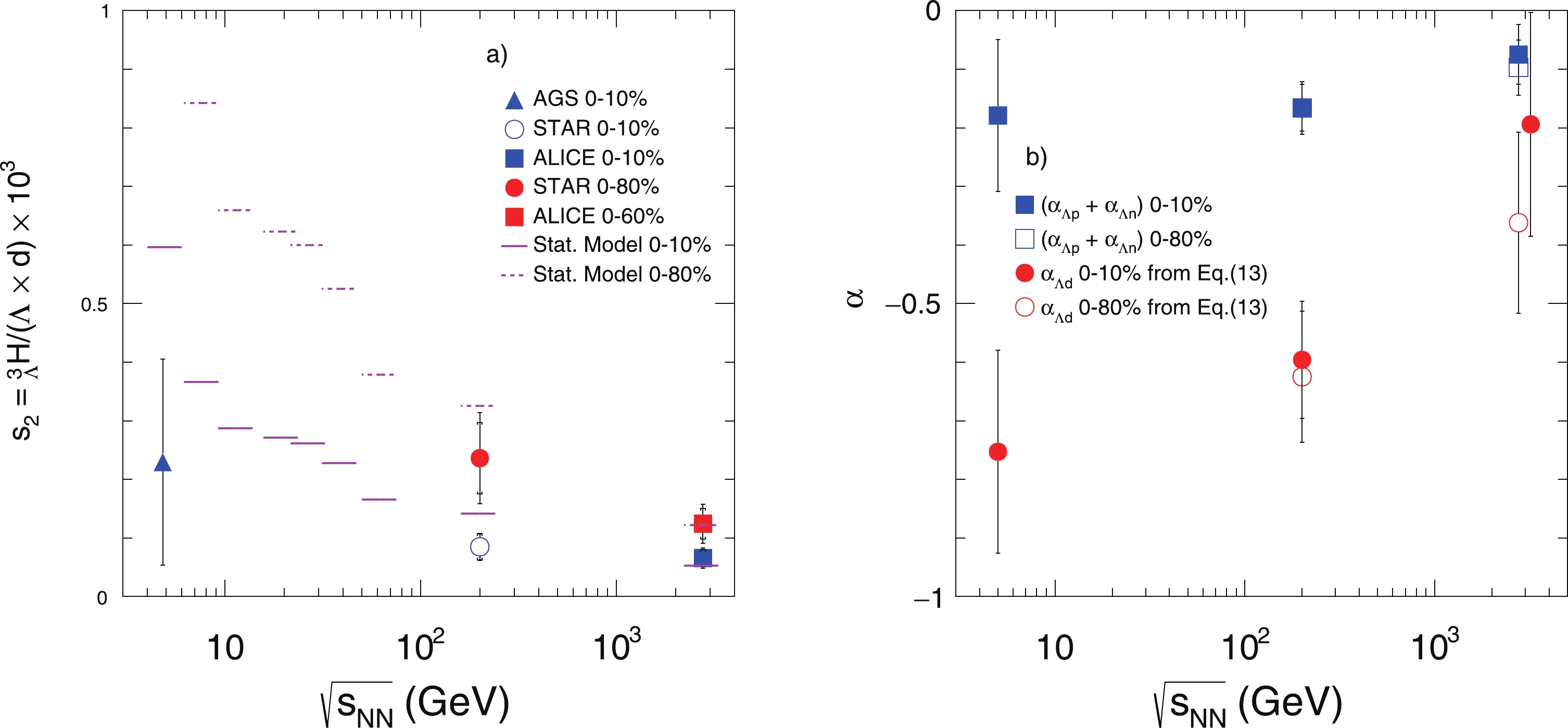
Figure 1. (color online) Collision energy dependence of the
${{{S}}_{\rm{2}}} = \dfrac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}}}{{{{{N}}_\Lambda }{{{N}}_{{d}}}}}$ ratio (left) and the density fluctuation correlation coefficients$ \alpha_{\Lambda p} + \alpha_{\Lambda n}$ and$ \alpha_{\Lambda d}$ (right) extracted from the experimental data (symbols) and calculated from the statistical model (horizontal bars) using parameters in Tables 2 and 3. See text for details.The right plot in Fig. 1 shows the value of the correlation coefficients
$ \rm \alpha_{\Lambda d} $ as a function of the collision energy for collisions at the 0-10% centrality (solid symbols) and at the 0-80% centrality (open symbols). The large uncertainty at$ \rm \sqrt{s_{NN}} $ = 2.76 TeV is owing to the propagation of the standard error from the large volume$ V_{\rm ch} $ used in collisions at the LHC energy. Within current experimental uncertainties, the value of$ \alpha_{\Lambda d} $ , which is negative and thus indicates an anti-correlation between the$ \Lambda $ and deuteron density fluctuations, becomes slightly less negative as the collision energy increases and approaches zero at$ \sqrt{s_{NN}} $ = 2.76 TeV. The negative$ \alpha_{\Lambda d} $ and the negative$ \alpha_{np} $ , shown in Tables 2 and 3, respectively, could be owing to the underestimation of the value of the$ \lambda $ parameter or the kinetic freeze-out volume used in our study. Full understanding of these results requires detailed studies based on the microscopic models of light cluster production in high-energy heavy-ion collisions [51, 52], which is, however, beyond the scope of the present study. Compared with the correlation coefficient$ \alpha_{\Lambda p}+\alpha_{\Lambda n} $ extracted from$ S_3 $ , which seems to vary very little over a broad range of collision energies, the value of$ \alpha_{\Lambda d} $ shows a more visible$ \sqrt{s_{NN}} $ dependence. Also, the deviation of$ \alpha_{\Lambda p}+\alpha_{\Lambda n} $ from zero is larger than that of$ \alpha_{\Lambda p}+\alpha_{\Lambda n} $ . This may suggest that$ \alpha_{\Lambda d} $ is a cleaner observable than$ \alpha_{\Lambda p}+\alpha_{\Lambda n} $ for studying the$ \sqrt{s_{NN}} $ dependence of baryon density fluctuations and their correlations, as seen from the comparison of Eq. (12) to Eq. (5). Future experimental measurements in a broad range of collision energies from AGS to RHIC will be very useful for shedding light on the underlying physics. -
In the coalescence model, the yield ratio
$ S_2 = N_{^3_{\Lambda}{\rm H}}/(N_{\Lambda}N_d) $ is the coalescence parameter for the production of$ \rm ^3_{\Lambda}H $ if it is considered as a bound system of$ \Lambda $ and a deuteron. Because of the strangeness carried by$ \Lambda $ , the$ S_2 $ may be different from the coalescence parameter$ B_2 $ for the deuteron production following the coalescence of a proton and a neutron [19, 53-57].From Eq. (12) for
$ S_2 $ and a similar equation for$ B_2 $ , given by${{{B}}_{\rm{2}}}{\rm{ = }}\frac{{{{{N}}_{{d}}}}}{{{{{N}}_{{p}}}{{{N}}_{{n}}}}}{\rm{ = }}{{{g}}_{{d}}}\frac{{\rm{1}}}{{{{m}}_{{p}}^{{\rm{3/2}}}}}{\left(\frac{{{\rm{2}}\pi }}{{{{{T}}_{\rm{K}}}}}\right)^{{\rm{3/2}}}}\frac{{\rm{1}}}{{{{{V}}_{\rm{K}}}}}({\rm{1 + }}{\alpha _{{{np}}}}),$

(14) taking
$ N_n = N_p $ then leads to$\frac{{{{{S}}_{\rm{2}}}}}{{{{{B}}_{\rm{2}}}}}{\rm{ = }}\frac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}}}{{{{{N}}_\Lambda }{{{N}}_{{d}}}}}\Bigg/\frac{{{{{N}}_{{d}}}}}{{{{{N}}_{{p}}}{{{N}}_{{n}}}}}{{ = g}}\frac{{{\rm{1 + }}{\alpha _{\Lambda {{d}}}}}}{{{\rm{1 + }}{\alpha _{{{np}}}}}},$

(15) where
$ g = \dfrac{g_{^3_{\Lambda}H}}{g_d}\dfrac{m_p^{3/2}(m_{\Lambda}+m_d)^{3/2}}{m_{\Lambda}^{3/2}m_d^{3/2}} \approx 0.23 $ . The ratio$ S_2/B_2 $ thus carries information about the difference between$ \alpha_{np} $ and$ \alpha_{\Lambda d} $ and thus about the difference between the baryon-baryon correlation and the baryon-strangeness correlation. We note that the$ B_2 $ coalescence parameter here refers to the ratio of integrated yields, whereas in the literature it is determined differentially in momentum [55, 58-60].Similarly, we can introduce the coalescence parameter
$ B_3 = \dfrac{N_{^3{\rm He}}}{N_pN_pN_n} $ for the production of$ {\rm{^3He }}$ from the three-body coalescence of two protons and a neutron, and the coalescence parameter$ B_{s_3} = \dfrac{N_{^3_{\Lambda}{\rm H}}}{N_{\Lambda}N_pN_n} $ for the production of$ {_\Lambda ^3{\rm{H}}} $ from the three-body coalescence of$ \Lambda $ , a proton, and a neutron. Their ratio is exactly the value of$ S_3 $ , as discussed in Section 2. Since the ratio$ S_2/B_2 $ does not involve the proton density fluctuation$ \rm \Delta p $ and other mixed density fluctuation correlations, it seems a more sensitive observable than$ S_3 $ for studying the$ \Lambda $ density fluctuation.The left plot in Fig. 2 shows the results for the yield ratio
$ S_2/B_2 $ =$ N_{^3_{\Lambda}{\rm H}}/(N_{\Lambda}N_d)/(N_d/N_p^2) $ from experimental data (solid triangles) and those predicted by the statistical model (solid horizontal bars) using the proton, deuteron, and$ \rm ^3_{\Lambda}H $ yields from the full$ p_T $ range, and including the feed-down correction for the proton yield. It is seen that the measured yield ratio increases slightly with increasing collision energy, as predicted by the statistical model. We note that the value of$ B_2 $ has also been determined in experiments from the proton and deuteron momentum spectra in a small$ p_T $ window [43]. The$ S_2/B_2 $ ratio obtained from collisions at the LHC energy for momentum per constituent$ p_{T}/A = 1.4\; {\rm GeV/c} $ [8, 39, 45] is$ 0.899\pm0.171 $ . Within their uncertainties, this value is similar to that obtained using yields from the full$ p_T $ range. However, the$ S_2/B_2 $ ratios measured for different$ p_T $ bins are unavailable from experiments in the energy range available at AGS and RHIC, and this is owing to the lack of$ {_\Lambda ^3{\rm{H}}} $ $ p_T $ spectra at these energies. Future measurements of$ {_\Lambda ^3{\rm{H}}} $ spectra over a broad range of energies are needed for extracting the$ \Lambda $ and deuteron density correlation coefficient$ \alpha_{\Lambda d} $ discussed below.
Figure 2. (color online) Left: The
$ S_2/B_2$ ratio extracted from experimental data (solid triangles) and predicted by the statistical model (solid horizontal bars) for collision at the 0-10% centrality [39, 43]. Right: Values of$\alpha_{\Lambda d}$ extracted from experimental results according to Eqs. (16) and (13).The
$ \Lambda $ and deuteron correlation coefficient$ \alpha_{\Lambda d} $ can also be extracted from the yield ratio$ S_2/B_2 $ given in Eq. (15), that is${\alpha _{\Lambda {{d}}}}{\rm{ = }}\left(\frac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}}}{{{{{N}}_\Lambda }{{{N}}_{{d}}}({{{N}}_{{d}}}{{/N}}_{{p}}^{\rm{2}})}}\Bigg/{{g}}\right) \times ({\rm{1 + }}{\alpha _{{{np}}}}){\rm{ - 1}},$

(16) by taking advantage of the empirical fact that the neutron and proton density fluctuation correlation
$ \rm \alpha_{np} $ is less affected by$ T_{\rm K} $ and$ V_{\rm K} $ . Shown in the right plot of Fig. 2 by triangles are the values of$ \alpha_{\Lambda d} $ extracted from the experimental results using Eq. (16). They are seen to have similar values to those obtained from Eq. (13) using$ S_2 = \dfrac{N_{^3_\Lambda {\rm H}}}{N_\Lambda N_d} $ , which are shown by closed circles and also in Fig. 1 where it is compared with the density fluctuation correlation coefficient$ \alpha_{\Lambda p} + \alpha_{\Lambda n} $ extracted from$ S_3 = \dfrac{N_{^3_\Lambda H}/N_\Lambda}{N_{^3 {\rm He}}/N_p} $ . -
In summary, we have argued that both the ratio
$ S_2 $ and the ratio$ S_2/B_2 $ , where$ S_2 $ and$ B_2 $ are, respectively, the coalescence parameter for the production of hypertriton from$ \Lambda $ and a deuteron, and of a deuteron from a proton and a neutron, are more sensitive observables than the previously proposed ratio$ S_3 $ =$ \dfrac{{{{{N}}_{_\Lambda ^3{\rm{H}}}}/{{{N}}_\Lambda }}}{{{{{N}}_{^3{\rm{He}}}}/{{{N}}_{{p}}}}} $ for studying the local baryon-strangeness correlation in the matter produced in relativistic heavy-ion collisions. We have substantiated this argument in the framework of baryon coalescence by demonstrating that the correlation coefficient$ \alpha_{\Lambda d} $ between$ \Lambda $ and deuteron density fluctuations extracted from measured$ S_2/B_2 $ shows a stronger dependence on the energy of heavy-ion collisions than the correlation coefficients$ \alpha_{\Lambda p} + \alpha_{\Lambda n} $ between$ \Lambda $ and nucleon density fluctuations extracted from the measured$ S_3 $ . Although the results in the present study are obtained without including the feed-down contribution to nucleons from$ \Delta $ resonances, they will not be qualitatively affected because of the low kinetic freeze-out temperature of ~100 MeV, which only contribues ~20% to the nucleon yield. Experimental measurements of the ratio$ S_2/B_2 $ are expected to provide a promising way to study the strangeness and baryon correlation in the matter produced from heavy-ion collisions as the collision energy or the baryon chemical potential of produced matter is varied, which in turn can shed light on the properties of the QGP to hadronic matter phase transition during collisions.
Yield ratio of hypertriton to light nuclei in heavy-ion collisions from ${ \sqrt{{ s}_{{NN}}}}$ = 4.9 GeV to 2.76 TeV
- Received Date: 2020-04-18
- Available Online: 2020-11-01
Abstract: We argue that the difference in the yield ratio






 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF



















 DownLoad:
DownLoad: