-
Black holes are the strongest evidence of general relativity (GR). In modern physics, black holes have become one of the most controversial areas of theoretical physics. When some of the results of quantum mechanics (QM) are inserted into the framework of GR, something remarkable occurs. This approach was first proposed by Hawking in 1975 (known as Hawking radiation) [1]. However, it leads to a very acute dilemma: the information (loss) paradox [2]. QM requires that the evolution of a black hole formed in a pure state must respect the unitary principle, namely, it remains a pure state at the end of evaporation. In contrast, Hawking radiation indicates radiation in a thermal (mixed) state
1 . It was not until the Page curve was proposed that this issue gradually became clearer [3, 4]. Significant breakthroughs have been made in the last 20 years. A key catalyst was the anti-de Sitter/Conformal Field Theory (AdS/CFT), or the holographic duality [5].The AdS/CFT duality opens a window for us to look at the problem of gravity in AdS from the perspective of CFT. A milestone work is the RT formula proposed by Ryu and Takayanagi to calculate the holographic entanglement entropy [6]. The RT formula establishes the relation between the entanglement entropy of the subregion and its homologous extremal surface (RT surface) area. This was followed by the quantum correction of the RT formula [7]. In 2015, the modified RT formula with high-order corrections, the quantum extremal surface (QES) prescription, was proposed [8].
Currently, all problems of evaluating entanglement entropy at the boundary translate into finding the minimal extremal surface in bulk spacetime. After the Page time, we have another additional extremal surface, which is located inside the event horzion of the evaporating AdS black hole, called an ''island'' [9−11]. Considering its contribution led to the unitary Page curve, at which point, the black hole information paradox was declared to be preliminarily solved. Interested readers can refer to the pedagogical review in Ref. [12].
The formula for calculating the fine-grained (entanglement) entropy (or von Neumann entropy) of Hawking radiation obtained by the QES prescription is summarized as the ''island formula'':
$ \begin{aligned} S_{\text{Rad}}=\text{Min} \bigg \{ \text{Ext} \bigg[ \frac{\text{Area} (\partial I)}{4G_N} +S_{\text{bulk}} (R \cup I) \bigg] \bigg \}, \end{aligned} $

(1) where I refers to the island region, and its boundary is denoted as
$ \partial I $ . The entropy of bulk fields consists of two contributions: the island I inside the black hole and radiation region R outside the black hole. The words ''Min'' and ''Ext'' guide us to extremize the generalized entropy first to find saddle points:$ \begin{aligned} \frac{\partial S_{\text{gen}}} {\partial x^{\mu}} \equiv \frac{\partial} {\partial x^{\mu}} \bigg (\frac{\text{Area} (\partial I)}{4G_N} +S_{\text{bulk}} (R \cup I) \bigg) =0. \end{aligned} $

(2) These saddle points correspond to the candidate ''QES''. Then, we pick the one with the smallest value, which is the final correct result of the fine-grained entropy of Hawking radiation. In addition, the island formula (1) can be derived equivalently by the strict gravitational path integral [12, 13, 14]:
$ \begin{aligned} S_{\text{Rad}}= \lim\limits_{n \to 1} \frac{1}{1-n} \log \text{Tr} (\rho_R^n), \end{aligned} $

(3) where the contribution of the connected replica wormhole (saddle) dominates at late times, and the Page curves can be reproduced naturally
2 .Recently, studies have demonstrated that the island formula does not depend on the AdS/CFT correspondence and has been applied far beyond asymptotically AdS black holes. Examples include the study of islands in asymptotically flat or dS spacetime, as well as combination with some intersecting fields. One can refer to a non-exhaustive list of progress in this field [15−90].
There are two motivations for this study. To date, most studies have focused on the reproduction of Page curves in special spacetime. They all found that islands emerging at late times could curb the growth of entropy and respect unitarity [16−26, 30, 33, 34, 36, 37, 40, 41, 47−49, 54, 55, 65]. A natural question is what are the constraints on obtaining a unitary Page curve using the island paradigm for general spacetime? Equivalently, we can consider the inverse of this problem: If a Page curve already exists, namely, unitarity is maintained, what constraints do the spacetime geometry need to satisfy? Thus, the first motivation is to find the constraints on general spacetime for which the Page curve exists. Meanwhile, the quantum focusing conjecture (QFC) also has a constraint on the generalized entropy at late times. How does this constraint relate to those imposed by the island paradigm? Therefore, the second motivation is based on the QFC perspective, and we again consider the requirements on spacetime geometry. Incorporating these dual considerations, we discern that the sufficient and necessary condition for the existence of Page curves is
$f^{\prime \prime}(r_h) < \frac{6 \kappa A^{\prime}(r_h)}{cG_N}$ for the second derivative of the blacken function in the vicinity of the horizon, while and the necessary and sufficient condition for the QFC theorem to be established is$f^{\prime \prime}(r_h) < \frac{6 \kappa^2 r_h A^{\prime}(r_h) {\rm e}^{2\kappa r_{\star}(b)} }{cG_N f(b)}$ . In particular, there is a relationship$ f^{\prime \prime}(r_h)<0 $ that satisfies both of these conditions, which implies that black holes with negative heat capacities must have islands and satisfy the QFC theorem. These discoveries culminate in the formulation of overarching geometric principles.We begin with a general metric that represents a static spherically symmetric black hole. In the static coordinate system under the Schwarzschild gauge, such metrics are written as
$ (D \ge 3) $ $ \begin{aligned} {\rm d}s^2=-f(r){\rm d}t^2 +f^{-1} (r){\rm d}r^2 +r^2 {\rm d}\omega^2_{D-2}, \end{aligned} $

(4) where
${\rm d}\omega^2_{D-2}$ is the volume of the unit$ (D-2) $ -sphere, and the area of the$ (D-2) $ -sphere with radius r is equal to$ r^{D-2} \omega_{D-2} $ . Moreover, the angular direction should be removed when we focus on two-dimensional (2D) black holes. To guarantee the existence of a black hole solution, we must impose some requirements on the blacken function$ f(r) $ . It must have simple and positive zeros, and it must have a value for its corresponding radial coordinates that exceeds the horizon and extends to space-like infinity. This is the only way in which the domain of exterior communication is ''outside'' the black hole.In some special cases, the blacken functions for radial and time coordinates are not equal. In fact, this corresponds to the configuration with the Einstein-Maxwell-dilation field equation
3 . For convenience, we ignore these few special cases in this study and assume that static spherically symmetric black hole solutions can all be written in the form of (4). Moreover, when the cosmological constant Λ is non-positive, it is asymptotically associated with flat or AdS black holes, which usually have only one horizon4 . However, for a positive cosmological constant, such black holes have a cosmic horizon in addition to their event horizons. For simplicity, we focus mainly on the case of a single horizon. In the case of multiple horizons, the corresponding calculation only requires parameter substitution without affecting the physical meaning. One can refer to [63] for the explicit calculations. Besides, due to the special property of the vanishing temperature of extremal black holes [55], in this paper, we only discuss non-extremal black holes.The rest of this paper is organized as follows. In Sec. II, we calculate the entanglement entropy for Hawking radiation by the island paradigm. We first prove that the island is absent at early times. Subsequently, we focus on the behavior of entropy at late times. We derive the constraint condition that the spacetime geometry must satisfy when the island appears, and we must obtain a unitary Page curve. In Sec. III, we apply the QFC to test our results and acquire a self-consistent conclusion. Finally, we present a discussion and summary in Sec. IV. The Planck unit
$ \hbar=k_B=c=1 $ is used throughout the paper. -
In this section, we evaluate the entanglement entropy of Hawking radiation using the island formula (1). We directly assume that there is an island in black hole spacetime due to the fact that islands are necessary and sufficient to reproduce the Page curve based on the island paradigm. We investigate the behavior of generalized entropy in both the early and late stages. Consequently, we indicate that there are no islands at early times, leading to information loss. Then, we focus on the behavior at late times. Finally, we obtain a constraint equation for the spacetime geometry to ensure the appearance of Page curves.
The Penrose diagram is shown in Fig. 1
5 . To extend the metric (4) to the left and right wedges, a Kruskal transformation is allowed: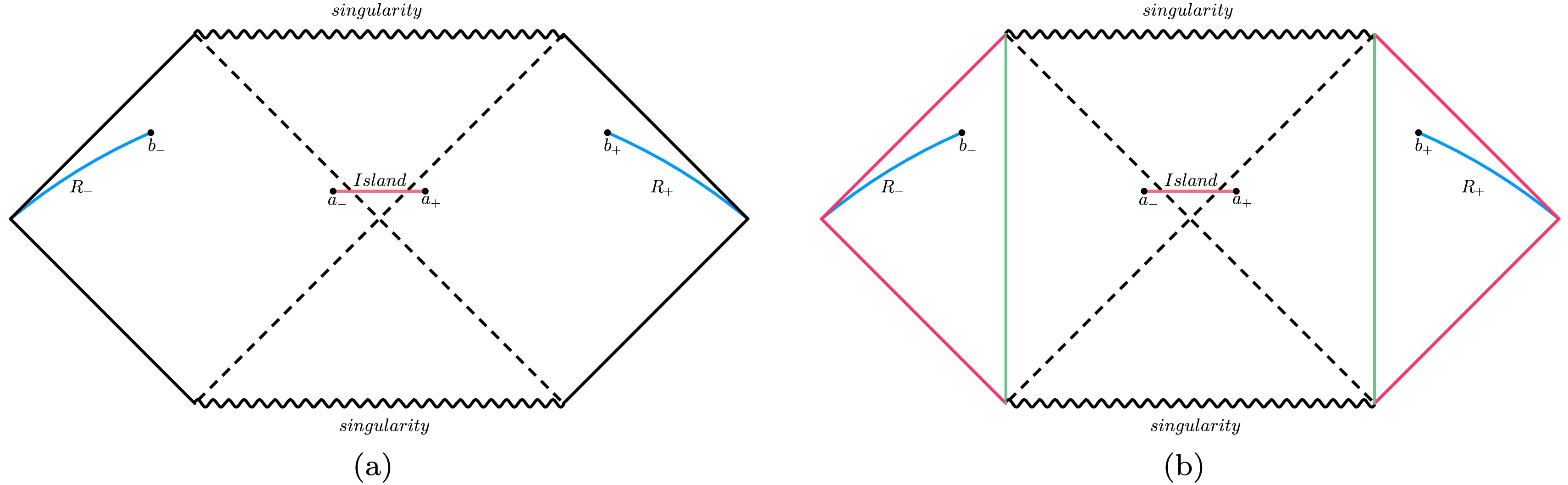
Figure 1. (color online) Penrose diagram of black holes (with a single horizon). The radiation regions are denoted by
$ R{\pm} $ , and their boundaries are the cut-off surfaces. The coordinates of boundaries of the radiation are$ b_{\pm}=(\pm t_b,b) $ . The coordinates of the islands boundaries are$ a_{\pm}=(\pm t_a,a) $ . On the left, this represents an asymptotically flat black hole. Hawking radiation can naturally diffuse to null infinity. On the right, it represents an asymptotically AdS black hole in thermal equilibrium with the bath (red region). We then impose the transparent boundary condition on the black hole region (black region) [10]. In such a way, Hawking radiation can also be collected by observers at space-like infinity.$ \begin{aligned}[b]& \text{Right wedge}: U \equiv -{\rm e}^{-\kappa u} = -{\rm e}^{-\kappa (t-r_{\star}(r))};\; V \equiv +{\rm e}^{+ \kappa v} = +{\rm e}^{+\kappa (t+r_{\star}(r))}, \\& \text{Left wedge}: U \equiv +{\rm e}^{-\kappa u} = +{\rm e}^{-\kappa (t-r_{\star}(r))}; \; V \equiv -{\rm e}^{+ \kappa v} = -{\rm e}^{+\kappa (t+r_{\star}(r))}, \end{aligned} $

(5) with the surface gravity κ:
$ \begin{aligned} \kappa \equiv 2\pi T_H = \frac{1}{2} f^{\prime}(r_h), \end{aligned} $

(6) where
$ T_H $ is the Hawking temperature,$ \prime $ represents the derivative with respect to the radial coordinate r, and$ r_h $ denotes the radius of the event horizon. Here, we set$ f(r_h)=0 $ . The tortoise coordinates are defined by$ \begin{aligned} r_{\star} (r) = \int^r \frac{1}{f(r)} {\rm d}\tilde{r}. \end{aligned} $

(7) After the Kruskal transformation, the metric (4) can be recast as
$ \begin{aligned} {\rm d}s^2=-\Omega^2(r) {\rm d}U {\rm d}V + r^2 {\rm d}\omega^2_{D-2}, \end{aligned} $

(8) with conformal factor
6 $ \begin{aligned} &\Omega_{\text{BH}}(r)= \frac{\sqrt{f(r)}}{\kappa {\rm e}^{\kappa r_{\star}(r)}}, \end{aligned} $

(9a) $ \begin{aligned} &\Omega_{\text{bath}}(r)=\frac{1}{\kappa {\rm e}^{\kappa r}}. \end{aligned} $

(9b) -
First, due to the fact that the explicit expression of the entanglement entropy is complicated in higher-dimensional case, we resort to the ''s-wave'' approximation [30]
7 . That is, we neglect the angular part of the wave function. The expression can be well approximated by the theory of two-dimensional CFT at the low energy limit. In this case, we only need to focus on the radial direction of the metric (8). In addition, we assume that the black holes is a pure state at the beginning of evaporation. Moreover, in this paper, we merely focus on the case in which the cut-off surface is distant from the black hole ($ b \gg r_h $ ) to facilitate subsequent calculations. In the case where the cut-off surface is close to the black hole, one can refer to [30, 48].In the construction of the no-island case, only radiation remains. We can only consider the complementary region of radiation based on the complementarity of von Neumann entropy. Consequently (see Appendix A),
$ \begin{aligned}[b] S_{\text{Rad}}=S(R)=\frac{c}{6} \log \Big[{\rm d}^2(b_-,b_+) \Omega(b_-) \Omega(b_+) \Big] = \left \{ \begin{array}{lr} \dfrac{c}{6} \log \bigg(\dfrac{4f(b)}{\kappa^2} \cosh^2 (\kappa t_b)\bigg), \qquad \text{for asymptotically flat black holes} &\\ \dfrac{c}{6} \log \bigg( \dfrac{4}{\kappa^2} \cosh^2(\kappa t_b) \bigg), \qquad \ \ \ \text{for asymptotically AdS black holes}& \end{array} \right. \end{aligned} $

(10) where c represents the central charge. In the limit of late times and large distances, we can take the approximation
$ \cosh(\kappa t_b) \simeq \dfrac{1}{2} {\rm e}^{\kappa t_b} $ . Then, the above equation becomes$ \begin{aligned} S_{\text{Rad}}(\text{without island}) \simeq \frac{c}{3} \kappa t_b. \end{aligned} $

(11) Apparently, without island construction, the entanglement entropy of radiation grows linearly with time at late times, which leads to information loss and is consistent with Hawking's view. In addition, the result (11) does not depend on the geometry
$ f(r) $ , which implies that the information paradox always exists.Next, we turn to the construction with an island to obtain the Page curve. Similarly, referring to the Penrose diagram in Fig. 1, we see that the entire Cauchy slice is divided into three intervals. For the disconnected union interval
$ R \cup I $ , the expression of the entanglement entropy is converted from (10) (only valid for a single interval) to the following form [92, 93]:$ \begin{aligned}[b] S_{\text{bulk}} (R \cup I) =\;&\frac{c}{3} \log \bigg( \frac{{\rm d}(a_+,a_-) {\rm d}(b_+,b_-) {\rm d}(a_+,b_+) {\rm d}(a_-,b_-)}{{\rm d}(a_+,b_-){\rm d}(a_-,b_+)} \bigg)=\frac{c}{6} \log \Big[16 \Omega^2(a) \Omega^2(b) {\rm e}^{2\kappa (r_{\star}(a)+r_{\star}(b))} \cosh^2(\kappa t_a) \cosh^2 (\kappa t_b) \Big] \\ &+\frac{c}{3} \log \bigg[ \frac{\cosh[\kappa (r_{\star}(a)-r_{\star}(b))] - \cosh[\kappa (t_a-t_b)] }{\cosh[\kappa (r_{\star}(a)-r_{\star}(b))] + \cosh[\kappa(t_a+t_b)]} \bigg], \end{aligned} $

(12) where
$ \begin{aligned} &\Omega^2(a) \Omega^2(b) = \Omega^2_{\text{BH}} (a) \Omega^2_{\text{BH}}(b)=\frac{f(a)f(b)}{\kappa^4 {\rm e}^{2\kappa (r_{\star}(a)+ r_{\star}(b))}}, \ \ \ \text{for asymptotically flat cases}, \end{aligned} $

(13a) $ \begin{aligned} &\Omega^2(a) \Omega^2(b) = \Omega^2_{\text{BH}} (a) \Omega^2_{\text{bath}}(b)=\frac{f(a)}{\kappa^4 {\rm e}^{2\kappa (r_{\star}(a)+b) }}.\qquad \text{for asymptotically AdS cases}. \end{aligned} $

(13b) Accordingly, the generalized entropy reads as
8 $ \begin{aligned}[b] S_{\text{gen}} =\;&\frac{A(a)}{2G_N} +\frac{c}{6} \log \bigg[ \frac{16 f(a)f(b)}{\kappa^4} \cosh^2(\kappa t_a) \cosh^2(\kappa t_b) \bigg] \\ &+\frac{c}{3} \log \bigg[ \frac{\cosh[\kappa (r_{\star}(a)-r_{\star}(b))] - \cosh[\kappa (t_a-t_b)] }{\cosh[\kappa (r_{\star}(a)-r_{\star}(b))] + \cosh[\kappa(t_a+t_b)]} \bigg],\end{aligned} $

(14) where
$ A(a) $ is the area of the island, which is a positive constant for$ D \ge 3 $ . Hereafter, we default to the dimension$ D \ge 3 $ to ensure that the area term$ A(r) $ is always non-negative, and we discuss the case of 2D black holes specifically in Appendix B.At very early times, we assume that
$ t_a \simeq t_b \simeq 0 \ll \kappa b $ . Then, the generalized entropy becomes$ \begin{aligned} S_{\text{gen}}^{(\text{early})} \simeq \frac{A(a)}{2G_N} +\frac{c}{6} \log \bigg[ \frac{16 f(a)f(b)}{\kappa^4} \cosh^2 (\kappa t_a) \cosh^2 (\kappa t_b) \bigg]. \end{aligned} $

(15) To obtain the QES, we extremize the above expression with respect to a and
$ t_a $ :$ \begin{aligned} \frac{\partial S_{\text{gen}}^{(\text{early})}}{\partial t_a}=\frac{c \kappa}{3} \tanh (\kappa t_a)=0. \end{aligned} $

(16) The only solution is
$ t_a=0 $ , so the approximation is correct. Then, the location of QES can be obtained by the following equation:$ \begin{aligned} \frac{\partial S_{\text{gen}}^{\text{(early)}}}{\partial a}= \frac{A^{\prime}(a)}{2G_N} +\frac{c}{6} \frac{f^{\prime} (a)}{f(a)}=0. \end{aligned} $

(17) We can rewrite this expression to obtain the constraint equation that is satisfied if the island appears at early times:
$ \begin{aligned} - \frac{3 A^{\prime}(a) f(a)}{f^{\prime}(a)} =cG_N \sim {\cal O}(G_N) \ll 1. \end{aligned} $

(18) Here, we assume that the central charge is relatively small:
$ c \sim {\cal O} (1) $ . Because the area term$ A^{\prime} (a) $ is finite and non-negative, there are two solutions that satisfy the above equation:$ \begin{aligned} &f(a) \sim 0, \qquad a \gtrsim r_h, \qquad f^{\prime}(a)<0, \end{aligned} $

(19a) $ \begin{aligned} \text{or} \ \ &f(a) \sim 0, \qquad a \lesssim r_h, \qquad f^{\prime}(a)>0 . \end{aligned} $

(19b) However, in the region
$ r \gtrsim r_h $ , the expression$ f^{\prime}(a\gtrsim r_h) $ is related to the surface gravity$ f^{\prime}(r_h)= 2 \kappa >0 $ (6). Thus, the first solution is not reasonable, while the second solution suggests that the island is located inside the event horizon. In fact, we demonstrate explicitly in Appendix C that the island cannot be inside the event horizon. Therefore, there is no physical solution for the constraint Eq. (18) at early times. We can infer that islands are absent at early times, which does not depend on the metric (4). -
By contrast, at large distances and late times, the left and right wedges are significantly separated. To simplify this, we can perform the following approximation [30]:
$ \begin{aligned}[b] &{\rm d}(a_+,a_-) \simeq {\rm d}(b_+,b_-) \simeq {\rm d}(a_+,b_-) \simeq {\rm d}(a_-,b_+) \\&\quad\gg {\rm d}(a_+,b_+) \simeq {\rm d}(a_-,b_-). \end{aligned} $

(20) Then, the entanglement entropy at late times is simplified as
$ \begin{aligned}[b] \begin{split} S_{\text{gen}}^{(\text{late})} \simeq \;&\frac{A(a)}{2G_N}+\frac{c}{3} \log [{\rm d}(a_+,b_+){\rm d}(a_-,b_-)] \\ =\;&\frac{A(a)}{2G_N} +\frac{c}{6} \log \bigg\{ \frac{4f(a)f(b)}{\kappa^4} \\\times &\bigg[ \cosh[\kappa (r_{\star}(a)-r_{\star}(b))]-\cosh[\kappa(t_a-t_b)] \bigg]^2 \bigg\}. \end{split} \end{aligned} $

(21) In same way, we extremize this with respect to time
$ t_a $ :$ \begin{aligned} \frac{\partial S_{\text{gen}}^{\text{(late)}} }{\partial t_a}= -\frac{c}{3} \frac{\kappa \sinh[\kappa (t_a-t_b)]}{\cosh[\kappa (r_{\star}(a) -r_{\star}(b))] - \cosh[\kappa (t_a-t_b)]} =0. \end{aligned} $

(22) The only solution is to set
$ t_a $ equal to$ t_b $ and then substitute this relation into the original expression and extremize it with respect to a:$ \begin{aligned}[b] \frac{\partial S_{\text{gen}}^{(\text{late})} }{\partial a} & = \frac{A^{\prime} (a)}{2G_N} +\frac{c}{6} \bigg[ \frac{f^{\prime}(a)}{f(a)} + \frac{2\kappa}{f(a)} \coth \Big[\frac{\kappa}{2}(r_{\star}(a) -r_{\star}(b))\Big] \bigg]=0, \\ &=\frac{A^{\prime}(a)}{2G_N} + \frac{c f^{\prime}(a)}{6f(a)} -\frac{c \kappa}{3 f(a)} \bigg( 1+\frac{2}{e^{\kappa x}-1} \bigg)=0, \end{aligned} $

(23) where we use
$ r_{\star}^{\prime}(a)=\dfrac{1}{f(a)} $ and set$ x\equiv r_{\star}(b) - r_{\star}(a) $ to simplify the equation. Here, we assume that the location of the cutoff surface ($ r=b $ ) has the same order as that of the island ($ r=a $ ), i.e., x is sufficiently large ($ e^{\kappa x}-1 \gg 0 $ ). Thus, the last term in the second line of the above equation does not become a large negative number and causes Eq. (23) to have no solution9 . Following (18), we rewrite this expression as$ \begin{aligned} \frac{3 A^{\prime}(a) f(a) }{4\kappa {\rm e}^{-\kappa x} +2\kappa -f^{\prime}(a) } =cG_N \ll 1, \end{aligned} $

(24) Now, we make the near horizon limit
$ a\simeq r_h $ and obtain$ \begin{aligned}[b] f(r) &\simeq f^{\prime}(r_h)(r-r_h) + {\cal O} [(r-r_h)^2]\\&=2 \kappa (r-r_h) + {\cal O} [(r-r_h)^2], \end{aligned} $

(25a) $ \begin{aligned} f^{\prime}(r)\simeq f^{\prime}(r_h) +f^{\prime \prime}(r_h) (r-r_h) + {\cal O} [(r-r_h)^2], \end{aligned} $

(25b) $ \begin{aligned} r_{\star}(r)=\int^r \frac{{\rm d} \tilde{r}}{f(r)} \simeq \frac{1}{2\kappa} \log \frac{|r-r_h|}{r_h} . \end{aligned} $

(25c) Substituting these equations into (24) yields the following constraint equation:
$ \begin{aligned} 0<y(a) = \frac{6 \kappa A^{\prime}(a) (a-r_h)}{4\kappa {\rm e}^{-\kappa r_{\star}(b)} \sqrt{\dfrac{(a-r_h)}{r_h}} - f^{\prime \prime}(r_h)(a-r_h) } =cG_N \ll 1. \end{aligned} $

(26) First, we know that the necessary and sufficient condition for the existence of islands is that Eq. (26) has a solution. Based on the non-negativity of the area term
$ A^{\prime}(a) $ (for$ D \ge 3 $ ), we obtain the constraint equation$ \begin{aligned} a>r_h, \qquad f^{\prime \prime}(r_h) < \frac{4 \kappa {\rm e}^{-\kappa r_{\star}(b)}}{ \sqrt{(a-r_h) r_h}}, \end{aligned} $

(27a) $ \begin{aligned} a<r_h, \qquad f^{\prime \prime}(r_h) < \frac{-4 \kappa {\rm e}^{-\kappa r_{\star}(b)}}{ \sqrt{(r_h-a) r_h}}. \end{aligned} $

(27b) These two solutions correspond to the island being located outside and inside the event horizon, respectively. However, we show in Appendix B that islands cannot be inside the event horizon, so the second solution (27b) should be discarded. Next, when the above condition is satisfied, there must be an island located outside the event horizon:
$ \begin{aligned} a\simeq r_h+ \frac{4c^2 G_N^2 {\rm e}^{-2\kappa r_{\star}(b) }}{9r_h (A^{\prime}(r_h))^2} +{\cal O} [(cG_N)^3]. \end{aligned} $

(28) Substituting this location into the constraint Eq. (27a), we obtain the necessary and sufficient condition for the existence of the island:
$ \begin{aligned} f^{\prime \prime}(r_h) < \frac{6 \kappa A^{\prime}(r_h)}{cG_N} \equiv \tilde{\alpha}. \end{aligned} $

(29) At first sight, one might naively assume that the result of Eq. (29) is trivial because, in the semiclassical frame, if the Newton constant
$ G_N $ is sufficiently small, then the constraint (29) is easily satisfied. On the one hand, we now pay attention to the behavior of entropy at late times (21). At this time, the black hole is at the end of evaporation. The quantum effect dominates and should not be ignored. On the other hand, even at the early stage, the result (29) is trivial only for non-extremal black holes with high temperature ($ T_H\sim \kappa \gg 1 $ ). However, for a near extremal black hole with almost vanishing temperature ($ T_H \sim 0 $ ), this constraint must be treated with great caution. Therefore, the constraint in Eq. (29) is a significant conclusion. Finally, according to the location (28), we obtain the entanglement entropy of radiation at late times as follows:$ \begin{aligned} \begin{split} S_{\text{Rad}}(\text{with island}) & \simeq \frac{A(r_h)}{2G_N} +{\cal O} (G_N) \simeq 2S_{\text{BH}}. \end{split} \end{aligned} $

(30) This is in line with our expectations. Recalling the result without an island (11), the Page time is determined by
$ \begin{aligned} t_{\text{Page}}=\frac{6S_{\text{BH}}}{c \kappa} =\frac{3 S_{\text{BH}}}{c \pi T_H}. \end{aligned} $

(31) Besides, we can calculate the scrambling time as a by-product. Drawing from the insights of the Hayden-Preskill thought experiment [94], it is posited that an external observer, situated asymptotically relative to the black hole, must patiently await the elapsed duration known as the ''scrambling time'' before information initially engulfed by the black hole can be retrieved by analyzing the emitted Hawking radiation. In the language of the entanglement wedge reconstruction, the scrambling time corresponds to the time when the information reaches the boundary of the island (
$ r=a $ ) from the cut-off surface ($ r=b $ ) [11]:$ \begin{aligned}[b] t_{\text{scr}} &\equiv \text{Min} [v(t_b,b)-v(t_a,a)] =r_{\star}(b) -r_{\star}(a) \\ &\simeq r_{\star}(b) - \frac{1}{2 \kappa} \log \frac{a-r_h}{r_h} \simeq \frac{1}{2\kappa} \log \frac{A^{\prime}(r_h)r_h}{cG_N} \\ &\simeq \frac{1}{2\pi T_H} \log S_{\text{BH}}, \end{aligned} $

(32) where
$ t_a, t_b $ are the times of sending and receiving information, respectively. In the penultimate line, we employed the approximation delineated in Eq. (25c) to facilitate our calculations and assumed that b has the same order as that of the event horizon$ b \sim r_h $ . Therefore, we adopted the established outcome for the four-dimensional scenario, aligning seamlessly with the findings reported in the seminal Hayden-Preskill thought experiment [95, 96], thus ensuring theoretical consistency.Above all, we protect the unitarity by the island formula. In particular, we obtain a sufficient and necessary condition for deriving the Page curve (29). In addition, because the area term
$ A^{\prime}(r_h) $ is non-negative, the critical value$ \tilde{\alpha} $ is always positive. Therefore, we can further infer that there must exist a Page curve when the following constraint is satisfied, i.e., a sufficient and unnecessary condition for Page curves:$ \begin{aligned} f^{\prime \prime}(r_h +{\cal O} (G_N))<0. \end{aligned} $

(33) Specifically, the radial coordinate r is confined to a region situated just outside the event horizon, adhering to the condition
$ r \gtrsim r_h $ , reflecting our focus on the immediate vicinity of the horizon through implementation of the near-horizon approximation. The impact of this condition on the results is discussed in detail in the following section. -
Up to now, we calculatec the Page curve using the island formula (1). Combing the results of the previous section, we obtain the behavior of entanglement entropy in the entire process of black hole evaporation as
$ \begin{aligned} S_{\text{Rad}}=\text{Min} \Bigg[\frac{2 \pi c}{3} T_H t, 2S_{\text{BH}}\Bigg]. \end{aligned} $

(34) In particular, we find that if the constraint condition (29) is satisfied, the Page curve must be reproduced, and there must exist an island outside the event horizon (28). This conclusion is universal and does not depend on the explicit form of the metric (4). In this sense, we provided the constraint conditions of spacetime when the Page curve is established.
In this section, we further study the constraint of Page curves on space-time from the perspective of QFC and compare the results with (33), given by the island paradigm. The classical focusing theorem asserts that the expansion θ of the congruence of the null geodesic never increases:
$ \begin{aligned} \frac{{\rm d}\theta}{{\rm d} \lambda} \le 0, \end{aligned} $

(35) where λ is the affine parameter. An important application of this theorem is to prove the second law of black holes. For a black hole with area A and entropy
$ S_{\text{BH}} $ ($ =\dfrac{A}{4G_N} $ ), the expansion θ is defined by$ \begin{aligned} \theta=\frac{1}{A} \frac{{\rm d}A}{{\rm d}\lambda}. \end{aligned} $

(36) Then, one can infer the second law,
$ {\rm d}S_{\text{BH}} \ge0 $ .However, once quantum effects are considered
10 , i.e., the black hole emits Hawking radiation, the second law is violated. For the sake of rationality, this law should be upgraded to the generalized second law. Accordingly, black hole entropy should be replaced with generalized entropy:${\rm d}S_{\text{gen}} \ge0$ . Therefore, the classical focusing theorem is extended to the QFC [97, 98], where the quantum expansion is given by replacing the area in the classical expansion with the generalized entropy (21):$ \begin{aligned} \frac{{\rm d} \Theta}{{\rm d} \lambda} \le 0, \end{aligned} $

(37) where Θ is the quantum expansion, which can be expressed in terms of generalized entropy:
$ \begin{aligned} \Theta = \frac{1}{A} \frac{\rm d}{{\rm d}\lambda} S_{\text{gen}}. \end{aligned} $

(38) Now, we investigate the QFC for the construction with an island. For the entanglement entropy at late times (21), the quantum expansion is written as
$ \begin{aligned}[b] \Theta=\;&\frac{1}{A} \frac{\rm d}{{\rm d} \lambda} S_{\text{gen}}\\=\;&\frac{1}{A} \frac{{\rm d}v_b}{{\rm d}\lambda} \bigg[ \frac{\partial S_{\text{gen}}}{\partial v_b} +\frac{{\rm d}v_a}{{\rm d}v_b} \frac{\partial S_{\text{gen}}}{ \partial v_a} +\frac{{\rm d}u_a}{{\rm d}v_b} \frac{\partial S_{\text{gen}}}{\partial u_a} \bigg]. \end{aligned} $

(39) Here, we introduce the affine parameter [98]
$ \begin{aligned} {\rm d}\lambda \equiv - \frac{\partial r(u,v)}{\partial u} {\rm d}v, \end{aligned} $

(40) for simplicity. Because QES makes the entanglement entropy extremized, i.e.,
$ \begin{aligned} \frac{\partial S_{\text{gen}}}{\partial u_a}=\frac{\partial S_{\text{gen}}}{\partial v_a}=0, \end{aligned} $

(41) we have
$ \begin{aligned}[b] \Theta=\;&\frac{1}{A} \frac{{\rm d}v_b}{{\rm d} \lambda} \frac{\partial S_{\text{gen}}}{\partial v_b} = \frac{1}{A(b)f(b)} \bigg[\frac{\partial S_{\text{gen}}}{\partial t_b} +f(b) \frac{\partial S_{\text{gen}}}{\partial b}\bigg] \\ =\;&\frac{ A^{\prime}(b)}{2A(b)G_N} - \frac{c \kappa }{3 A(b) f(b)} \coth \\&\times\bigg[\frac{\kappa}{2}((t_a-t_b)+(r_{\star}(a) - r_{\star}(b))\bigg] + \frac{c }{6 A(b) f(b)} f^{\prime}(b) \\ =\;&\frac{A^{\prime}(b)}{2A(b) G_N} +\frac{c}{6 A(b)f(b)} f^{\prime}(b) \\&+ \frac{c \kappa }{3 A(b) f(b)} \bigg( 1+ \frac{1}{{\rm e}^{\kappa(r_{\star}(b)-r_{\star}(a))}-1} \bigg) >0. \end{aligned} $

(42) Here, we have used the relation
$ t_a=t_b $ , because the cut-off surface is far from the event horizon,$ f(b>r_h \simeq a)>0 $ . Therefore, the entanglement entropy is always increasing with the null time$ v_b $ , and the quantum expansion is positive. Moreover, following the QFC, we obtain the derivative of the quantum expansion as$ \begin{aligned}[b] \frac{{\rm d}\Theta}{{\rm d}\lambda} &= \frac{\rm d}{{\rm d}\lambda} \bigg(\frac{1}{A} \frac{{\rm d}S_{\text{gen}}}{{\rm d}\lambda} \bigg)=\frac{1}{{\rm d}\lambda} \bigg( \frac{1}{A} \frac{{\rm d}v_b}{{\rm d}\lambda} \frac{\partial S_{\text{gen}}}{\partial v_b} \bigg)= \frac{1}{f(b)} \frac{\rm d}{{\rm d}v_b} X \\ &= - \frac{[A^{\prime}(b)]^2-A(b) A^{\prime \prime}(b)}{2G_N A^2(b)} - \frac{c}{6A^2(b) f^2(b)} (Y+Z), \end{aligned} $

(43) where
$ \begin{aligned}[b] X=\;& \frac{A^{\prime}(b)}{A(b)G_N} +\frac{c \kappa}{3A(b) f(b)} \coth \bigg(\frac{\kappa}{2}\Big(r_{\star}(b) - r_{\star}(a)\Big)\bigg)\\& +\frac{c}{6A(b)} \frac{f^{\prime}(b)}{f(b)}, \end{aligned} $

(44a) $ \begin{aligned} Y=f(b) A^{\prime}(b) \Big[ 2\kappa \coth \bigg(\frac{\kappa}{2}\Big(r_{\star}(b) - r_{\star}(a)\Big)\bigg) +f^{\prime}(b) \Big] >0, \end{aligned} $

(44b) $ \begin{aligned}[b] Z=\;& A(b) \Bigg[ 4\kappa^2 \frac{{\rm e}^{\kappa (r_{\star}(a) - r_{\star}(b))}}{\big({\rm e}^{\kappa (r_{\star}(a)-r_{\star}(b))} -1 \big)^2} \\&+\frac{2\kappa f^{\prime}(b) \big( {\rm e}^{\kappa r_{\star}(b)}+{\rm e}^{\kappa r_{\star}(a) }\big)}{{\rm e}^{\kappa r_{\star}(b)}-{\rm e}^{\kappa r_{\star}(a)}} + (f^{\prime}(b))^2 -f(b) f^{\prime \prime}(b) \Bigg]. \end{aligned} $

(44c) In the above calculations, the QES condition (41) is used to simplify the second line of the expression (43) to eliminate terms related to
$ u_A $ and$ v_a $ . The first term of Eq. (43) is related to the area, which is always positive for spherically symmetric black holes because the area term is a linear function of the radius r. Therefore, the only requirement that the QFC theorem must satisfy is non-vanishing Z. Further, because the location of the cutoff surface$ (r=b) $ is artificially selected, if we assume that it has the same order as that of the horizon,$ b \sim r_h \simeq a $ , then the expression Z can be reduced to the following form:$ \begin{aligned} \begin{split} Z & \sim A(r_h) \bigg( \frac{4 \kappa^2}{{\rm e}^{\kappa (r_{\star}(a)- r_{\star}(b))}} + 2\kappa f^{\prime}(r_h) + (f^{\prime}(r_h))^2 -f(b) f^{\prime \prime}(r_h) \bigg) \\ & = A(r_h) \bigg (4 \kappa^2\bigg( \frac{1}{{\rm e}^{\kappa (r_{\star}(a)- r_{\star}(b))}} +2 \bigg) -f(b)f^{\prime \prime}(r_h) \bigg) \\ & \simeq A(r_h) \Bigg( \frac{4 \kappa^2 {\rm e}^{\kappa r_{\star}(b)}}{\sqrt{\dfrac{a-r_h}{r_h}}} -f(b)f^{\prime \prime} (r_h) \Bigg)>0. \end{split} \end{aligned} $

(45) In the first line, we have used
$ {\rm e}^{\kappa r_{\star}(b)} \gg {\rm e}^{\kappa r_{\star}(a)} \gg1 $ and$ b \sim r_h $ . In the last line, we do not expand$ f(b) $ because, although the cut-off surface has the same order as that of the horizon$ (b \sim r_h) $ , its gravitational effect is so small (the asymptotic region of the observer) that it cannot be included in the near-horizon region$ (r \simeq r_h) $ . Therefore, we can determine that the sufficient and necessary conditions for QFC are valid based on the location of the island expressed in Eq. (28):$ \begin{aligned} f^{\prime \prime}(r_h) < \frac{6 \kappa^2 r_h A^{\prime}(r_h) {\rm e}^{2\kappa r_{\star}(b)} }{cG_N f(b)} \equiv \tilde{\beta} = \tilde{\alpha} \cdot \frac{\kappa r_h {\rm e}^{2\kappa r_{\star}(b)}}{f(b) }. \end{aligned} $

(46) The explanation of the physical significance here is consistent with that given below Eq. (29), and this constraint is also a non-trivial result. In particular, based on the non-negativity of
$ \tilde{\beta} $ , we also obtain a sufficient and unnecessary condition for QFC to hold:$ \begin{aligned} f^{\prime \prime}(r_h)<0. \end{aligned} $

(47) Comparing this constraint and the result from the island paradigm (33), we find that the derived QFC result (47) contains (33). Namely, the applicability of QFC is wider. Moreover, both are a sufficient and unnecessary condition for the Page curve and QFC to be established. Therefore, we can conclude that a sufficient and unnecessary condition for a Page curve for general spacetime (4) to exist and satisfy QFC is that the second derivative of the blacken function is negative in the near horizon region. We stress that this conclusion is only valid at the semi-classical level, where the whole spacetime is can be regards as static.
Now, we display some physical meaning of the results. As we know, the first derivative of the blacken function
$ f(r) $ at the event horizon$ r_h $ is the Hawking temperature$ T_H = \dfrac{f^{\prime} (r_h)}{4\pi} $ of the black hole. Therefore, its second derivative is related to the heat capacity:$ \begin{aligned}[b] C_H &\equiv T_H \bigg( \frac{\partial S_{BH}}{\partial T_H} \bigg) = T_H \bigg( \frac{\partial S_{BH} / \partial r_h}{\partial T_H / \partial r_h} \bigg) \\ & \sim f^{\prime}(r_h) \cdot \frac{A^{\prime}(r_h)}{f^{\prime \prime} (r_h)}. \end{aligned} $

(48) Then, the positive or negative heat capacity is consistent with
$ f^{\prime \prime} (r_h) $ . Namely, when the condition (33) is satisfied, the capacity is always negative. Therefore,$ f^{\prime \prime}(r_h)<0 $ is necessary and sufficient for the heat capacity of a black hole to be negative. Then, we can further summarize secondary conclusions: a black hole with a negative heat capacity must have islands at late times. Moreover, this is also supported by the QFC. We present the results of calculations for some typical black holes in Table 1.Black Hole $ f(r) $ 

$ f^{\prime \prime}(r_h) $ 

$ \tilde{\alpha} $ 

$ \tilde{\beta} $ 

Location of Islands/QFC Callan-Giddings-Harvey-Strominger [18, 19] $ 1-{\rm e}^{-2 \lambda (r-r_h)} $ 

$ \begin{array}{c}-4\lambda^2 \\ \sim - {\cal O}\bigg(\frac{1}{r_h^2}\bigg) \end{array}$ 

$\begin{array}{c} \frac{12\lambda^2 {\rm e}^{2\lambda r_h}}{cG_N} \\ \sim {\cal O} \bigg( \frac{1}{r_h^2 G_N}\bigg) \end{array}$ 

$\begin{array}{c} \frac{12\lambda^3 r_h {\rm e}^{\lambda (2r_h +r_{\star}(b))}}{cG_N(1-{\rm e}^{-2\lambda(b-r_h))}} \\ \sim {\cal O} \bigg( \frac{1}{r_h^2 G_N}\bigg) \end{array}$ 

$\begin{array}{c} r_h+ \frac{c^2G_N^2}{12\lambda {\rm e}^{2\lambda r_h}} {\rm e}^{\lambda r_{\star}(b)} \\ \text{QFC is satisified} \end{array}$ 

Jackiw-Teitelboim [24] $ \frac{r^2 -r_h^2}{\ell^2} $ 

$\begin{array}{c} \frac{2}{\ell^2} \\ \sim {\cal O}\bigg(\frac{1}{r_h^2}\bigg) \end{array}$ 

$\begin{array}{c} \frac{6r_h}{cG_N \ell^3} \\ \sim {\cal O} \bigg(\frac{1}{r_h^2 G_N}\bigg) \end{array}$ 

$\begin{array}{c} \frac{6r_h^3 {\rm e}^{\frac{r_h r_{\star}(b)}{\ell^2}}}{cG_N(b^2-r_h^2) \ell^3} \\ \sim {\cal O} \bigg(\frac{1}{r_h^2 G_N}\bigg) \end{array}$ 

$\begin{array}{c} r_h+ \frac{c^2G_N^2 \ell}{6}{\rm e}^{\frac{r_h}{\ell^2} r_{\star}(b)}\\ \text{QFC is satisified} \end{array}$ 

Bañados-Teiteboim-Zanelli [79] $ \frac{r^2 -r_h^2}{\ell^2} $ 

$\begin{array}{c} \frac{2}{\ell^2} \\ \sim {\cal O} \bigg(\frac{1}{r_h^2}\bigg) \end{array}$ 

$\begin{array}{c} \frac{12\pi r_h}{cG_N \ell^2} \\ \sim {\cal O}\bigg(\frac{1}{r_h G_N}\bigg) \end{array}$ 

$\begin{array}{c} \frac{12 \pi r_h^3 {\rm e}^{- \frac{r_h r_{\star}(b)}{\ell^3}}}{cG_N (b^2-r_h^2)\ell^2} \\ \sim {\cal O}\bigg(\frac{1}{r_h G_N}\bigg) \end{array}$ 

$\begin{array}{c} r_h+ \frac{c^2G_N^2}{12\pi} {\rm e}^{\frac{r_h}{\ell^2} r_{\star}(b)} \\ \text{QFC is satisfied} \end{array}$ 

Rotating Bañados-Teiteboim-Zanelli [47] $ \frac{(r^2-r_h^2)(r^2-r_-^2)}{r^2 \ell^2} $ 

$\begin{array}{c} \frac{2(3r_-^2+r_h^2)}{r_h^2 \ell^2} \\ \sim {\cal O}\bigg(\frac{1}{r_h^2}\bigg) \end{array}$ 

$\begin{array}{c} \frac{12\pi (r_h^2-r_-^2)}{cG_N r_h \ell^2} \\ \sim {\cal O}\bigg(\frac{1}{r_h G_N}\bigg) \end{array}$ 

$\begin{array}{c} \frac{12\pi (r_h^2-r_-^2)^2b^2 {\rm e}^{\frac{(r_h^2-r_-^2)r_{\star}(b)}{r_h \ell^2}}}{cG_N r_h \ell^2 (b^2 -r_h^2)(b^2-r_-^2)} \\ \sim {\cal O}\bigg(\frac{1}{r_h G_N}\bigg) \end{array}$ 

$\begin{array}{c} r_h+\frac{c^2 G_N^2}{18\pi^2 r_h} {\rm e}^{2 \big(\frac{r_h^2 -r_-^2}{r_h \ell^2}\big) r_{\star}(b)}\\ \text{QFC is satisfied} \end{array}$ 

Schwarzschild [30, 48] $ 1-\frac{r_h}{r} $ 

$\begin{array}{c} -\frac{2}{r_h^2} \\ \sim -{\cal O} \bigg(\frac{1}{r_h^2}\bigg) \end{array}$ 

$\begin{array}{c} \frac{24\pi}{cG_N} \\ \sim {\cal O}\bigg(\frac{1}{G_N}\bigg) \end{array}$ 

$\begin{array}{c} \frac{12 \pi b {\rm e}^{\frac{r_{\star}(b)}{2r_h}}}{cG_N(b-r_h)} \\ \sim {\cal O}\bigg(\frac{1}{G_N}\bigg) \end{array}$ 

$\begin{array}{c} r_h+ \frac{c^2G_N^2}{48\pi r_h} {\rm e}^{\frac{r_{\star}(b)}{2r_h}} \\ \text{QFC is satisfied bn} \end{array}$ 

Schwarzschild-AdS [28] $ 1-\frac{r_0}{r}+\frac{r^2}{\ell^2} $ 

$\begin{array}{c} \frac{2}{\ell^2}-\frac{2r_0}{r_h^2} \\ \sim {\cal O}\bigg(\frac{1}{r_h^2}\bigg) \end{array}$ 

$\begin{array}{c} \frac{24\pi r_h \big(\frac{2r_h}{\ell^2}+\frac{r_0}{r_h^2} \big)}{cG_N} \\ \sim {\cal O}\bigg(\frac{1}{G_N}\bigg) \end{array}$ 

$\begin{array}{c} \frac{12 \pi r_h^2\big(\frac{2r_h}{\ell^2}+\frac{r_0}{r_h^2} \big)^2 }{cG_N \big(1+\frac{b^2}{\ell^2}-\frac{r_0}{b} \big)} \times \\ \text{exp}\bigg(\big(\frac{r_h}{\ell^2}+\frac{r_0}{2r_h^2}\big)r_{\star}(b) \bigg) \\ \sim {\cal O}\bigg(\frac{1}{G_N}\bigg) \end{array}$ 

$\begin{array}{c} r_h+ \frac{c^2G_N^2}{48 \pi r_h} {\rm e}^{\big(\frac{r_h}{\ell^2}+\frac{r_0}{2r_h^2}\big) r_{\star}(b)} \\ \text{QFC is satisified} \end{array}$ 

Schwarzschild-dS [77] $ \frac{(r_U-r)(r-r_h)(r+r_h+r_U)}{\ell^2 r} $ 

$ \begin{array}{c}-\frac{2(r_h^2+r_h r_U+r_U^2)}{\ell^2 r_h^2} \\ \sim -{\cal O}\bigg(\frac{1}{r_h^2}\bigg) \end{array}$ 

$\begin{array}{c} \frac{24\pi (r_U-r_h)(2r_h+r_U)}{cG_N \ell^2} \\ \sim {\cal O}\bigg(\frac{1}{G_N}\bigg) \end{array}$ 

$\begin{array}{c} \frac{12 \pi b(r_h-r_U)^2(2r_h+r_U)^2}{cG_N (b-r_h)(r_U-b)(b+r_h+r_U) \ell^2} \times \\ \text{exp}\big(\frac{(r_U-r_h)(2r_h+r_U)r_{\star}(b)}{2r_h \ell^2}\big) \\ \sim {\cal O}\bigg(\frac{1}{G_N}\bigg) \end{array}$ 

$\begin{array}{c} r_h+\frac{c^2G_N^2 {\rm e}^{\frac{(r_c-r_h)(r_h-r_u)}{6r_h\ell^2}}}{48 \pi r_h {\rm e}^{-r_{\star}(b)}} \\ \text{QFC is satisified} \end{array}$ 

Reissner-Nordstr $ \ddot{\text{o}} $ m [54]

$ \big(1-\frac{r_h}{r} \big) \big(1-\frac{r_-}{r} \big) $ 

$\begin{array}{c} \frac{4r_-2r_h}{r_h^3} \\ \sim {\cal O}\bigg(\frac{1}{r_h^2}\bigg) \end{array}$ 

$\begin{array}{c} \frac{24\pi \big(1-\frac{r_-}{r_h} \big)}{cG_N} \\ \sim {\cal O}\bigg(\frac{1}{G_N}\bigg) \end{array}$ 

$\begin{array}{c} \frac{12 \pi b^2(r_h-r_-)^2}{cG_N(b-r_-)(b-r_h)r_h^2} \times \\ \text{exp} \bigg( \frac{(r_h-r_-)r_{\star}(b)}{2r_h^2} \bigg) \\ \sim {\cal O}\bigg(\frac{1}{G_N}\bigg) \end{array}$ 

$\begin{array}{c} r_h+\frac{c^2G_N^2}{48 \pi r_h} {\rm e}^{\big(\frac{r_h-r_-}{2r_h^2}\big) r_{\star}(b)} \\ \text{QFC is satisified} \end{array}$ 

Reissner-Nordström-AdS [85, 90] $\begin{array}{c} \frac{(r-r_-)(r-r_h)}{\ell^2 r} \times \\ \big(\ell^2+r^2+r_-^2+r_h^2+r_hr_-\big) \end{array}$ 

$\begin{array}{c} \frac{2(r_-^3+r_-^2r_h+2r_h^3)}{r_h^2 \ell^2} +\\ \frac{2r_- \ell}{r_h^2 \ell^2}\\ \sim {\cal O}\bigg(\frac{1}{r_h}\bigg) \end{array}$ 

$\begin{array}{c} \frac{24\pi (r_h-r_-)}{cG_N \ell^2} \times \\ \Big(r_-^2+r_-r_h+ 2r_h^2+\ell^2 \Big) \\ \sim {\cal O}\bigg(\frac{r_h}{G_N}\bigg) \end{array}$ 

$\begin{array}{c} \frac{12 \pi b((r_-^2+r_- r_h +2r_h^2+\ell^2)^2}{cG_N(b-r_-)(b-r_h) \ell^2 } \times \\ \frac{r_h-r_-)^2}{(b^2+r_-^2+r_hr_- + r_h^2+\ell^2)} \times \\ \text{exp} \bigg( \frac{(r_h-r_-)r_{\star}(b)}{2r_h\ell^2} \bigg) \\ \sim {\cal O}\bigg(\frac{r_h}{G_N}\bigg) \end{array}$ 

$\begin{array}{c} r_h+\frac{c^2 G_N^2}{144\pi r_h^3} {\rm e}^{r_{\star}(b)} \times \\ {\rm e}^{\big(\frac{(3r_h^2+2r_hr_-+r_-^2)(r_h-r_-)}{r_h \ell^2}\big)}\\ \text{QFC is satisfied} \end{array}$ 

Higher-dimensional Schwarzschild [30] $ 1-\big(\frac{r_h}{r}\big)^{d-3} $ 

$\begin{array}{c} -\frac{6-5d+d^2}{r_h^2}\\ \sim {-\cal O}\bigg(\frac{1}{r_h^2}\bigg) \end{array}$ 

$\begin{array}{c} \frac{3(d-3)(d-2)r_h^{d-4} \omega_{d-2}}{cG_N}\\ \sim {\cal O} \bigg(\frac{r_h^{d-4}}{G_N}\bigg) \end{array}$ 

$\begin{array}{c} \frac{3(d-3)^2(d-2)r_h^{d-4} \omega_{d-2}}{2cG_N \bigg( 1- \bigg( \frac{r_h}{b} \bigg)^{d-3} \bigg)} \times\\ \text{exp} \bigg( \frac{(d-3)r_{\star}(b)}{2r_h} \bigg)\\ \sim {\cal O}\bigg( \frac{r_h^{d-4}}{G_N} \bigg) \end{array}$ 

$\begin{array}{c} r_h +\frac{cG_N {\rm e}^{\frac{d-3}{2r_h}} r_{\star}(b) }{12\omega_{d-2} r_h^{d-3}}\\ \text{QFC is satisfied} \end{array}$ 

Liouville [22] $ 1-{\rm e}^{-2\sqrt{|C|}r} $ 

$\begin{array}{c} -4|C|\\ \sim -{\cal O}\bigg(\frac{1}{r_h^2}\bigg) \end{array}$ 

$ \begin{array}{c} -\frac{96 |C|}{ cG_N}\\ \sim -{\cal O}\bigg(\frac{1}{r_h^2G_N}\bigg) \end{array}$ 

$ 0 $ 

No Island
QFC is satisfiedTable 1. Related results for several black holes. Here, we assume that the the cosmological constant Λ, AdS length
$ \ell $ , and horizon$ r_h,r_-,r_U $ have the same order. The Newton constant is sufficiently small:$ cG_N \sim {\cal O}(G_N) \ll 1 $ . Thus,$ \tilde{\alpha} \sim \tilde{\beta} \gg f^{\prime \prime}(r_h) $ in most cases. In particular, for a Liouville black hole [22], there is no island because of its blacken factor not satisfying the relation$ f^{\prime \prime}(r_h)< \tilde{\alpha} $ . We discuss this special 2D black hole in detail in Appendix B. -
In summary, we calculated the Page curve from the general static spherical symmetry metric (4) and obtained the entanglement entropy of radiation, behaving as expressed in (34). We found that the island is always outside the event horizon (28). We also obtained a sufficient and necessary condition (29) for the emergence of islands. This methodology sets a benchmark for employing the island paradigm in Page curve computations. In particular, we used the Liouville black hole [22] as an example to support our conclusion. In addition, we emphasize that this conclusion is valid only under the semi-classical approximation, namely, the metric is static and the approximation is valid (25). When the size of the black hole that evaporates to the final stage is sufficiently small, the quantum effect cannot be ignored, and the near-horizon approximation (25) may also fail due to the effect of high curvature at the event horizon of black holes at the final stage. Meanwhile, we followed the perspective of QFC to prove our results. Explicit calculations indicate that the QFC is always satisfied when condition (46) is present, which also ensures the validity of behavior of the entanglement entropy at late times (21). In particular, we found that a common constraint equation that satisfies conditions (29) and (46) is the condition expressed via (33) and (47). These are both self-consistent, which implies the rationality of our calculation.
Therefore, we considered the inverse problem of calculating Page curves and concluded the following. When the constraint expressed in Eq. (29) is satisfied, one can always obtain the unitary Page curve from the generic metric (4). Meanwhile, the QFC will always hold under the constraint (46). Our study significantly contributes to the comprehension of black hole evaporation dynamics and the resolution of the information paradox by leveraging insights from the island paradigm and QFC. Finally, the common constraint of spacetime (47) affirms the universality of Page curves, transcending model-specific restrictions and reinforcing the compatibility of information conservation within the semi-classical gravity framework. This also suggests that spherically symmetric static black holes with a negative heat capacity must have an island and satisfy the QFC theorem.
Our calculations have very broad applicability beyond specific model dependencies. As long as the metric satisfies (4), one can use our calculation to obtain the corresponding island (28), Page curves (29), and the condition for the QFC theorem (46). Therefore, our calculation can be used as a standard procedure to obtain the Page curve (34) and QFC theorem (37).
In the future, we would like to consider the following aspects:
First, our metric (4) only fits an eternal black hole. Although the information paradox for eternal black holes is more straightforward, we are yet to acquire universal results from more realistic models of evaporating black holes. When a dynamical black hole is taken into account, the back-reaction should be considered seriously [99, 100]. The constraint of Eqs. (29) and (46) may be modified. Besides, although the calculations for the QFC in this study are based on a static black hole background, the QFC is a more general theorem. The QFC theorem is rarely studied in terms of a dynamic black hole background [98, 101]. Subsequent studies can extend our results ((27a) and (29)) to the evaporating version.
In addition, we only focused on the contribution of ''s-wave,'' and the other modes with angular momentum were omitted. Nevertheless, we still need to be cautious when using this reduction. In particular, when the observer is close enough to the black hole, this approximation is not valid. Some calculations beyond the s-wave approximation are discussed in [98]. Meanwhile, in the case of non-spherical symmetry, there is a lack of well-defined conformal transformations, such as the Kruskal coordinate transformation (8). The metric (4) cannot be maximally extended to the two-sided geometry form (8). The calculation method presented in this paper is difficult to perform under a non-spherical symmetric configuration. Another interesting and beneficial aspect is to contemplate the scenario where the cut-off surface is close to the black hole (
$ b- r_h \ll 0 $ ). Although the outcomes of the island in this case have been examined in [30, 48], it is worthwhile to investigate whether the QFC theorem holds in this situation.Finally, although we can infer that black holes with negative heat capacities must have islands and follow the QFC, we should apply this conclusion with caution. For a more general case, such as axially symmetric black holes or black holes with topological phase transitions, we still need to treat the constraint expressed in Eqs. (29) and (46) strictly. There may be better physical explanations in the future, and our superficial discussion here may provide some possible references. We end our discussion here.
-
We would like to thank Shuyi Lin, Yuqi-Lei, Ruidong Zhu, and Xiaokai He for their discussions related to the island rule.
-
In this appendix, we briefly give the expression of entanglement entropy in a curved black hole background and discuss what we should consider when using them.
Initially, different from the 2D simple case, the expression of entanglement entropy in the 4D scenario is complicated and has an area-like divergence. Namely, the entropy for matter fields has following expression:
$ \begin{aligned} S_{\text{bulk}} (R \cup I) = \frac{\text{Area}(\partial I)}{\epsilon^2} +S_{\text{bulk}}^{\text{finite}} (R \cup I), \end{aligned} $

(A1) where
$ \epsilon $ is the cutoff, which dominates the area-like divergent term. Then, we can absorb this term by renormalizing the Newton constant:$ \begin{aligned} \frac{1}{4G_N^{(r)}} \equiv \frac{1}{4G_N}+\frac{1}{\epsilon^2}. \end{aligned} $

(A2) Consequently, we can replace the corresponding parts of the island formula (1) with
$ G_N^{(r)} $ and$ S_{\text{bulk}}^{\text{finite}} (R \cup I) $ to yield a finite contribution of the entanglement entropy. Thus, the entanglement entropy in 4D spacetime is$ \begin{aligned} S_{\text{Rad}}=\text{Min} \bigg \{ \text{Ext} \bigg[ \frac{\text{Area}(\partial I)}{4G_N^{(r)}} + S_{\text{bulk}}^{\text{(finite)}} (R \cup I) \bigg] \bigg \}. \end{aligned} $

(A3) Secondly, due to the s-wave approximation, the renormalized von Neumann entropy in vacuum CFT
$ _2 $ in flat spacetime${\rm d}s^2=-{\rm d}x^+{\rm d}x^-$ (with the light cone coordinate$ x^{\pm}=t \pm r $ ) is [92, 93]$ \begin{aligned} S_{\text{bulk}}(A \cup B)=\frac{c}{3} \log (d_{AB}), \end{aligned} $

(A4) with
$ \begin{aligned} d_{AB}\equiv \sqrt{[x^+(A)-x^+(B)][x^-(B)-x^-(A)]}, \end{aligned} $

(A5) in the geodesic distance between points A and B in flat metric. To apply Eq. (A3) to curved spacetime, we must perform the Wely transformation into curved 2D metric
${\rm d}s^2_{2D}=-\Omega^2(x^+,x^-) {\rm d}x^+{\rm d}x^-$ [9]. After the Weyl transformation, we finally obtain the entanglement entropy in general 2D spacetime as [19]$ \begin{aligned} S_{\text{bulk}} (A \cup B)=\frac{c}{6} \log \bigg[ {\rm d}^2(A,B) \Omega(A) \Omega(B) \bigg] \Bigg|_{t_{\pm}=0}. \end{aligned} $

(A6) For the higher-dimensional case, we can still calculate the entanglement entropy by this formula in a manner similar to that of Eq. (8). For the 3D case, we just replace the area term with the length of the system, and for the 2D case, we express the area term in terms of the dilaton.
-
In this appendix, we present the details of the result for 2D black holes in Table 1. The bulk action for 2D gravity can be written in the following form [18, 19, 22]:
$ \begin{aligned} I_{\text{bulk}}=\frac{1}{16\pi G_N} \int {\rm d}^2x \sqrt{-g} \bigg[\Phi \big( R+K(\Phi) (\nabla \Phi)^2 -2V(\Phi) \big) \bigg], \end{aligned} $

(B1) where
$ K(\Phi) $ and$ V(\Phi) $ are$ \begin{aligned} K=0, \qquad \ \ \ V=-\lambda^2, \ \ \qquad \ \text{for JT gravity} \end{aligned} $

(B2a) $ \begin{aligned} K=\frac{1}{\Phi^2}, \qquad V=-2\lambda^2, \ \ \qquad \text{for CGHS model} \end{aligned} $

(B2b) $ \begin{aligned} K=0, \qquad \ \ \ V=-2\lambda^2 {\rm e}^{B \Phi}, \ \ \ \ \text{for Liouville model} \end{aligned} $

(B2c) where λ determines the length of the cosmological constant, and
$ B>0 $ is a constant. We can obtain the vacuum black hole solutions by solving the equations of motion from the action (B1). In the Schwarzschild gauge, the vacuum black hole metric is$ \begin{aligned} {\rm{d}}s^2=-f(r){\rm{d}}t^2+f(r)^{-1}{\rm{d}}r^2, \end{aligned} $

(B3) where
$ \begin{aligned} f(r)=\frac{r^2-r_h^2}{\ell^2}, \qquad \qquad \ \ \ \ \text{for JT gravity} \end{aligned} $

(B4a) $ \begin{aligned} &f(r)=1-{\rm e}^{-2\lambda (r-r_h)}, \qquad \ \text{for CGHS model} \end{aligned} $

(B4b) $ \begin{aligned} f(r)=1-{\rm e}^{-2\sqrt{|C|}\cdot r }. \ \ \qquad \text{for Liouville model} \end{aligned} $

(B4c) Here,
$ \ell $ sets the AdS length, and$ C<0 $ is a constant. For the case of the JT and CGHS models, we can easily calculate and find that their blacken factors$ f(r) $ (B4a) (B4b) satisfy the constraint expressed in Eq. (29) and then obtain the correct results in Table 1. However, in the case of the Liouville black hole, there is no island due to its special properties. Now, we study this situation in detail.A key property of a Liouville black hole is that its area term
$ A(r) $ is negative. For the Liouville solution (B4c), it can be proven that the time t has a periodicity along the imaginary axis. We introduce a new coordinate as$ r=\dfrac{\sqrt{|C|}}{2} R^2 $ . Then, the metric (B4c) in Euclidean time$t={\rm i}\tau$ near the event horizon$ r=0 $ has the following form:$ \begin{aligned} {\rm d}s^2=R^2 {\rm d}(\sqrt{|C|} \tau)^2 +{\rm d}R^2. \end{aligned} $

(B5) Therefore, the Euclidean time has a periodicity of
$ \dfrac{2\pi}{\sqrt{|C|}} $ . Then, we obtain the Hawking temperature$ T_H=\dfrac{\sqrt{|C|}}{2\pi} $ . The expression of the dilaton ϕ in$ (t,r) $ coordinates is given by$ \begin{aligned} \phi= - \frac{2}{B} \sqrt{|C|} r - \frac{1}{B} \log \frac{\lambda^2 B}{C}. \end{aligned} $

(B6) The mass of a black hole is
$ \begin{aligned} M= \frac{2\sqrt{|C|}}{B \pi}. \end{aligned} $

(B7) It is obvious that B must be positive. Therefore, the full restrictions for the parameters are
$ \begin{aligned} C<0, \qquad B>0, \qquad \lambda^2<0. \end{aligned} $

(B8) Combined with the Hawking temperature, we find that
$ T=\frac{B}{4} M $ . Finally, based on the first law of thermodynamics, we obtain the Bekenstein-Hawking entropy as$ \begin{aligned} S_{\text{BH}}=\int \frac{{\rm d}M}{T} = \frac{4}{B} \log M - \frac{2}{B} \log \bigg( \frac{-4 \lambda^2}{B \pi^2} \bigg). \end{aligned} $

(B9) Therefore, the black hole entropy is related to the dilaton at the event horizon:
$ \begin{aligned} S_{\text{BH}}=2\phi_H =2 \phi(r=0). \end{aligned} $

(B10) The area of a Liouville black hole and its derivative are given by
$ \begin{aligned} \begin{split} &A(r)=-\frac{16}{B} \sqrt{|C|} r - \frac{8}{B} \log \frac{\lambda^2 B}{C}, \\ &A^{\prime}(r)=- \frac{16}{B} \sqrt{|C|}. \end{split} \end{aligned} $

(B11) Because of the negative value of
$ A^{\prime}(r_h) $ , the blacken factor (B4c) does not satisfy the constraint expressed in Eq. (29), so the Liouville black hole does not have an island. Then, we prove the validity of our results in Table 1. For more information about Liouville black holes, see Ref. [22]. -
In this appendix, we prove that islands cannot exist inside the event horizon. In Sec. II, we obtain the location of the island by extremizing generalized entropy ((18) and (26)). In addition to the solution where the island is outside the event horizon, there is a solution where the island is inside the event horizon ((19b) and (27b)). The crux of the matter is that our results are based on the Penrose diagram in Fig. 1, where the island is already assumed to be outside the event horizon, so this solution should be discarded. Now, we present the corresponding explicit calculation. In this case, the correct Penrose diagram is shown in Fig. 2.
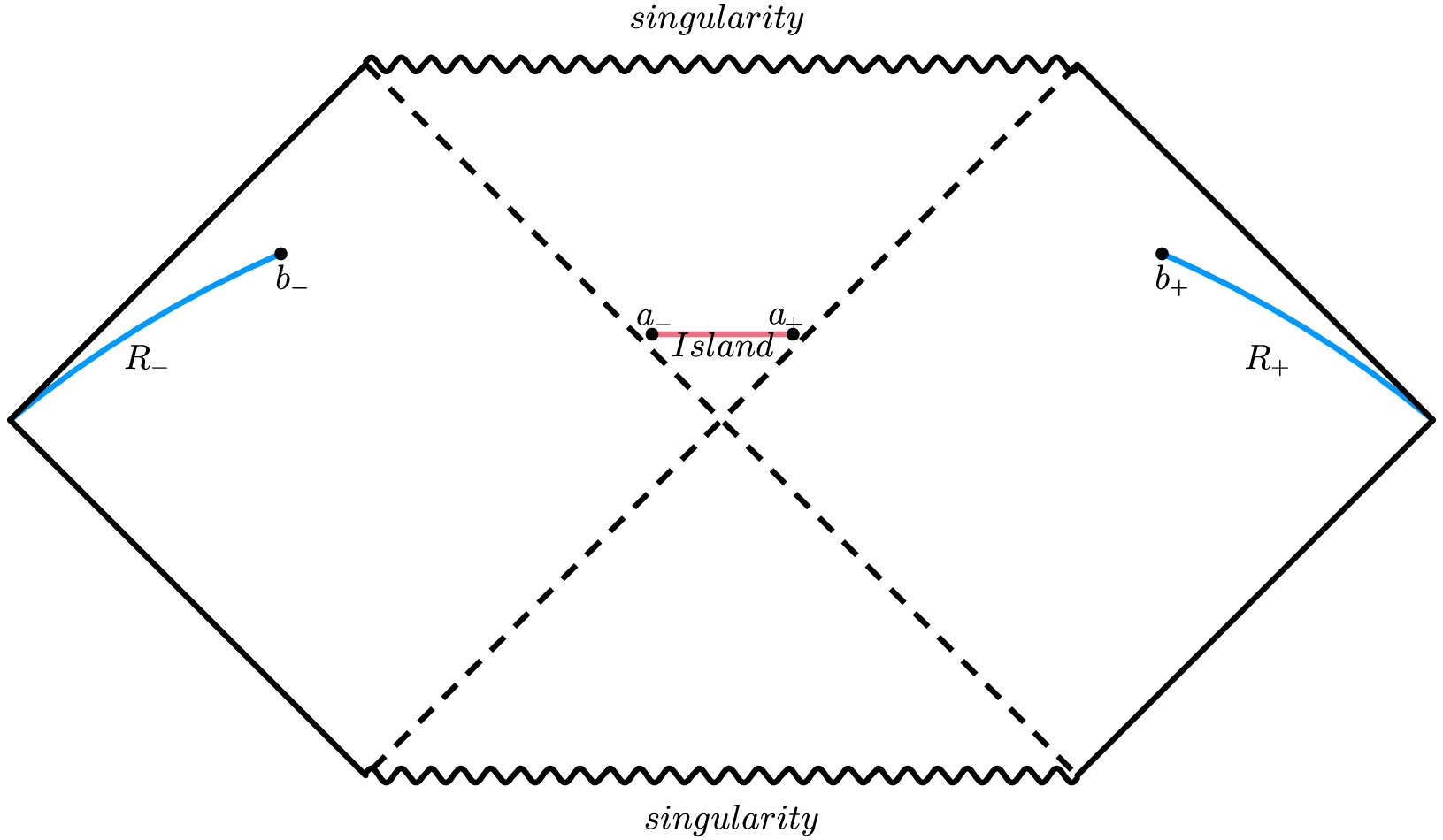
Figure 2. (color online) Penrose diagram in which the island is assumed to be inside the event horizon.
In this construction, the island is located on the top wedge.Thus, the corresponding Kruskal coordinate is different from that in (5). We redefine the Kruskal coordinate as follows:
$ \begin{aligned}[b]& \text{Top Wedge}: \ U\equiv + {\rm e}^{\kappa u}=+ {\rm e}^{\kappa (t-r_{\star}(r))}, \\& V\equiv + {\rm e}^{\kappa v} =+ {\rm e}^{\kappa (t+r_{\star}(r))}. \end{aligned} $

(C1) The generalized entropy at late times is given by substituting the coordinate of the island
$ a_{\pm}=(\pm t_a,a) $ , which is$ \begin{aligned}[b] S_{\text{gen}} \simeq\;& \frac{A(a)}{2G_N} + \frac{c}{3} \log \Big[ {\rm d}(a_+,b_+) {\rm d}(a_-,b_-) \Big] = \frac{A(a)}{2G_N} + \frac{c}{6} \log \Bigg[ \frac{f(a) f(b)}{\kappa^4} \bigg( 1+{\rm e}^{\kappa (r_{\star}(a)+r_{\star}(b)-t_a-t_b)}\bigg) \bigg(-1+{\rm e}^{\kappa(r_{\star}(a)+r_{\star}(b)+t_a+t_b)} \bigg) \times \\ &-{\rm e}^{-2\kappa (2r_{\star}(a)+r_{\star}(b))} \bigg({\rm e}^{\kappa(r_{\star}(a)-t_a)} + {\rm e}^{\kappa(r_{\star}(b)-t_b)} \bigg) \bigg( {\rm e}^{\kappa(r_{\star}(a)+t_a)} + {\rm e}^{\kappa(r_{\star}(b)-t_b)} \bigg) \Bigg]. \end{aligned} $

(C2) Extrimizing this equation with respect to a gives
$ \begin{aligned}[b]&\frac{\partial S_{\text{gen}}}{\partial a}=\frac{1}{6} \Bigg( \frac{3 A^{\prime}(a)}{G_N} + \\ &\frac{c \Big(\kappa \text{csch} [\kappa (r_{\star}(a)+r_{\star}(b))] \text{sech} [\frac{1}{2} \kappa (r_{\star}(a)+r_{\star}(b))] \Big( \text{cosh} [\frac{1}{2} \kappa (3r_{\star}(a)+r_{\star}(b))]+3\big( \text{sinh} [\frac{1}{2} \kappa (3r_{\star}(a)+r_{\star}(b))]\big) +f^{\prime}(a) \Big )}{f(a)}\Bigg) =0. \end{aligned} $

(C3) This equation has no solution, i.e., there is no island for this construction. Therefore, we can prove that islands cannot exist inside the event horizon, and the solutions (19b) and (27b) are not physical and should be rejected.
Geometric constraints via Page curves: insights from island rule and quantum focusing conjecture
- Received Date: 2024-12-20
- Available Online: 2025-04-15
Abstract: To explore the inverse problem tied to the Page curve phenomenon and island paradigm, we investigate the geometric conditions underpinning black hole evaporation, where information is preserved and islands manifest, giving rise to the characteristic Page curve. Focusing on a broad class of static spherical symmetry black hole metrics in asymptotically Minkowski or (anti-)de Sitter spacetimes, we derive a pivotal constraint, the second derivative of the blacken factor






 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF


















 DownLoad:
DownLoad: